↑

Properties

Label 2-1078-7.4-c1-0-30
Degree 22
Conductor 10781078
Sign −0.900−0.435i-0.900 - 0.435i
Analytic cond. 8.607878.60787
Root an. cond. 2.933912.93391
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−0.5 − 0.866i)2-s + (1.41 − 2.44i)3-s + (−0.499 + 0.866i)4-s − 2.82·6-s + 0.999·8-s + (−2.49 − 4.33i)9-s + (0.5 − 0.866i)11-s + (1.41 + 2.44i)12-s − 4.24·13-s + (−0.5 − 0.866i)16-s + (−1.41 + 2.44i)17-s + (−2.5 + 4.33i)18-s + (−2.12 − 3.67i)19-s − 0.999·22-s + (−3 − 5.19i)23-s + (1.41 − 2.44i)24-s + ⋯
L(s)  = 1  + (−0.353 − 0.612i)2-s + (0.816 − 1.41i)3-s + (−0.249 + 0.433i)4-s − 1.15·6-s + 0.353·8-s + (−0.833 − 1.44i)9-s + (0.150 − 0.261i)11-s + (0.408 + 0.707i)12-s − 1.17·13-s + (−0.125 − 0.216i)16-s + (−0.342 + 0.594i)17-s + (−0.589 + 1.02i)18-s + (−0.486 − 0.842i)19-s − 0.213·22-s + (−0.625 − 1.08i)23-s + (0.288 − 0.499i)24-s + ⋯

Functional equation

Λ(s)=(1078s/2 ΓC(s) L(s)=((−0.900−0.435i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 1078 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.900 - 0.435i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(1078s/2 ΓC(s+1/2) L(s)=((−0.900−0.435i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 1078 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (-0.900 - 0.435i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 10781078    =    2⋅72⋅112 \cdot 7^{2} \cdot 11
Sign: −0.900−0.435i-0.900 - 0.435i
Analytic conductor: 8.607878.60787
Root analytic conductor: 2.933912.93391
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ1078(67,⋅)\chi_{1078} (67, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 1078, ( :1/2), −0.900−0.435i)(2,\ 1078,\ (\ :1/2),\ -0.900 - 0.435i)

Particular Values

L(1)L(1) ≈\approx 1.1201511561.120151156
L(12)L(\frac12) ≈\approx 1.1201511561.120151156
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1+(0.5+0.866i)T 1 + (0.5 + 0.866i)T
7 1 1
11 1+(−0.5+0.866i)T 1 + (-0.5 + 0.866i)T
good3 1+(−1.41+2.44i)T+(−1.5−2.59i)T2 1 + (-1.41 + 2.44i)T + (-1.5 - 2.59i)T^{2}
5 1+(−2.5+4.33i)T2 1 + (-2.5 + 4.33i)T^{2}
13 1+4.24T+13T2 1 + 4.24T + 13T^{2}
17 1+(1.41−2.44i)T+(−8.5−14.7i)T2 1 + (1.41 - 2.44i)T + (-8.5 - 14.7i)T^{2}
19 1+(2.12+3.67i)T+(−9.5+16.4i)T2 1 + (2.12 + 3.67i)T + (-9.5 + 16.4i)T^{2}
23 1+(3+5.19i)T+(−11.5+19.9i)T2 1 + (3 + 5.19i)T + (-11.5 + 19.9i)T^{2}
29 1+4T+29T2 1 + 4T + 29T^{2}
31 1+(3.53−6.12i)T+(−15.5−26.8i)T2 1 + (3.53 - 6.12i)T + (-15.5 - 26.8i)T^{2}
37 1+(1+1.73i)T+(−18.5+32.0i)T2 1 + (1 + 1.73i)T + (-18.5 + 32.0i)T^{2}
41 1+2.82T+41T2 1 + 2.82T + 41T^{2}
43 1−10T+43T2 1 - 10T + 43T^{2}
47 1+(6.36+11.0i)T+(−23.5+40.7i)T2 1 + (6.36 + 11.0i)T + (-23.5 + 40.7i)T^{2}
53 1+(1−1.73i)T+(−26.5−45.8i)T2 1 + (1 - 1.73i)T + (-26.5 - 45.8i)T^{2}
59 1+(−5.65+9.79i)T+(−29.5−51.0i)T2 1 + (-5.65 + 9.79i)T + (-29.5 - 51.0i)T^{2}
61 1+(4.94+8.57i)T+(−30.5+52.8i)T2 1 + (4.94 + 8.57i)T + (-30.5 + 52.8i)T^{2}
67 1+(4−6.92i)T+(−33.5−58.0i)T2 1 + (4 - 6.92i)T + (-33.5 - 58.0i)T^{2}
71 1−16T+71T2 1 - 16T + 71T^{2}
73 1+(4.24−7.34i)T+(−36.5−63.2i)T2 1 + (4.24 - 7.34i)T + (-36.5 - 63.2i)T^{2}
79 1+(−4−6.92i)T+(−39.5+68.4i)T2 1 + (-4 - 6.92i)T + (-39.5 + 68.4i)T^{2}
83 1−12.7T+83T2 1 - 12.7T + 83T^{2}
89 1+(−3.53−6.12i)T+(−44.5+77.0i)T2 1 + (-3.53 - 6.12i)T + (-44.5 + 77.0i)T^{2}
97 1−7.07T+97T2 1 - 7.07T + 97T^{2}
show more
show less
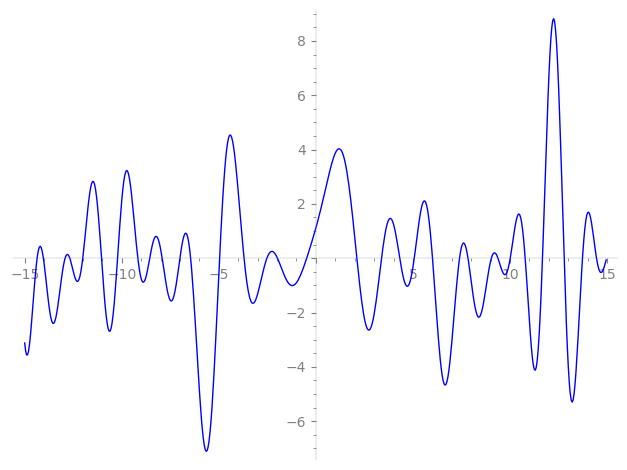
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−9.171316356343740830047405349700, −8.558859488221935065722470645786, −7.919059253841763668079832475053, −7.00373500656771295472481831342, −6.45319301036509355692565872599, −4.96062900121633490672830171472, −3.70046234650284048089165966192, −2.52574081106356780936833481250, −1.97992678074676154169877537150, −0.47469420424988958869217618089, 2.11040485851714521394313151517, 3.38027428860066129907985008354, 4.32104686664175196371116493131, 5.06540838151111309884663631527, 6.00618605548551538378192941071, 7.39702067397034029106159043892, 7.83414443072269252509386446566, 9.037594107898982837976759381387, 9.376942397849247572567098136008, 10.00942849901404432893968260493

Graph of the ZZ-function along the critical line