↑

Properties

Label 2-161-161.68-c1-0-6
Degree 22
Conductor 161161
Sign 0.507−0.861i0.507 - 0.861i
Analytic cond. 1.285591.28559
Root an. cond. 1.133831.13383
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−0.559 + 0.969i)2-s + (2.13 − 1.23i)3-s + (0.372 + 0.645i)4-s + (−0.871 + 1.50i)5-s + 2.75i·6-s + (−0.785 + 2.52i)7-s − 3.07·8-s + (1.53 − 2.66i)9-s + (−0.975 − 1.68i)10-s + (4.42 − 2.55i)11-s + (1.59 + 0.918i)12-s − 1.11i·13-s + (−2.01 − 2.17i)14-s + 4.29i·15-s + (0.976 − 1.69i)16-s + (−1.62 − 2.81i)17-s + ⋯
L(s)  = 1  + (−0.395 + 0.685i)2-s + (1.23 − 0.711i)3-s + (0.186 + 0.322i)4-s + (−0.389 + 0.674i)5-s + 1.12i·6-s + (−0.297 + 0.954i)7-s − 1.08·8-s + (0.512 − 0.886i)9-s + (−0.308 − 0.534i)10-s + (1.33 − 0.769i)11-s + (0.459 + 0.265i)12-s − 0.309i·13-s + (−0.537 − 0.581i)14-s + 1.10i·15-s + (0.244 − 0.422i)16-s + (−0.394 − 0.682i)17-s + ⋯

Functional equation

Λ(s)=(161s/2 ΓC(s) L(s)=((0.507−0.861i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 161 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.507 - 0.861i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(161s/2 ΓC(s+1/2) L(s)=((0.507−0.861i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 161 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.507 - 0.861i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 161161    =    7⋅237 \cdot 23
Sign: 0.507−0.861i0.507 - 0.861i
Analytic conductor: 1.285591.28559
Root analytic conductor: 1.133831.13383
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ161(68,⋅)\chi_{161} (68, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 161, ( :1/2), 0.507−0.861i)(2,\ 161,\ (\ :1/2),\ 0.507 - 0.861i)

Particular Values

L(1)L(1) ≈\approx 1.13218+0.646812i1.13218 + 0.646812i
L(12)L(\frac12) ≈\approx 1.13218+0.646812i1.13218 + 0.646812i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad7 1+(0.785−2.52i)T 1 + (0.785 - 2.52i)T
23 1+(4.76+0.499i)T 1 + (4.76 + 0.499i)T
good2 1+(0.559−0.969i)T+(−1−1.73i)T2 1 + (0.559 - 0.969i)T + (-1 - 1.73i)T^{2}
3 1+(−2.13+1.23i)T+(1.5−2.59i)T2 1 + (-2.13 + 1.23i)T + (1.5 - 2.59i)T^{2}
5 1+(0.871−1.50i)T+(−2.5−4.33i)T2 1 + (0.871 - 1.50i)T + (-2.5 - 4.33i)T^{2}
11 1+(−4.42+2.55i)T+(5.5−9.52i)T2 1 + (-4.42 + 2.55i)T + (5.5 - 9.52i)T^{2}
13 1+1.11iT−13T2 1 + 1.11iT - 13T^{2}
17 1+(1.62+2.81i)T+(−8.5+14.7i)T2 1 + (1.62 + 2.81i)T + (-8.5 + 14.7i)T^{2}
19 1+(−2.74+4.74i)T+(−9.5−16.4i)T2 1 + (-2.74 + 4.74i)T + (-9.5 - 16.4i)T^{2}
29 1+6.58T+29T2 1 + 6.58T + 29T^{2}
31 1+(−4.15+2.40i)T+(15.5−26.8i)T2 1 + (-4.15 + 2.40i)T + (15.5 - 26.8i)T^{2}
37 1+(−1.07−0.619i)T+(18.5+32.0i)T2 1 + (-1.07 - 0.619i)T + (18.5 + 32.0i)T^{2}
41 1+10.3iT−41T2 1 + 10.3iT - 41T^{2}
43 1−7.76iT−43T2 1 - 7.76iT - 43T^{2}
47 1+(−1.68−0.972i)T+(23.5+40.7i)T2 1 + (-1.68 - 0.972i)T + (23.5 + 40.7i)T^{2}
53 1+(−0.912+0.526i)T+(26.5−45.8i)T2 1 + (-0.912 + 0.526i)T + (26.5 - 45.8i)T^{2}
59 1+(6.93−4.00i)T+(29.5−51.0i)T2 1 + (6.93 - 4.00i)T + (29.5 - 51.0i)T^{2}
61 1+(3.59−6.22i)T+(−30.5−52.8i)T2 1 + (3.59 - 6.22i)T + (-30.5 - 52.8i)T^{2}
67 1+(7.28−4.20i)T+(33.5−58.0i)T2 1 + (7.28 - 4.20i)T + (33.5 - 58.0i)T^{2}
71 1−1.68T+71T2 1 - 1.68T + 71T^{2}
73 1+(−5.01+2.89i)T+(36.5−63.2i)T2 1 + (-5.01 + 2.89i)T + (36.5 - 63.2i)T^{2}
79 1+(9.52+5.50i)T+(39.5+68.4i)T2 1 + (9.52 + 5.50i)T + (39.5 + 68.4i)T^{2}
83 1−11.4T+83T2 1 - 11.4T + 83T^{2}
89 1+(5.34−9.25i)T+(−44.5−77.0i)T2 1 + (5.34 - 9.25i)T + (-44.5 - 77.0i)T^{2}
97 1−12.4T+97T2 1 - 12.4T + 97T^{2}
show more
show less
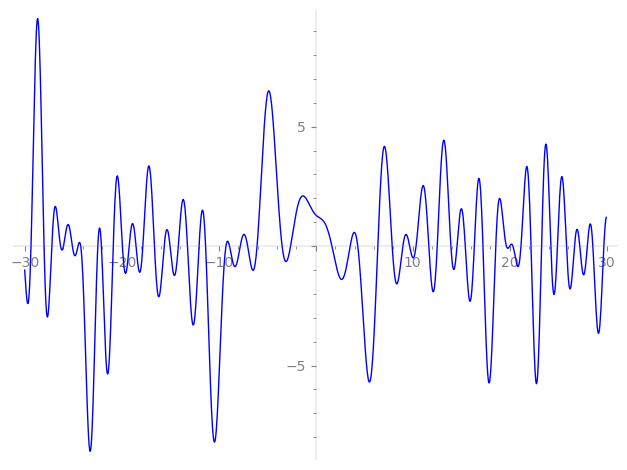
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−13.21992417828459527624487356088, −12.02767006298554321315826615047, −11.35200313474712428030918812690, −9.253028284810469308877593937524, −8.883805978456413034008651916188, −7.77883520472449609029984148346, −7.01827096021616567630983081866, −6.04739187518912033738077173784, −3.46459840327484294226962780875, −2.59304067524168429186896821376, 1.66966884574055433533808159256, 3.54834700039656961383275738515, 4.33383094682761684975757504276, 6.39892800750024859367845069547, 7.88107746808528651599700347516, 8.982507083428816010347735882342, 9.680779034713982077274554937441, 10.34228528825902482635318138498, 11.66005720790818311120169645053, 12.52521317561719268387565687134

Graph of the ZZ-function along the critical line