↑

Properties

Label 2-13e2-13.12-c7-0-7
Degree 22
Conductor 169169
Sign 0.246+0.969i0.246 + 0.969i
Analytic cond. 52.793052.7930
Root an. cond. 7.265887.26588
Motivic weight 77
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + 19.6i·2-s − 72.4·3-s − 257.·4-s − 413. i·5-s − 1.42e3i·6-s + 1.00e3i·7-s − 2.53e3i·8-s + 3.06e3·9-s + 8.11e3·10-s + 3.48e3i·11-s + 1.86e4·12-s − 1.97e4·14-s + 2.99e4i·15-s + 1.68e4·16-s − 6.15e3·17-s + 6.01e4i·18-s + ⋯
L(s)  = 1  + 1.73i·2-s − 1.54·3-s − 2.00·4-s − 1.48i·5-s − 2.68i·6-s + 1.10i·7-s − 1.74i·8-s + 1.40·9-s + 2.56·10-s + 0.788i·11-s + 3.11·12-s − 1.92·14-s + 2.29i·15-s + 1.02·16-s − 0.303·17-s + 2.43i·18-s + ⋯

Functional equation

Λ(s)=(169s/2 ΓC(s) L(s)=((0.246+0.969i) Λ‾(8−s)\begin{aligned}\Lambda(s)=\mathstrut & 169 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.246 + 0.969i)\, \overline{\Lambda}(8-s) \end{aligned}
Λ(s)=(169s/2 ΓC(s+7/2) L(s)=((0.246+0.969i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 169 ^{s/2} \, \Gamma_{\C}(s+7/2) \, L(s)\cr =\mathstrut & (0.246 + 0.969i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 169169    =    13213^{2}
Sign: 0.246+0.969i0.246 + 0.969i
Analytic conductor: 52.793052.7930
Root analytic conductor: 7.265887.26588
Motivic weight: 77
Rational: no
Arithmetic: yes
Character: χ169(168,⋅)\chi_{169} (168, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 169, ( :7/2), 0.246+0.969i)(2,\ 169,\ (\ :7/2),\ 0.246 + 0.969i)

Particular Values

L(4)L(4) ≈\approx 0.34739841460.3473984146
L(12)L(\frac12) ≈\approx 0.34739841460.3473984146
L(92)L(\frac{9}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad13 1 1
good2 1−19.6iT−128T2 1 - 19.6iT - 128T^{2}
3 1+72.4T+2.18e3T2 1 + 72.4T + 2.18e3T^{2}
5 1+413.iT−7.81e4T2 1 + 413. iT - 7.81e4T^{2}
7 1−1.00e3iT−8.23e5T2 1 - 1.00e3iT - 8.23e5T^{2}
11 1−3.48e3iT−1.94e7T2 1 - 3.48e3iT - 1.94e7T^{2}
17 1+6.15e3T+4.10e8T2 1 + 6.15e3T + 4.10e8T^{2}
19 1−4.14e4iT−8.93e8T2 1 - 4.14e4iT - 8.93e8T^{2}
23 1−6.31e4T+3.40e9T2 1 - 6.31e4T + 3.40e9T^{2}
29 1−7.51e4T+1.72e10T2 1 - 7.51e4T + 1.72e10T^{2}
31 1−1.33e5iT−2.75e10T2 1 - 1.33e5iT - 2.75e10T^{2}
37 1−1.71e5iT−9.49e10T2 1 - 1.71e5iT - 9.49e10T^{2}
41 1−7.47e5iT−1.94e11T2 1 - 7.47e5iT - 1.94e11T^{2}
43 1−1.87e5T+2.71e11T2 1 - 1.87e5T + 2.71e11T^{2}
47 1+2.26e5iT−5.06e11T2 1 + 2.26e5iT - 5.06e11T^{2}
53 1+1.34e6T+1.17e12T2 1 + 1.34e6T + 1.17e12T^{2}
59 1+2.50e6iT−2.48e12T2 1 + 2.50e6iT - 2.48e12T^{2}
61 1+5.09e5T+3.14e12T2 1 + 5.09e5T + 3.14e12T^{2}
67 1−4.07e6iT−6.06e12T2 1 - 4.07e6iT - 6.06e12T^{2}
71 1−2.56e5iT−9.09e12T2 1 - 2.56e5iT - 9.09e12T^{2}
73 1−5.38e6iT−1.10e13T2 1 - 5.38e6iT - 1.10e13T^{2}
79 1−5.18e6T+1.92e13T2 1 - 5.18e6T + 1.92e13T^{2}
83 1+5.32e6iT−2.71e13T2 1 + 5.32e6iT - 2.71e13T^{2}
89 1+8.69e6iT−4.42e13T2 1 + 8.69e6iT - 4.42e13T^{2}
97 1+3.76e6iT−8.07e13T2 1 + 3.76e6iT - 8.07e13T^{2}
show more
show less
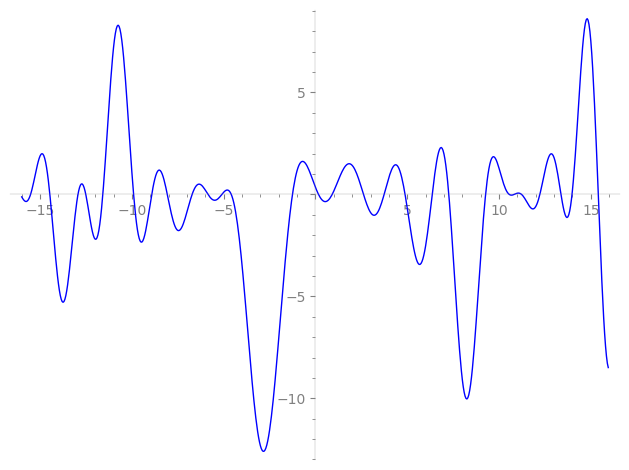
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−12.51093962649296178477959669427, −11.58321521563468076732969835301, −9.906040506655325032015727267893, −8.917579321960762068162349682698, −8.070471818271077788097263058812, −6.71842351885329967917528104719, −5.85375815146812132258733447973, −5.09738863687875403514159850001, −4.58678126790499134535816905482, −1.25200992463072740043971154339, 0.16546893688342005974362134973, 0.911361078239831954019097088255, 2.61787490388716319399267259029, 3.73988974833626095740925269069, 4.88079537785908184590519504944, 6.36028528516263733370021909967, 7.26314566552125032027356397042, 9.268023804459677606836722670634, 10.55488992558382464989946519330, 10.82537748264663546341752192524

Graph of the ZZ-function along the critical line