↑

Properties

Label 2-42e2-49.12-c0-0-0
Degree $2$
Conductor $1764$
Sign $0.232 - 0.972i$
Analytic cond. $0.880350$
Root an. cond. $0.938270$
Motivic weight $0$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−0.826 + 0.563i)7-s + (−0.590 + 1.22i)13-s + (0.975 + 0.563i)19-s + (0.826 + 0.563i)25-s + (−1.61 + 0.930i)31-s + (0.722 + 1.84i)37-s + (0.0332 − 0.145i)43-s + (0.365 − 0.930i)49-s + (1.45 − 0.571i)61-s + (−0.955 − 1.65i)67-s + (−0.167 + 0.246i)73-s + (−0.365 + 0.632i)79-s + (−0.202 − 1.34i)91-s + 0.867i·97-s + (−0.587 + 1.90i)103-s + ⋯
L(s)  = 1  + (−0.826 + 0.563i)7-s + (−0.590 + 1.22i)13-s + (0.975 + 0.563i)19-s + (0.826 + 0.563i)25-s + (−1.61 + 0.930i)31-s + (0.722 + 1.84i)37-s + (0.0332 − 0.145i)43-s + (0.365 − 0.930i)49-s + (1.45 − 0.571i)61-s + (−0.955 − 1.65i)67-s + (−0.167 + 0.246i)73-s + (−0.365 + 0.632i)79-s + (−0.202 − 1.34i)91-s + 0.867i·97-s + (−0.587 + 1.90i)103-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 1764 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.232 - 0.972i)\, \overline{\Lambda}(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 1764 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.232 - 0.972i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(1764\)    =    \(2^{2} \cdot 3^{2} \cdot 7^{2}\)
Sign: $0.232 - 0.972i$
Analytic conductor: \(0.880350\)
Root analytic conductor: \(0.938270\)
Motivic weight: \(0\)
Rational: no
Arithmetic: yes
Character: $\chi_{1764} (649, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 1764,\ (\ :0),\ 0.232 - 0.972i)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(0.9032618248\)
\(L(\frac12)\) \(\approx\) \(0.9032618248\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad2 \( 1 \)
3 \( 1 \)
7 \( 1 + (0.826 - 0.563i)T \)
good5 \( 1 + (-0.826 - 0.563i)T^{2} \)
11 \( 1 + (-0.988 + 0.149i)T^{2} \)
13 \( 1 + (0.590 - 1.22i)T + (-0.623 - 0.781i)T^{2} \)
17 \( 1 + (-0.955 - 0.294i)T^{2} \)
19 \( 1 + (-0.975 - 0.563i)T + (0.5 + 0.866i)T^{2} \)
23 \( 1 + (0.955 - 0.294i)T^{2} \)
29 \( 1 + (-0.222 - 0.974i)T^{2} \)
31 \( 1 + (1.61 - 0.930i)T + (0.5 - 0.866i)T^{2} \)
37 \( 1 + (-0.722 - 1.84i)T + (-0.733 + 0.680i)T^{2} \)
41 \( 1 + (0.900 - 0.433i)T^{2} \)
43 \( 1 + (-0.0332 + 0.145i)T + (-0.900 - 0.433i)T^{2} \)
47 \( 1 + (-0.365 + 0.930i)T^{2} \)
53 \( 1 + (-0.733 - 0.680i)T^{2} \)
59 \( 1 + (-0.826 + 0.563i)T^{2} \)
61 \( 1 + (-1.45 + 0.571i)T + (0.733 - 0.680i)T^{2} \)
67 \( 1 + (0.955 + 1.65i)T + (-0.5 + 0.866i)T^{2} \)
71 \( 1 + (-0.222 + 0.974i)T^{2} \)
73 \( 1 + (0.167 - 0.246i)T + (-0.365 - 0.930i)T^{2} \)
79 \( 1 + (0.365 - 0.632i)T + (-0.5 - 0.866i)T^{2} \)
83 \( 1 + (-0.623 + 0.781i)T^{2} \)
89 \( 1 + (0.988 + 0.149i)T^{2} \)
97 \( 1 - 0.867iT - T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

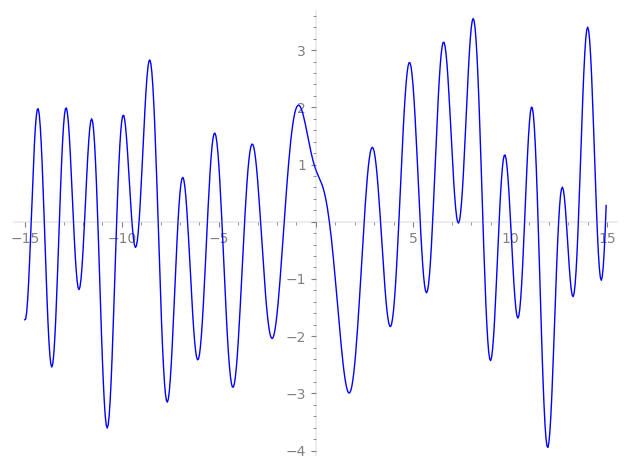
Imaginary part of the first few zeros on the critical line

−9.476605675225854430690541183072, −9.109741304963965972019918059091, −8.126263108261862559686834295362, −7.10235969981292409880484890757, −6.60895600833690614797475677467, −5.58868580702850391724913819448, −4.84135292788876722116137899542, −3.67289543871433556881637552835, −2.84982457306577629642088138994, −1.63244375822373456471380590988, 0.69347759071025182469069164394, 2.48395941916319935296508840565, 3.32921518054907508555654711833, 4.27310679034682571741678712989, 5.37270272152770468975319676049, 6.01316272848023151044913984902, 7.29575311101666243852545916410, 7.38182767828418661917798789364, 8.602610128831082964533823011725, 9.437806640231741288607240951869

Graph of the $Z$-function along the critical line