↑

Properties

Label 2-2160-15.14-c2-0-6
Degree 22
Conductor 21602160
Sign −0.707−0.707i-0.707 - 0.707i
Analytic cond. 58.855758.8557
Root an. cond. 7.671747.67174
Motivic weight 22
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (3.53 − 3.53i)5-s + 5i·7-s − 1.41i·11-s − 9i·13-s − 11.3·17-s − 21·19-s + 1.41·23-s − 25.0i·25-s + 38.1i·29-s − 40·31-s + (17.6 + 17.6i)35-s + 25i·37-s + 52.3i·41-s + 64i·43-s + 22.6·47-s + ⋯
L(s)  = 1  + (0.707 − 0.707i)5-s + 0.714i·7-s − 0.128i·11-s − 0.692i·13-s − 0.665·17-s − 1.10·19-s + 0.0614·23-s − 1.00i·25-s + 1.31i·29-s − 1.29·31-s + (0.505 + 0.505i)35-s + 0.675i·37-s + 1.27i·41-s + 1.48i·43-s + 0.481·47-s + ⋯

Functional equation

Λ(s)=(2160s/2 ΓC(s) L(s)=((−0.707−0.707i) Λ‾(3−s)\begin{aligned}\Lambda(s)=\mathstrut & 2160 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.707 - 0.707i)\, \overline{\Lambda}(3-s) \end{aligned}
Λ(s)=(2160s/2 ΓC(s+1) L(s)=((−0.707−0.707i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 2160 ^{s/2} \, \Gamma_{\C}(s+1) \, L(s)\cr =\mathstrut & (-0.707 - 0.707i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 21602160    =    24⋅33⋅52^{4} \cdot 3^{3} \cdot 5
Sign: −0.707−0.707i-0.707 - 0.707i
Analytic conductor: 58.855758.8557
Root analytic conductor: 7.671747.67174
Motivic weight: 22
Rational: no
Arithmetic: yes
Character: χ2160(1889,⋅)\chi_{2160} (1889, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 2160, ( :1), −0.707−0.707i)(2,\ 2160,\ (\ :1),\ -0.707 - 0.707i)

Particular Values

L(32)L(\frac{3}{2}) ≈\approx 0.59549770030.5954977003
L(12)L(\frac12) ≈\approx 0.59549770030.5954977003
L(2)L(2) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1 1
3 1 1
5 1+(−3.53+3.53i)T 1 + (-3.53 + 3.53i)T
good7 1−5iT−49T2 1 - 5iT - 49T^{2}
11 1+1.41iT−121T2 1 + 1.41iT - 121T^{2}
13 1+9iT−169T2 1 + 9iT - 169T^{2}
17 1+11.3T+289T2 1 + 11.3T + 289T^{2}
19 1+21T+361T2 1 + 21T + 361T^{2}
23 1−1.41T+529T2 1 - 1.41T + 529T^{2}
29 1−38.1iT−841T2 1 - 38.1iT - 841T^{2}
31 1+40T+961T2 1 + 40T + 961T^{2}
37 1−25iT−1.36e3T2 1 - 25iT - 1.36e3T^{2}
41 1−52.3iT−1.68e3T2 1 - 52.3iT - 1.68e3T^{2}
43 1−64iT−1.84e3T2 1 - 64iT - 1.84e3T^{2}
47 1−22.6T+2.20e3T2 1 - 22.6T + 2.20e3T^{2}
53 1+72.1T+2.80e3T2 1 + 72.1T + 2.80e3T^{2}
59 1+90.5iT−3.48e3T2 1 + 90.5iT - 3.48e3T^{2}
61 1+97T+3.72e3T2 1 + 97T + 3.72e3T^{2}
67 1+131iT−4.48e3T2 1 + 131iT - 4.48e3T^{2}
71 1−89.0iT−5.04e3T2 1 - 89.0iT - 5.04e3T^{2}
73 1−17iT−5.32e3T2 1 - 17iT - 5.32e3T^{2}
79 1−117T+6.24e3T2 1 - 117T + 6.24e3T^{2}
83 1+57.9T+6.88e3T2 1 + 57.9T + 6.88e3T^{2}
89 1−147.iT−7.92e3T2 1 - 147. iT - 7.92e3T^{2}
97 1−41iT−9.40e3T2 1 - 41iT - 9.40e3T^{2}
show more
show less
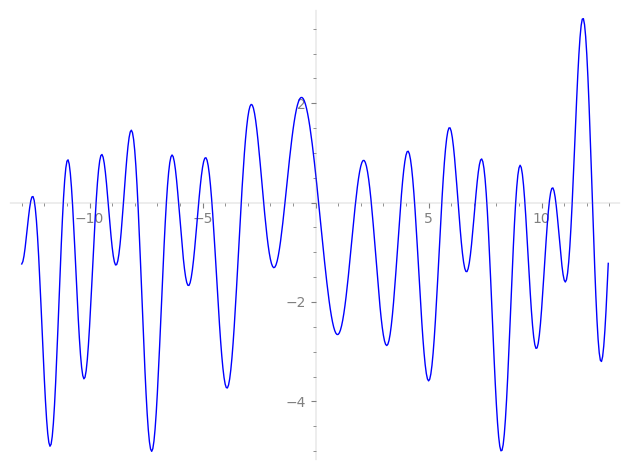
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−9.176410235074926734081731739691, −8.499458238294057053171123929856, −7.86074355653614687308563263222, −6.60076542143041766899026620553, −6.06068095944580790446690847324, −5.17080828204824696250574661241, −4.58682408313203652006964305614, −3.28914110157508641836244195694, −2.29652701738692474314126576570, −1.35324049645282745087552969738, 0.13508166798981376824091877532, 1.76894622033294934605330827258, 2.46469806440888987275187681810, 3.77160875468792456922303715010, 4.38001022824450552800813770398, 5.58456596733105015064515099083, 6.30668597144511230388065753821, 7.06496771434930337393498562400, 7.57683409115659473416068047037, 8.852388189139691522678511801231

Graph of the ZZ-function along the critical line