↑

Properties

Label 2-270-5.2-c2-0-13
Degree 22
Conductor 270270
Sign 0.163+0.986i0.163 + 0.986i
Analytic cond. 7.356967.35696
Root an. cond. 2.712372.71237
Motivic weight 22
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (1 + i)2-s + 2i·4-s + (−4.62 − 1.89i)5-s + (−6.13 − 6.13i)7-s + (−2 + 2i)8-s + (−2.73 − 6.52i)10-s + 8.32·11-s + (14.2 − 14.2i)13-s − 12.2i·14-s − 4·16-s + (−21.9 − 21.9i)17-s − 30.5i·19-s + (3.79 − 9.25i)20-s + (8.32 + 8.32i)22-s + (−17.1 + 17.1i)23-s + ⋯
L(s)  = 1  + (0.5 + 0.5i)2-s + 0.5i·4-s + (−0.925 − 0.379i)5-s + (−0.876 − 0.876i)7-s + (−0.250 + 0.250i)8-s + (−0.273 − 0.652i)10-s + 0.757·11-s + (1.09 − 1.09i)13-s − 0.876i·14-s − 0.250·16-s + (−1.29 − 1.29i)17-s − 1.61i·19-s + (0.189 − 0.462i)20-s + (0.378 + 0.378i)22-s + (−0.744 + 0.744i)23-s + ⋯

Functional equation

Λ(s)=(270s/2 ΓC(s) L(s)=((0.163+0.986i) Λ‾(3−s)\begin{aligned}\Lambda(s)=\mathstrut & 270 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.163 + 0.986i)\, \overline{\Lambda}(3-s) \end{aligned}
Λ(s)=(270s/2 ΓC(s+1) L(s)=((0.163+0.986i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 270 ^{s/2} \, \Gamma_{\C}(s+1) \, L(s)\cr =\mathstrut & (0.163 + 0.986i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 270270    =    2⋅33⋅52 \cdot 3^{3} \cdot 5
Sign: 0.163+0.986i0.163 + 0.986i
Analytic conductor: 7.356967.35696
Root analytic conductor: 2.712372.71237
Motivic weight: 22
Rational: no
Arithmetic: yes
Character: χ270(217,⋅)\chi_{270} (217, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 270, ( :1), 0.163+0.986i)(2,\ 270,\ (\ :1),\ 0.163 + 0.986i)

Particular Values

L(32)L(\frac{3}{2}) ≈\approx 0.860870−0.729568i0.860870 - 0.729568i
L(12)L(\frac12) ≈\approx 0.860870−0.729568i0.860870 - 0.729568i
L(2)L(2) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1+(−1−i)T 1 + (-1 - i)T
3 1 1
5 1+(4.62+1.89i)T 1 + (4.62 + 1.89i)T
good7 1+(6.13+6.13i)T+49iT2 1 + (6.13 + 6.13i)T + 49iT^{2}
11 1−8.32T+121T2 1 - 8.32T + 121T^{2}
13 1+(−14.2+14.2i)T−169iT2 1 + (-14.2 + 14.2i)T - 169iT^{2}
17 1+(21.9+21.9i)T+289iT2 1 + (21.9 + 21.9i)T + 289iT^{2}
19 1+30.5iT−361T2 1 + 30.5iT - 361T^{2}
23 1+(17.1−17.1i)T−529iT2 1 + (17.1 - 17.1i)T - 529iT^{2}
29 1−37.8iT−841T2 1 - 37.8iT - 841T^{2}
31 1+13.8T+961T2 1 + 13.8T + 961T^{2}
37 1+(27.6+27.6i)T+1.36e3iT2 1 + (27.6 + 27.6i)T + 1.36e3iT^{2}
41 1+19.1T+1.68e3T2 1 + 19.1T + 1.68e3T^{2}
43 1+(−13.8+13.8i)T−1.84e3iT2 1 + (-13.8 + 13.8i)T - 1.84e3iT^{2}
47 1+(−3.65−3.65i)T+2.20e3iT2 1 + (-3.65 - 3.65i)T + 2.20e3iT^{2}
53 1+(−13.0+13.0i)T−2.80e3iT2 1 + (-13.0 + 13.0i)T - 2.80e3iT^{2}
59 1+18.2iT−3.48e3T2 1 + 18.2iT - 3.48e3T^{2}
61 1+11.2T+3.72e3T2 1 + 11.2T + 3.72e3T^{2}
67 1+(−79.5−79.5i)T+4.48e3iT2 1 + (-79.5 - 79.5i)T + 4.48e3iT^{2}
71 1−15.3T+5.04e3T2 1 - 15.3T + 5.04e3T^{2}
73 1+(−34.5+34.5i)T−5.32e3iT2 1 + (-34.5 + 34.5i)T - 5.32e3iT^{2}
79 1−80.8iT−6.24e3T2 1 - 80.8iT - 6.24e3T^{2}
83 1+(−12.4+12.4i)T−6.88e3iT2 1 + (-12.4 + 12.4i)T - 6.88e3iT^{2}
89 1+0.375iT−7.92e3T2 1 + 0.375iT - 7.92e3T^{2}
97 1+(37.9+37.9i)T+9.40e3iT2 1 + (37.9 + 37.9i)T + 9.40e3iT^{2}
show more
show less
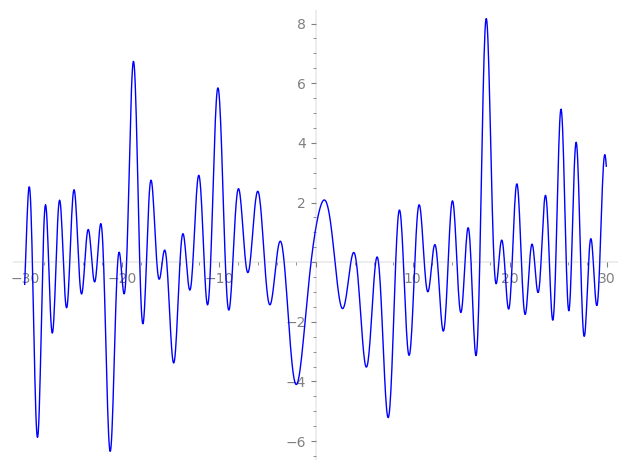
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−11.49399276652225040518292736596, −10.83071477882756787958546343270, −9.318738735043460905227933682691, −8.547229381907031056131467167478, −7.22564927868087526383064206689, −6.76171757438334057167039629699, −5.26546511015823932722964130615, −4.07643847695168054145102059060, −3.25394227171755998090796040680, −0.48328298737525934096403058654, 1.99113156340892331599633803380, 3.62203056379191333528496468407, 4.18644401056445974935941764368, 6.18083600477869917402805286938, 6.46864443441938883095688482267, 8.245293521856778029784645794258, 9.035346082067799787486270679235, 10.21470343905843880546990182553, 11.18965799497690072790544971928, 11.98018112764859970659944272215

Graph of the ZZ-function along the critical line