↑

Properties

Label 2-3150-5.4-c1-0-25
Degree 22
Conductor 31503150
Sign 0.894+0.447i0.894 + 0.447i
Analytic cond. 25.152825.1528
Root an. cond. 5.015265.01526
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + i·2-s − 4-s + i·7-s − i·8-s − 2·11-s + i·13-s − 14-s + 16-s − i·17-s − 4·19-s − 2i·22-s − 7i·23-s − 26-s − i·28-s + 29-s + ⋯
L(s)  = 1  + 0.707i·2-s − 0.5·4-s + 0.377i·7-s − 0.353i·8-s − 0.603·11-s + 0.277i·13-s − 0.267·14-s + 0.250·16-s − 0.242i·17-s − 0.917·19-s − 0.426i·22-s − 1.45i·23-s − 0.196·26-s − 0.188i·28-s + 0.185·29-s + ⋯

Functional equation

Λ(s)=(3150s/2 ΓC(s) L(s)=((0.894+0.447i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 3150 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.894 + 0.447i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(3150s/2 ΓC(s+1/2) L(s)=((0.894+0.447i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 3150 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.894 + 0.447i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 31503150    =    2⋅32⋅52⋅72 \cdot 3^{2} \cdot 5^{2} \cdot 7
Sign: 0.894+0.447i0.894 + 0.447i
Analytic conductor: 25.152825.1528
Root analytic conductor: 5.015265.01526
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ3150(2899,⋅)\chi_{3150} (2899, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 3150, ( :1/2), 0.894+0.447i)(2,\ 3150,\ (\ :1/2),\ 0.894 + 0.447i)

Particular Values

L(1)L(1) ≈\approx 1.0984151331.098415133
L(12)L(\frac12) ≈\approx 1.0984151331.098415133
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1−iT 1 - iT
3 1 1
5 1 1
7 1−iT 1 - iT
good11 1+2T+11T2 1 + 2T + 11T^{2}
13 1−iT−13T2 1 - iT - 13T^{2}
17 1+iT−17T2 1 + iT - 17T^{2}
19 1+4T+19T2 1 + 4T + 19T^{2}
23 1+7iT−23T2 1 + 7iT - 23T^{2}
29 1−T+29T2 1 - T + 29T^{2}
31 1−3T+31T2 1 - 3T + 31T^{2}
37 1−6iT−37T2 1 - 6iT - 37T^{2}
41 1−3T+41T2 1 - 3T + 41T^{2}
43 1+iT−43T2 1 + iT - 43T^{2}
47 1+12iT−47T2 1 + 12iT - 47T^{2}
53 1+11iT−53T2 1 + 11iT - 53T^{2}
59 1+3T+59T2 1 + 3T + 59T^{2}
61 1−5T+61T2 1 - 5T + 61T^{2}
67 1−12iT−67T2 1 - 12iT - 67T^{2}
71 1+4T+71T2 1 + 4T + 71T^{2}
73 1+14iT−73T2 1 + 14iT - 73T^{2}
79 1−2T+79T2 1 - 2T + 79T^{2}
83 1−3iT−83T2 1 - 3iT - 83T^{2}
89 1−10T+89T2 1 - 10T + 89T^{2}
97 1−10iT−97T2 1 - 10iT - 97T^{2}
show more
show less
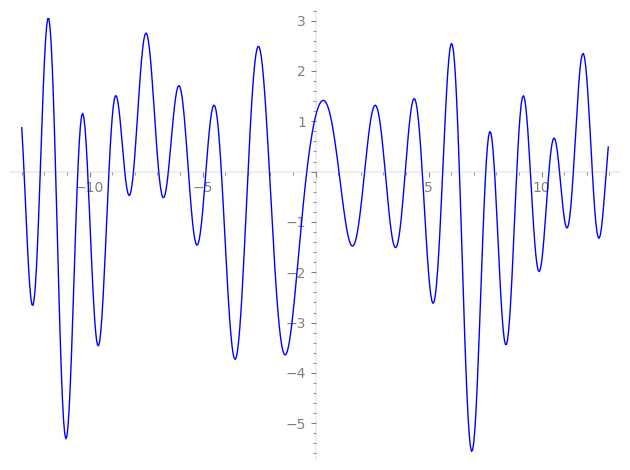
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−8.441209391517775762363458788091, −8.056352970138622546857262447687, −6.93824515043766526694219211083, −6.51388161801017148728456699899, −5.62442206046540222346720984564, −4.85295102670311579135486305208, −4.16767890302369733025766307495, −2.98695522803573316007910573951, −2.04139695684873212623534625709, −0.38891980352997469944515553764, 1.03404631717384617889362410988, 2.17203488202553299466463835934, 3.08103096442649605749197867140, 3.96838017352575114936592203683, 4.71876505107304421530976423227, 5.62609604427547737567948586981, 6.36490624378062815697104960504, 7.52875966659886035518224209237, 7.917622718233711942763675610896, 8.902186558754098285799163241864

Graph of the ZZ-function along the critical line