↑

Properties

Label 8-3332e4-1.1-c0e4-0-6
Degree $8$
Conductor $1.233\times 10^{14}$
Sign $1$
Analytic cond. $7.64624$
Root an. cond. $1.28952$
Motivic weight $0$
Arithmetic yes
Rational yes
Primitive no
Self-dual yes
Analytic rank $0$

Origins

Origins of factors

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  − 16-s − 2·25-s + 4·37-s + 4·41-s − 4·53-s + 4·61-s + 8·101-s − 4·109-s + 127-s + 131-s + 137-s + 139-s + 149-s + 151-s + 157-s + 163-s + 167-s + 4·169-s + 173-s + 179-s + 181-s + 191-s + 193-s + 197-s + 199-s + 211-s + 223-s + ⋯
L(s)  = 1  − 16-s − 2·25-s + 4·37-s + 4·41-s − 4·53-s + 4·61-s + 8·101-s − 4·109-s + 127-s + 131-s + 137-s + 139-s + 149-s + 151-s + 157-s + 163-s + 167-s + 4·169-s + 173-s + 179-s + 181-s + 191-s + 193-s + 197-s + 199-s + 211-s + 223-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut &\left(2^{8} \cdot 7^{8} \cdot 17^{4}\right)^{s/2} \, \Gamma_{\C}(s)^{4} \, L(s)\cr=\mathstrut & \,\Lambda(1-s)\end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut &\left(2^{8} \cdot 7^{8} \cdot 17^{4}\right)^{s/2} \, \Gamma_{\C}(s)^{4} \, L(s)\cr=\mathstrut & \,\Lambda(1-s)\end{aligned}\]

Invariants

Degree: \(8\)
Conductor: \(2^{8} \cdot 7^{8} \cdot 17^{4}\)
Sign: $1$
Analytic conductor: \(7.64624\)
Root analytic conductor: \(1.28952\)
Motivic weight: \(0\)
Rational: yes
Arithmetic: yes
Character: Trivial
Primitive: no
Self-dual: yes
Analytic rank: \(0\)
Selberg data: \((8,\ 2^{8} \cdot 7^{8} \cdot 17^{4} ,\ ( \ : 0, 0, 0, 0 ),\ 1 )\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(1.732842717\)
\(L(\frac12)\) \(\approx\) \(1.732842717\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$\Gal(F_p)$$F_p(T)$
bad2$C_2^2$ \( 1 + T^{4} \)
7 \( 1 \)
17$C_2^2$ \( 1 + T^{4} \)
good3$C_4\times C_2$ \( 1 + T^{8} \)
5$C_2$$\times$$C_2^2$ \( ( 1 + T^{2} )^{2}( 1 + T^{4} ) \)
11$C_4\times C_2$ \( 1 + T^{8} \)
13$C_1$$\times$$C_1$ \( ( 1 - T )^{4}( 1 + T )^{4} \)
19$C_2^2$ \( ( 1 + T^{4} )^{2} \)
23$C_4\times C_2$ \( 1 + T^{8} \)
29$C_2$$\times$$C_2^2$ \( ( 1 + T^{2} )^{2}( 1 + T^{4} ) \)
31$C_4\times C_2$ \( 1 + T^{8} \)
37$C_1$$\times$$C_2^2$ \( ( 1 - T )^{4}( 1 + T^{4} ) \)
41$C_1$$\times$$C_2^2$ \( ( 1 - T )^{4}( 1 + T^{4} ) \)
43$C_2^2$ \( ( 1 + T^{4} )^{2} \)
47$C_2$ \( ( 1 + T^{2} )^{4} \)
53$C_1$$\times$$C_2$ \( ( 1 + T )^{4}( 1 + T^{2} )^{2} \)
59$C_2^2$ \( ( 1 + T^{4} )^{2} \)
61$C_1$$\times$$C_2^2$ \( ( 1 - T )^{4}( 1 + T^{4} ) \)
67$C_1$$\times$$C_1$ \( ( 1 - T )^{4}( 1 + T )^{4} \)
71$C_4\times C_2$ \( 1 + T^{8} \)
73$C_2$$\times$$C_2^2$ \( ( 1 + T^{2} )^{2}( 1 + T^{4} ) \)
79$C_4\times C_2$ \( 1 + T^{8} \)
83$C_2^2$ \( ( 1 + T^{4} )^{2} \)
89$C_2$ \( ( 1 + T^{2} )^{4} \)
97$C_2$$\times$$C_2^2$ \( ( 1 + T^{2} )^{2}( 1 + T^{4} ) \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{8} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

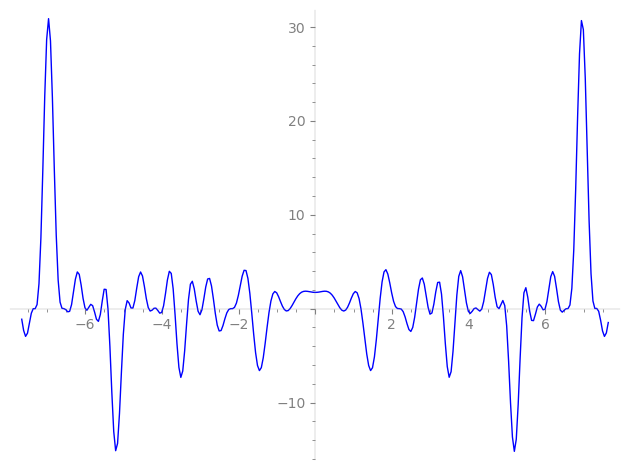
Imaginary part of the first few zeros on the critical line

−6.38074750558724713506387760046, −5.99954975419966063869477084708, −5.92382771393812870142007529452, −5.77978260182117092063982908069, −5.58335395566535234691631687726, −5.40727290182896558775355125325, −4.95418161849566728379002367075, −4.79402301021264576183173810456, −4.76753043535797753058362060340, −4.34711649536263176711536211360, −4.21932873224493985530803065026, −4.14120816936939216910316474815, −3.97289982351400572450958247198, −3.66746285278900886454033567017, −3.32725907116285632353854040213, −3.07206091283863621809561178184, −2.95020549410106364208670631832, −2.62417097887203082597183717696, −2.21442304092380673199566575360, −2.18186718006145966775075437264, −2.16111172548121158707996751811, −1.66724438166004028089889012926, −1.19184740120769111915557366517, −0.821717434010725781486152411641, −0.65721834447309321850082619888, 0.65721834447309321850082619888, 0.821717434010725781486152411641, 1.19184740120769111915557366517, 1.66724438166004028089889012926, 2.16111172548121158707996751811, 2.18186718006145966775075437264, 2.21442304092380673199566575360, 2.62417097887203082597183717696, 2.95020549410106364208670631832, 3.07206091283863621809561178184, 3.32725907116285632353854040213, 3.66746285278900886454033567017, 3.97289982351400572450958247198, 4.14120816936939216910316474815, 4.21932873224493985530803065026, 4.34711649536263176711536211360, 4.76753043535797753058362060340, 4.79402301021264576183173810456, 4.95418161849566728379002367075, 5.40727290182896558775355125325, 5.58335395566535234691631687726, 5.77978260182117092063982908069, 5.92382771393812870142007529452, 5.99954975419966063869477084708, 6.38074750558724713506387760046

Graph of the $Z$-function along the critical line