↑

Properties

Label 2-352-88.69-c1-0-1
Degree $2$
Conductor $352$
Sign $-0.362 - 0.932i$
Analytic cond. $2.81073$
Root an. cond. $1.67652$
Motivic weight $1$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.582 − 0.189i)3-s + (−0.858 + 1.18i)5-s + (−1.36 + 4.21i)7-s + (−2.12 + 1.54i)9-s + (−2.69 + 1.93i)11-s + (−3.27 − 4.50i)13-s + (−0.276 + 0.850i)15-s + (2.06 + 1.50i)17-s + (2.87 − 0.933i)19-s + 2.71i·21-s + 3.70·23-s + (0.886 + 2.72i)25-s + (−2.02 + 2.78i)27-s + (3.48 + 1.13i)29-s + (1.20 − 0.876i)31-s + ⋯
L(s)  = 1  + (0.336 − 0.109i)3-s + (−0.383 + 0.528i)5-s + (−0.517 + 1.59i)7-s + (−0.707 + 0.514i)9-s + (−0.811 + 0.584i)11-s + (−0.908 − 1.25i)13-s + (−0.0713 + 0.219i)15-s + (0.501 + 0.364i)17-s + (0.658 − 0.214i)19-s + 0.591i·21-s + 0.773·23-s + (0.177 + 0.545i)25-s + (−0.389 + 0.536i)27-s + (0.646 + 0.209i)29-s + (0.216 − 0.157i)31-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 352 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.362 - 0.932i)\, \overline{\Lambda}(2-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 352 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (-0.362 - 0.932i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(352\)    =    \(2^{5} \cdot 11\)
Sign: $-0.362 - 0.932i$
Analytic conductor: \(2.81073\)
Root analytic conductor: \(1.67652\)
Motivic weight: \(1\)
Rational: no
Arithmetic: yes
Character: $\chi_{352} (113, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 352,\ (\ :1/2),\ -0.362 - 0.932i)\)

Particular Values

\(L(1)\) \(\approx\) \(0.538109 + 0.786521i\)
\(L(\frac12)\) \(\approx\) \(0.538109 + 0.786521i\)
\(L(\frac{3}{2})\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad2 \( 1 \)
11 \( 1 + (2.69 - 1.93i)T \)
good3 \( 1 + (-0.582 + 0.189i)T + (2.42 - 1.76i)T^{2} \)
5 \( 1 + (0.858 - 1.18i)T + (-1.54 - 4.75i)T^{2} \)
7 \( 1 + (1.36 - 4.21i)T + (-5.66 - 4.11i)T^{2} \)
13 \( 1 + (3.27 + 4.50i)T + (-4.01 + 12.3i)T^{2} \)
17 \( 1 + (-2.06 - 1.50i)T + (5.25 + 16.1i)T^{2} \)
19 \( 1 + (-2.87 + 0.933i)T + (15.3 - 11.1i)T^{2} \)
23 \( 1 - 3.70T + 23T^{2} \)
29 \( 1 + (-3.48 - 1.13i)T + (23.4 + 17.0i)T^{2} \)
31 \( 1 + (-1.20 + 0.876i)T + (9.57 - 29.4i)T^{2} \)
37 \( 1 + (-1.41 - 0.460i)T + (29.9 + 21.7i)T^{2} \)
41 \( 1 + (-0.970 - 2.98i)T + (-33.1 + 24.0i)T^{2} \)
43 \( 1 + 6.25iT - 43T^{2} \)
47 \( 1 + (-0.821 - 2.52i)T + (-38.0 + 27.6i)T^{2} \)
53 \( 1 + (-5.75 - 7.92i)T + (-16.3 + 50.4i)T^{2} \)
59 \( 1 + (-2.45 - 0.796i)T + (47.7 + 34.6i)T^{2} \)
61 \( 1 + (3.28 - 4.51i)T + (-18.8 - 58.0i)T^{2} \)
67 \( 1 - 11.5iT - 67T^{2} \)
71 \( 1 + (-0.909 - 0.660i)T + (21.9 + 67.5i)T^{2} \)
73 \( 1 + (-4.36 + 13.4i)T + (-59.0 - 42.9i)T^{2} \)
79 \( 1 + (-0.619 + 0.450i)T + (24.4 - 75.1i)T^{2} \)
83 \( 1 + (5.03 - 6.92i)T + (-25.6 - 78.9i)T^{2} \)
89 \( 1 + 8.84T + 89T^{2} \)
97 \( 1 + (-0.241 + 0.175i)T + (29.9 - 92.2i)T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

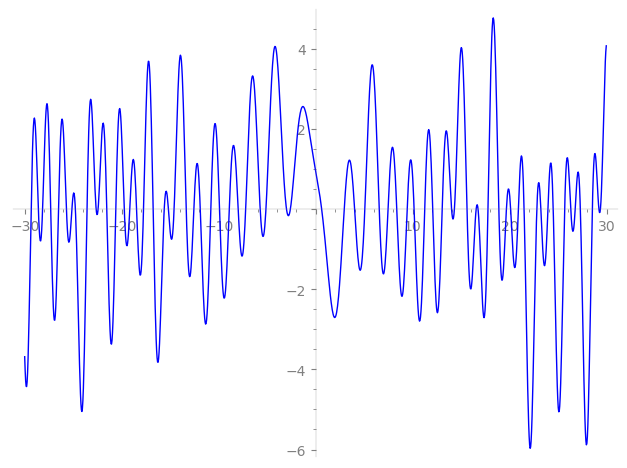
Imaginary part of the first few zeros on the critical line

−11.90182850425433833496953353769, −10.81498168967777664590787163123, −9.916550581096086060846570344136, −8.913548855960224507622993697164, −7.993495003839717367719290363504, −7.22299053829820748943282944406, −5.72467065889602698625336004896, −5.13292582710670235986446925545, −3.00911810451099059063107542960, −2.61721445623282846499850796311, 0.61388405386499024225865285607, 2.93732452195337259853817671863, 4.00870037131012106789797311325, 5.06142786117801898677767509941, 6.54598665635207576652885652312, 7.45185210938260658276553600247, 8.352333335279250178355384992024, 9.424428668279162609352686214967, 10.14199365434962644369535468032, 11.23303357930251296268241409641

Graph of the $Z$-function along the critical line