↑

Properties

Label 2-3584-224.69-c0-0-2
Degree $2$
Conductor $3584$
Sign $0.555 - 0.831i$
Analytic cond. $1.78864$
Root an. cond. $1.33740$
Motivic weight $0$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.707 + 0.707i)7-s + (−0.707 + 0.707i)9-s + (0.292 − 0.707i)11-s + (−1 + i)23-s + (0.707 + 0.707i)25-s + (0.292 + 0.707i)29-s + (1.70 + 0.707i)37-s + (0.707 − 1.70i)43-s + 1.00i·49-s + (−0.707 + 1.70i)53-s − 1.00·63-s + (−0.707 − 1.70i)67-s + (0.707 − 0.292i)77-s + 1.41i·79-s − 1.00i·81-s + ⋯
L(s)  = 1  + (0.707 + 0.707i)7-s + (−0.707 + 0.707i)9-s + (0.292 − 0.707i)11-s + (−1 + i)23-s + (0.707 + 0.707i)25-s + (0.292 + 0.707i)29-s + (1.70 + 0.707i)37-s + (0.707 − 1.70i)43-s + 1.00i·49-s + (−0.707 + 1.70i)53-s − 1.00·63-s + (−0.707 − 1.70i)67-s + (0.707 − 0.292i)77-s + 1.41i·79-s − 1.00i·81-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 3584 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.555 - 0.831i)\, \overline{\Lambda}(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 3584 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.555 - 0.831i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(3584\)    =    \(2^{9} \cdot 7\)
Sign: $0.555 - 0.831i$
Analytic conductor: \(1.78864\)
Root analytic conductor: \(1.33740\)
Motivic weight: \(0\)
Rational: no
Arithmetic: yes
Character: $\chi_{3584} (3009, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 3584,\ (\ :0),\ 0.555 - 0.831i)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(1.275218929\)
\(L(\frac12)\) \(\approx\) \(1.275218929\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad2 \( 1 \)
7 \( 1 + (-0.707 - 0.707i)T \)
good3 \( 1 + (0.707 - 0.707i)T^{2} \)
5 \( 1 + (-0.707 - 0.707i)T^{2} \)
11 \( 1 + (-0.292 + 0.707i)T + (-0.707 - 0.707i)T^{2} \)
13 \( 1 + (-0.707 + 0.707i)T^{2} \)
17 \( 1 + T^{2} \)
19 \( 1 + (-0.707 + 0.707i)T^{2} \)
23 \( 1 + (1 - i)T - iT^{2} \)
29 \( 1 + (-0.292 - 0.707i)T + (-0.707 + 0.707i)T^{2} \)
31 \( 1 - T^{2} \)
37 \( 1 + (-1.70 - 0.707i)T + (0.707 + 0.707i)T^{2} \)
41 \( 1 + iT^{2} \)
43 \( 1 + (-0.707 + 1.70i)T + (-0.707 - 0.707i)T^{2} \)
47 \( 1 + T^{2} \)
53 \( 1 + (0.707 - 1.70i)T + (-0.707 - 0.707i)T^{2} \)
59 \( 1 + (-0.707 - 0.707i)T^{2} \)
61 \( 1 + (0.707 - 0.707i)T^{2} \)
67 \( 1 + (0.707 + 1.70i)T + (-0.707 + 0.707i)T^{2} \)
71 \( 1 + iT^{2} \)
73 \( 1 + iT^{2} \)
79 \( 1 - 1.41iT - T^{2} \)
83 \( 1 + (-0.707 + 0.707i)T^{2} \)
89 \( 1 - iT^{2} \)
97 \( 1 - T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

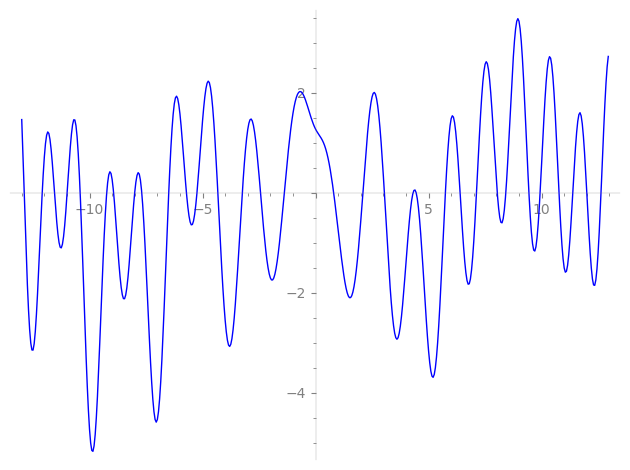
Imaginary part of the first few zeros on the critical line

−8.935072212308500282125009767967, −8.003055556160490830667970849485, −7.68912260019325639425251617179, −6.49644311024527566743766557059, −5.71237380976683009218298044170, −5.24749622346762657890946317926, −4.32309214743614810578938141672, −3.24167093855471127612065672866, −2.43467870799578720931788950688, −1.38353307970384567398214102261, 0.800750680784287917208046400652, 2.09369384177325243478475484454, 3.04445845460318611117030602416, 4.31243237005727480693327736918, 4.45194238776571933584332476643, 5.74346815093206601266829786547, 6.39539091510184593201753401676, 7.11936918972780292562604534109, 8.039133087192735715250182288606, 8.415833442581956001093067034724

Graph of the $Z$-function along the critical line