↑

Properties

Label 2-735-7.4-c1-0-20
Degree 22
Conductor 735735
Sign −0.701+0.712i-0.701 + 0.712i
Analytic cond. 5.869005.86900
Root an. cond. 2.422602.42260
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−0.5 − 0.866i)2-s + (0.5 − 0.866i)3-s + (0.500 − 0.866i)4-s + (0.5 + 0.866i)5-s − 0.999·6-s − 3·8-s + (−0.499 − 0.866i)9-s + (0.499 − 0.866i)10-s + (−0.499 − 0.866i)12-s + 6·13-s + 0.999·15-s + (0.500 + 0.866i)16-s + (1 − 1.73i)17-s + (−0.499 + 0.866i)18-s + (−4 − 6.92i)19-s + 20-s + ⋯
L(s)  = 1  + (−0.353 − 0.612i)2-s + (0.288 − 0.499i)3-s + (0.250 − 0.433i)4-s + (0.223 + 0.387i)5-s − 0.408·6-s − 1.06·8-s + (−0.166 − 0.288i)9-s + (0.158 − 0.273i)10-s + (−0.144 − 0.249i)12-s + 1.66·13-s + 0.258·15-s + (0.125 + 0.216i)16-s + (0.242 − 0.420i)17-s + (−0.117 + 0.204i)18-s + (−0.917 − 1.58i)19-s + 0.223·20-s + ⋯

Functional equation

Λ(s)=(735s/2 ΓC(s) L(s)=((−0.701+0.712i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 735 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.701 + 0.712i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(735s/2 ΓC(s+1/2) L(s)=((−0.701+0.712i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 735 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (-0.701 + 0.712i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 735735    =    3⋅5⋅723 \cdot 5 \cdot 7^{2}
Sign: −0.701+0.712i-0.701 + 0.712i
Analytic conductor: 5.869005.86900
Root analytic conductor: 2.422602.42260
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ735(361,⋅)\chi_{735} (361, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 735, ( :1/2), −0.701+0.712i)(2,\ 735,\ (\ :1/2),\ -0.701 + 0.712i)

Particular Values

L(1)L(1) ≈\approx 0.555565−1.32566i0.555565 - 1.32566i
L(12)L(\frac12) ≈\approx 0.555565−1.32566i0.555565 - 1.32566i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad3 1+(−0.5+0.866i)T 1 + (-0.5 + 0.866i)T
5 1+(−0.5−0.866i)T 1 + (-0.5 - 0.866i)T
7 1 1
good2 1+(0.5+0.866i)T+(−1+1.73i)T2 1 + (0.5 + 0.866i)T + (-1 + 1.73i)T^{2}
11 1+(−5.5−9.52i)T2 1 + (-5.5 - 9.52i)T^{2}
13 1−6T+13T2 1 - 6T + 13T^{2}
17 1+(−1+1.73i)T+(−8.5−14.7i)T2 1 + (-1 + 1.73i)T + (-8.5 - 14.7i)T^{2}
19 1+(4+6.92i)T+(−9.5+16.4i)T2 1 + (4 + 6.92i)T + (-9.5 + 16.4i)T^{2}
23 1+(4+6.92i)T+(−11.5+19.9i)T2 1 + (4 + 6.92i)T + (-11.5 + 19.9i)T^{2}
29 1+2T+29T2 1 + 2T + 29T^{2}
31 1+(−2+3.46i)T+(−15.5−26.8i)T2 1 + (-2 + 3.46i)T + (-15.5 - 26.8i)T^{2}
37 1+(−1−1.73i)T+(−18.5+32.0i)T2 1 + (-1 - 1.73i)T + (-18.5 + 32.0i)T^{2}
41 1−6T+41T2 1 - 6T + 41T^{2}
43 1−4T+43T2 1 - 4T + 43T^{2}
47 1+(−4−6.92i)T+(−23.5+40.7i)T2 1 + (-4 - 6.92i)T + (-23.5 + 40.7i)T^{2}
53 1+(5−8.66i)T+(−26.5−45.8i)T2 1 + (5 - 8.66i)T + (-26.5 - 45.8i)T^{2}
59 1+(−2+3.46i)T+(−29.5−51.0i)T2 1 + (-2 + 3.46i)T + (-29.5 - 51.0i)T^{2}
61 1+(1+1.73i)T+(−30.5+52.8i)T2 1 + (1 + 1.73i)T + (-30.5 + 52.8i)T^{2}
67 1+(2−3.46i)T+(−33.5−58.0i)T2 1 + (2 - 3.46i)T + (-33.5 - 58.0i)T^{2}
71 1+12T+71T2 1 + 12T + 71T^{2}
73 1+(1−1.73i)T+(−36.5−63.2i)T2 1 + (1 - 1.73i)T + (-36.5 - 63.2i)T^{2}
79 1+(4+6.92i)T+(−39.5+68.4i)T2 1 + (4 + 6.92i)T + (-39.5 + 68.4i)T^{2}
83 1−4T+83T2 1 - 4T + 83T^{2}
89 1+(3+5.19i)T+(−44.5+77.0i)T2 1 + (3 + 5.19i)T + (-44.5 + 77.0i)T^{2}
97 1−18T+97T2 1 - 18T + 97T^{2}
show more
show less
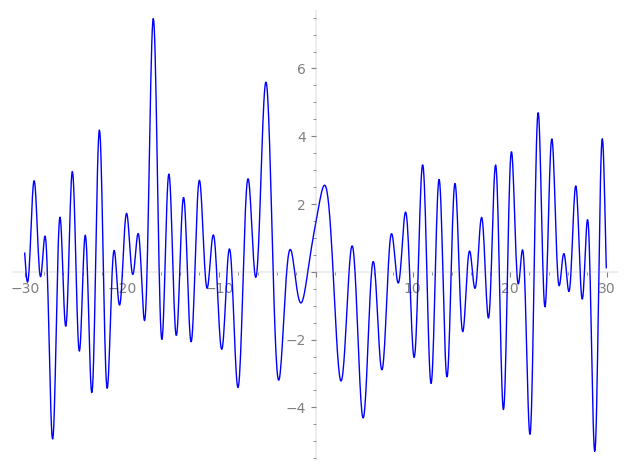
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.21513019721889744588714716247, −9.142671875229193952026188171827, −8.652693295096881803388888080875, −7.46699723572523875021634620936, −6.30232677462348876300017408378, −6.06215823738007843248542834061, −4.38811972194348669394918723538, −2.99376094750741457280557848510, −2.17265511341079055014304820807, −0.816880209091725251028262654467, 1.79144008333543833352681486237, 3.43584376871147869793226047999, 4.05053938266912190787151039746, 5.75599649755096734032464695844, 6.10687461110529749998803352213, 7.45495127667200245001312433880, 8.287735619010345288068790827385, 8.714492154223897861050509021309, 9.673084331152652731725376117625, 10.56324952502121165516736973958

Graph of the ZZ-function along the critical line