↑

Properties

Label 2-867-51.50-c0-0-1
Degree 22
Conductor 867867
Sign 0.242+0.970i0.242 + 0.970i
Analytic cond. 0.4326890.432689
Root an. cond. 0.6577910.657791
Motivic weight 00
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  − i·3-s + 4-s − i·7-s − 9-s − i·12-s − 13-s + 16-s + 19-s − 21-s − 25-s + i·27-s − i·28-s + i·31-s − 36-s + i·37-s + ⋯
L(s)  = 1  − i·3-s + 4-s − i·7-s − 9-s − i·12-s − 13-s + 16-s + 19-s − 21-s − 25-s + i·27-s − i·28-s + i·31-s − 36-s + i·37-s + ⋯

Functional equation

Λ(s)=(867s/2 ΓC(s) L(s)=((0.242+0.970i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 867 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.242 + 0.970i)\, \overline{\Lambda}(1-s) \end{aligned}
Λ(s)=(867s/2 ΓC(s) L(s)=((0.242+0.970i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 867 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.242 + 0.970i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 867867    =    3⋅1723 \cdot 17^{2}
Sign: 0.242+0.970i0.242 + 0.970i
Analytic conductor: 0.4326890.432689
Root analytic conductor: 0.6577910.657791
Motivic weight: 00
Rational: no
Arithmetic: yes
Character: χ867(866,⋅)\chi_{867} (866, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 867, ( :0), 0.242+0.970i)(2,\ 867,\ (\ :0),\ 0.242 + 0.970i)

Particular Values

L(12)L(\frac{1}{2}) ≈\approx 1.1604251971.160425197
L(12)L(\frac12) ≈\approx 1.1604251971.160425197
L(1)L(1) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad3 1+iT 1 + iT
17 1 1
good2 1−T2 1 - T^{2}
5 1+T2 1 + T^{2}
7 1+iT−T2 1 + iT - T^{2}
11 1+T2 1 + T^{2}
13 1+T+T2 1 + T + T^{2}
19 1−T+T2 1 - T + T^{2}
23 1+T2 1 + T^{2}
29 1+T2 1 + T^{2}
31 1−iT−T2 1 - iT - T^{2}
37 1−iT−T2 1 - iT - T^{2}
41 1+T2 1 + T^{2}
43 1−T+T2 1 - T + T^{2}
47 1−T2 1 - T^{2}
53 1−T2 1 - T^{2}
59 1−T2 1 - T^{2}
61 1+iT−T2 1 + iT - T^{2}
67 1+T+T2 1 + T + T^{2}
71 1+T2 1 + T^{2}
73 1+2iT−T2 1 + 2iT - T^{2}
79 1−2iT−T2 1 - 2iT - T^{2}
83 1−T2 1 - T^{2}
89 1−T2 1 - T^{2}
97 1−iT−T2 1 - iT - T^{2}
show more
show less
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

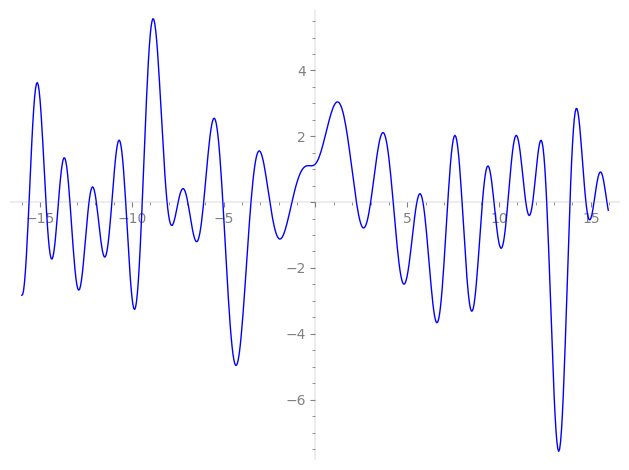
−10.33767084617862649312571636417, −9.436042633965790400104060375776, −8.082946923644960185747051369010, −7.47840772732253939270171255021, −6.95648980062904258100146040314, −6.08873293668499679089115136713, −5.04471798888127980928689362369, −3.51199453032489949428313646278, −2.48269598618486767460476972861, −1.28083172523360863352780036711, 2.23116220643134679818699238569, 3.00651133261333091032583759329, 4.23371768031106467026078435975, 5.52547231356637765662424170241, 5.85362616513724557997568557462, 7.20775196127926524992927847255, 7.985556402735614193597198120505, 9.094861609146242046503673493876, 9.724572920709902619385376824533, 10.48063571240119516119357018170

Graph of the ZZ-function along the critical line