↑

Properties

Label 2-980-28.3-c1-0-65
Degree 22
Conductor 980980
Sign 0.239+0.970i0.239 + 0.970i
Analytic cond. 7.825337.82533
Root an. cond. 2.797382.79738
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.569 + 1.29i)2-s + (1.51 − 2.62i)3-s + (−1.35 + 1.47i)4-s + (−0.866 + 0.5i)5-s + (4.25 + 0.465i)6-s + (−2.67 − 0.908i)8-s + (−3.08 − 5.33i)9-s + (−1.14 − 0.836i)10-s + (−1.03 − 0.598i)11-s + (1.82 + 5.77i)12-s − 4.83i·13-s + 3.02i·15-s + (−0.349 − 3.98i)16-s + (−2.20 − 1.27i)17-s + (5.15 − 7.02i)18-s + (−0.711 − 1.23i)19-s + ⋯
L(s)  = 1  + (0.402 + 0.915i)2-s + (0.873 − 1.51i)3-s + (−0.675 + 0.737i)4-s + (−0.387 + 0.223i)5-s + (1.73 + 0.190i)6-s + (−0.946 − 0.321i)8-s + (−1.02 − 1.77i)9-s + (−0.360 − 0.264i)10-s + (−0.312 − 0.180i)11-s + (0.525 + 1.66i)12-s − 1.34i·13-s + 0.781i·15-s + (−0.0873 − 0.996i)16-s + (−0.534 − 0.308i)17-s + (1.21 − 1.65i)18-s + (−0.163 − 0.282i)19-s + ⋯

Functional equation

Λ(s)=(980s/2 ΓC(s) L(s)=((0.239+0.970i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 980 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.239 + 0.970i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(980s/2 ΓC(s+1/2) L(s)=((0.239+0.970i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 980 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.239 + 0.970i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 980980    =    22⋅5⋅722^{2} \cdot 5 \cdot 7^{2}
Sign: 0.239+0.970i0.239 + 0.970i
Analytic conductor: 7.825337.82533
Root analytic conductor: 2.797382.79738
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ980(31,⋅)\chi_{980} (31, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 980, ( :1/2), 0.239+0.970i)(2,\ 980,\ (\ :1/2),\ 0.239 + 0.970i)

Particular Values

L(1)L(1) ≈\approx 1.38629−1.08553i1.38629 - 1.08553i
L(12)L(\frac12) ≈\approx 1.38629−1.08553i1.38629 - 1.08553i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1+(−0.569−1.29i)T 1 + (-0.569 - 1.29i)T
5 1+(0.866−0.5i)T 1 + (0.866 - 0.5i)T
7 1 1
good3 1+(−1.51+2.62i)T+(−1.5−2.59i)T2 1 + (-1.51 + 2.62i)T + (-1.5 - 2.59i)T^{2}
11 1+(1.03+0.598i)T+(5.5+9.52i)T2 1 + (1.03 + 0.598i)T + (5.5 + 9.52i)T^{2}
13 1+4.83iT−13T2 1 + 4.83iT - 13T^{2}
17 1+(2.20+1.27i)T+(8.5+14.7i)T2 1 + (2.20 + 1.27i)T + (8.5 + 14.7i)T^{2}
19 1+(0.711+1.23i)T+(−9.5+16.4i)T2 1 + (0.711 + 1.23i)T + (-9.5 + 16.4i)T^{2}
23 1+(−5.02+2.90i)T+(11.5−19.9i)T2 1 + (-5.02 + 2.90i)T + (11.5 - 19.9i)T^{2}
29 1−0.774T+29T2 1 - 0.774T + 29T^{2}
31 1+(−3.31+5.74i)T+(−15.5−26.8i)T2 1 + (-3.31 + 5.74i)T + (-15.5 - 26.8i)T^{2}
37 1+(2.55+4.42i)T+(−18.5+32.0i)T2 1 + (2.55 + 4.42i)T + (-18.5 + 32.0i)T^{2}
41 1−7.46iT−41T2 1 - 7.46iT - 41T^{2}
43 1−1.38iT−43T2 1 - 1.38iT - 43T^{2}
47 1+(−0.535−0.927i)T+(−23.5+40.7i)T2 1 + (-0.535 - 0.927i)T + (-23.5 + 40.7i)T^{2}
53 1+(1.68−2.91i)T+(−26.5−45.8i)T2 1 + (1.68 - 2.91i)T + (-26.5 - 45.8i)T^{2}
59 1+(4.94−8.55i)T+(−29.5−51.0i)T2 1 + (4.94 - 8.55i)T + (-29.5 - 51.0i)T^{2}
61 1+(8.31−4.79i)T+(30.5−52.8i)T2 1 + (8.31 - 4.79i)T + (30.5 - 52.8i)T^{2}
67 1+(−9.14−5.27i)T+(33.5+58.0i)T2 1 + (-9.14 - 5.27i)T + (33.5 + 58.0i)T^{2}
71 1+16.3iT−71T2 1 + 16.3iT - 71T^{2}
73 1+(0.0927+0.0535i)T+(36.5+63.2i)T2 1 + (0.0927 + 0.0535i)T + (36.5 + 63.2i)T^{2}
79 1+(9.32−5.38i)T+(39.5−68.4i)T2 1 + (9.32 - 5.38i)T + (39.5 - 68.4i)T^{2}
83 1−15.8T+83T2 1 - 15.8T + 83T^{2}
89 1+(−3.41+1.97i)T+(44.5−77.0i)T2 1 + (-3.41 + 1.97i)T + (44.5 - 77.0i)T^{2}
97 1+8.71iT−97T2 1 + 8.71iT - 97T^{2}
show more
show less
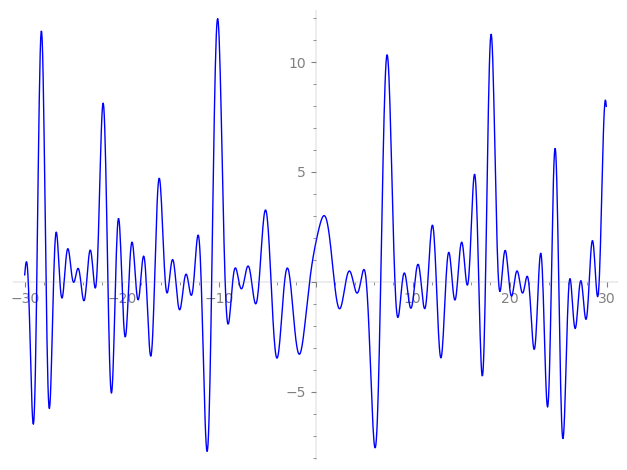
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−9.335923765280315664536050344983, −8.562541074442241853861304533715, −7.915954407709107940407650895124, −7.39961046982278343803505742980, −6.60632696550023502152682907288, −5.84271101326127364863208716370, −4.60334226082875792186594821143, −3.21250472918432122987530023448, −2.62057678040086249379826973770, −0.63329472521355241934001442928, 1.91919614013487304687550444561, 3.09931635675502347998600982868, 3.86508032815955943935641189172, 4.63275135654475363870115122659, 5.21413789892317399748465023730, 6.69815890871022212794950435402, 8.165400368993681224590323890543, 8.924234887900229986692676141322, 9.357248251772116565545640002688, 10.22788558469297428229182374446

Graph of the ZZ-function along the critical line