↑

Properties

Label 2-3040-760.619-c0-0-1
Degree 22
Conductor 30403040
Sign 0.671+0.740i0.671 + 0.740i
Analytic cond. 1.517151.51715
Root an. cond. 1.231721.23172
Motivic weight 00
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−0.5 + 0.866i)5-s + 7-s + (−0.5 − 0.866i)9-s + 11-s + (−1 − 1.73i)13-s + (0.5 − 0.866i)19-s + (−0.5 − 0.866i)23-s + (−0.499 − 0.866i)25-s + (−0.5 + 0.866i)35-s − 37-s + (0.5 − 0.866i)41-s + 0.999·45-s + (1 + 1.73i)47-s + (0.5 + 0.866i)53-s + (−0.5 + 0.866i)55-s + ⋯
L(s)  = 1  + (−0.5 + 0.866i)5-s + 7-s + (−0.5 − 0.866i)9-s + 11-s + (−1 − 1.73i)13-s + (0.5 − 0.866i)19-s + (−0.5 − 0.866i)23-s + (−0.499 − 0.866i)25-s + (−0.5 + 0.866i)35-s − 37-s + (0.5 − 0.866i)41-s + 0.999·45-s + (1 + 1.73i)47-s + (0.5 + 0.866i)53-s + (−0.5 + 0.866i)55-s + ⋯

Functional equation

Λ(s)=(3040s/2 ΓC(s) L(s)=((0.671+0.740i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 3040 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.671 + 0.740i)\, \overline{\Lambda}(1-s) \end{aligned}
Λ(s)=(3040s/2 ΓC(s) L(s)=((0.671+0.740i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 3040 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.671 + 0.740i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 30403040    =    25⋅5⋅192^{5} \cdot 5 \cdot 19
Sign: 0.671+0.740i0.671 + 0.740i
Analytic conductor: 1.517151.51715
Root analytic conductor: 1.231721.23172
Motivic weight: 00
Rational: no
Arithmetic: yes
Character: χ3040(239,⋅)\chi_{3040} (239, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 3040, ( :0), 0.671+0.740i)(2,\ 3040,\ (\ :0),\ 0.671 + 0.740i)

Particular Values

L(12)L(\frac{1}{2}) ≈\approx 1.1463417051.146341705
L(12)L(\frac12) ≈\approx 1.1463417051.146341705
L(1)L(1) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1 1
5 1+(0.5−0.866i)T 1 + (0.5 - 0.866i)T
19 1+(−0.5+0.866i)T 1 + (-0.5 + 0.866i)T
good3 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
7 1−T+T2 1 - T + T^{2}
11 1−T+T2 1 - T + T^{2}
13 1+(1+1.73i)T+(−0.5+0.866i)T2 1 + (1 + 1.73i)T + (-0.5 + 0.866i)T^{2}
17 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
23 1+(0.5+0.866i)T+(−0.5+0.866i)T2 1 + (0.5 + 0.866i)T + (-0.5 + 0.866i)T^{2}
29 1+(0.5−0.866i)T2 1 + (0.5 - 0.866i)T^{2}
31 1−T2 1 - T^{2}
37 1+T+T2 1 + T + T^{2}
41 1+(−0.5+0.866i)T+(−0.5−0.866i)T2 1 + (-0.5 + 0.866i)T + (-0.5 - 0.866i)T^{2}
43 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
47 1+(−1−1.73i)T+(−0.5+0.866i)T2 1 + (-1 - 1.73i)T + (-0.5 + 0.866i)T^{2}
53 1+(−0.5−0.866i)T+(−0.5+0.866i)T2 1 + (-0.5 - 0.866i)T + (-0.5 + 0.866i)T^{2}
59 1+(−1+1.73i)T+(−0.5−0.866i)T2 1 + (-1 + 1.73i)T + (-0.5 - 0.866i)T^{2}
61 1+(0.5−0.866i)T2 1 + (0.5 - 0.866i)T^{2}
67 1+(0.5−0.866i)T2 1 + (0.5 - 0.866i)T^{2}
71 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
73 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
79 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
83 1−T2 1 - T^{2}
89 1+(−0.5−0.866i)T+(−0.5+0.866i)T2 1 + (-0.5 - 0.866i)T + (-0.5 + 0.866i)T^{2}
97 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
show more
show less
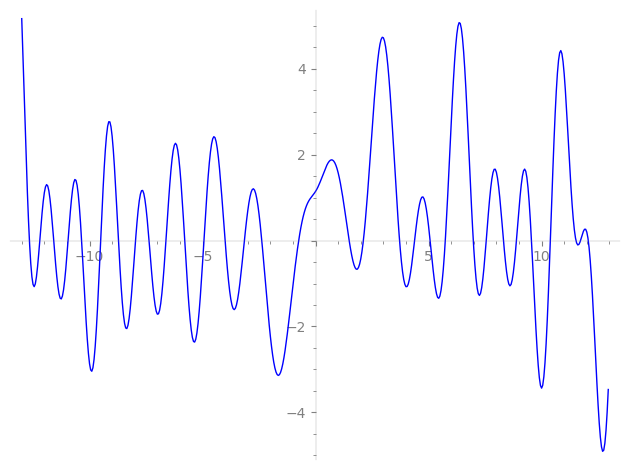
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−8.719707224417906527857920457540, −7.965064455222957655769838325575, −7.36760313654601233156382907999, −6.61962447106984075551930490720, −5.78837685987911999117401301561, −4.93924852574024289387332294438, −4.00222382936215213990925273132, −3.14876284602560299187250097473, −2.37953556364473894614404126086, −0.74695730338733601657553426168, 1.48731107266169626390219365833, 2.11394386211900300612107285870, 3.71668784814152797422293806826, 4.38003906219632290771811946169, 5.06798101386282369704926604996, 5.73887332879638268222262183474, 6.97852297093724148939125358379, 7.55062751389045490258270488112, 8.314671073311699696086634380387, 8.886269989091098314224352124940

Graph of the ZZ-function along the critical line