↑

Properties

Label 2-2156-7.2-c1-0-23
Degree 22
Conductor 21562156
Sign −0.266+0.963i-0.266 + 0.963i
Analytic cond. 17.215717.2157
Root an. cond. 4.149184.14918
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−0.5 − 0.866i)3-s + (−0.5 + 0.866i)5-s + (1 − 1.73i)9-s + (−0.5 − 0.866i)11-s + 4·13-s + 0.999·15-s + (−3 − 5.19i)17-s + (−1 + 1.73i)19-s + (−0.5 + 0.866i)23-s + (2 + 3.46i)25-s − 5·27-s + 2·29-s + (−0.5 − 0.866i)31-s + (−0.499 + 0.866i)33-s + (4.5 − 7.79i)37-s + ⋯
L(s)  = 1  + (−0.288 − 0.499i)3-s + (−0.223 + 0.387i)5-s + (0.333 − 0.577i)9-s + (−0.150 − 0.261i)11-s + 1.10·13-s + 0.258·15-s + (−0.727 − 1.26i)17-s + (−0.229 + 0.397i)19-s + (−0.104 + 0.180i)23-s + (0.400 + 0.692i)25-s − 0.962·27-s + 0.371·29-s + (−0.0898 − 0.155i)31-s + (−0.0870 + 0.150i)33-s + (0.739 − 1.28i)37-s + ⋯

Functional equation

Λ(s)=(2156s/2 ΓC(s) L(s)=((−0.266+0.963i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 2156 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.266 + 0.963i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(2156s/2 ΓC(s+1/2) L(s)=((−0.266+0.963i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 2156 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (-0.266 + 0.963i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 21562156    =    22⋅72⋅112^{2} \cdot 7^{2} \cdot 11
Sign: −0.266+0.963i-0.266 + 0.963i
Analytic conductor: 17.215717.2157
Root analytic conductor: 4.149184.14918
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ2156(177,⋅)\chi_{2156} (177, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 2156, ( :1/2), −0.266+0.963i)(2,\ 2156,\ (\ :1/2),\ -0.266 + 0.963i)

Particular Values

L(1)L(1) ≈\approx 1.2504656391.250465639
L(12)L(\frac12) ≈\approx 1.2504656391.250465639
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1 1
7 1 1
11 1+(0.5+0.866i)T 1 + (0.5 + 0.866i)T
good3 1+(0.5+0.866i)T+(−1.5+2.59i)T2 1 + (0.5 + 0.866i)T + (-1.5 + 2.59i)T^{2}
5 1+(0.5−0.866i)T+(−2.5−4.33i)T2 1 + (0.5 - 0.866i)T + (-2.5 - 4.33i)T^{2}
13 1−4T+13T2 1 - 4T + 13T^{2}
17 1+(3+5.19i)T+(−8.5+14.7i)T2 1 + (3 + 5.19i)T + (-8.5 + 14.7i)T^{2}
19 1+(1−1.73i)T+(−9.5−16.4i)T2 1 + (1 - 1.73i)T + (-9.5 - 16.4i)T^{2}
23 1+(0.5−0.866i)T+(−11.5−19.9i)T2 1 + (0.5 - 0.866i)T + (-11.5 - 19.9i)T^{2}
29 1−2T+29T2 1 - 2T + 29T^{2}
31 1+(0.5+0.866i)T+(−15.5+26.8i)T2 1 + (0.5 + 0.866i)T + (-15.5 + 26.8i)T^{2}
37 1+(−4.5+7.79i)T+(−18.5−32.0i)T2 1 + (-4.5 + 7.79i)T + (-18.5 - 32.0i)T^{2}
41 1+6T+41T2 1 + 6T + 41T^{2}
43 1−8T+43T2 1 - 8T + 43T^{2}
47 1+(4−6.92i)T+(−23.5−40.7i)T2 1 + (4 - 6.92i)T + (-23.5 - 40.7i)T^{2}
53 1+(5+8.66i)T+(−26.5+45.8i)T2 1 + (5 + 8.66i)T + (-26.5 + 45.8i)T^{2}
59 1+(−0.5−0.866i)T+(−29.5+51.0i)T2 1 + (-0.5 - 0.866i)T + (-29.5 + 51.0i)T^{2}
61 1+(1−1.73i)T+(−30.5−52.8i)T2 1 + (1 - 1.73i)T + (-30.5 - 52.8i)T^{2}
67 1+(5.5+9.52i)T+(−33.5+58.0i)T2 1 + (5.5 + 9.52i)T + (-33.5 + 58.0i)T^{2}
71 1−11T+71T2 1 - 11T + 71T^{2}
73 1+(7+12.1i)T+(−36.5+63.2i)T2 1 + (7 + 12.1i)T + (-36.5 + 63.2i)T^{2}
79 1+(−7+12.1i)T+(−39.5−68.4i)T2 1 + (-7 + 12.1i)T + (-39.5 - 68.4i)T^{2}
83 1+4T+83T2 1 + 4T + 83T^{2}
89 1+(−6.5+11.2i)T+(−44.5−77.0i)T2 1 + (-6.5 + 11.2i)T + (-44.5 - 77.0i)T^{2}
97 1−9T+97T2 1 - 9T + 97T^{2}
show more
show less
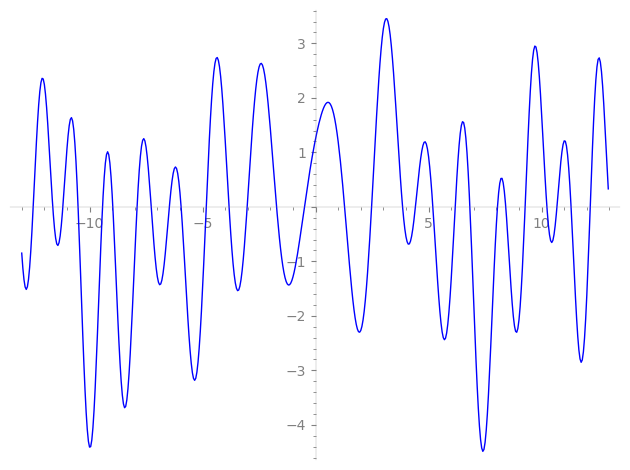
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−8.970223580286368908302998071960, −7.896262769461582017507628713383, −7.27154564983345459006577599381, −6.46861111383483806530564430342, −5.94904164635695306718978825712, −4.83754937906546296450857556127, −3.82746184643946894282068710752, −3.02603202034414322023880640952, −1.72519382814047571503695776360, −0.49293345069549535176435835483, 1.28439983828070620310397239280, 2.47893070609580533230786152337, 3.84991415018960788003360682028, 4.41758361444581985897074544700, 5.18838588539747291173815597652, 6.17791496282122700598820513981, 6.82616851982956168273645930075, 8.048262074180668912546398423150, 8.413600060032320351069710890438, 9.271203085496987075978915162261

Graph of the ZZ-function along the critical line