↑

Properties

Label 2-30e2-180.23-c0-0-1
Degree $2$
Conductor $900$
Sign $0.979 - 0.203i$
Analytic cond. $0.449158$
Root an. cond. $0.670192$
Motivic weight $0$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.965 + 0.258i)2-s + (−0.965 + 0.258i)3-s + (0.866 + 0.499i)4-s − 6-s + (0.448 − 1.67i)7-s + (0.707 + 0.707i)8-s + (0.866 − 0.499i)9-s + (−0.965 − 0.258i)12-s + (0.866 − 1.50i)14-s + (0.500 + 0.866i)16-s + (0.965 − 0.258i)18-s + 1.73i·21-s + (−0.965 + 0.258i)23-s + (−0.866 − 0.5i)24-s + (−0.707 + 0.707i)27-s + (1.22 − 1.22i)28-s + ⋯
L(s)  = 1  + (0.965 + 0.258i)2-s + (−0.965 + 0.258i)3-s + (0.866 + 0.499i)4-s − 6-s + (0.448 − 1.67i)7-s + (0.707 + 0.707i)8-s + (0.866 − 0.499i)9-s + (−0.965 − 0.258i)12-s + (0.866 − 1.50i)14-s + (0.500 + 0.866i)16-s + (0.965 − 0.258i)18-s + 1.73i·21-s + (−0.965 + 0.258i)23-s + (−0.866 − 0.5i)24-s + (−0.707 + 0.707i)27-s + (1.22 − 1.22i)28-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 900 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.979 - 0.203i)\, \overline{\Lambda}(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 900 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.979 - 0.203i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(900\)    =    \(2^{2} \cdot 3^{2} \cdot 5^{2}\)
Sign: $0.979 - 0.203i$
Analytic conductor: \(0.449158\)
Root analytic conductor: \(0.670192\)
Motivic weight: \(0\)
Rational: no
Arithmetic: yes
Character: $\chi_{900} (743, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 900,\ (\ :0),\ 0.979 - 0.203i)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(1.415411283\)
\(L(\frac12)\) \(\approx\) \(1.415411283\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad2 \( 1 + (-0.965 - 0.258i)T \)
3 \( 1 + (0.965 - 0.258i)T \)
5 \( 1 \)
good7 \( 1 + (-0.448 + 1.67i)T + (-0.866 - 0.5i)T^{2} \)
11 \( 1 + (-0.5 + 0.866i)T^{2} \)
13 \( 1 + (0.866 - 0.5i)T^{2} \)
17 \( 1 + iT^{2} \)
19 \( 1 + T^{2} \)
23 \( 1 + (0.965 - 0.258i)T + (0.866 - 0.5i)T^{2} \)
29 \( 1 + (-0.866 - 1.5i)T + (-0.5 + 0.866i)T^{2} \)
31 \( 1 + (0.5 + 0.866i)T^{2} \)
37 \( 1 - iT^{2} \)
41 \( 1 + (1.5 + 0.866i)T + (0.5 + 0.866i)T^{2} \)
43 \( 1 + (0.866 + 0.5i)T^{2} \)
47 \( 1 + (-0.965 - 0.258i)T + (0.866 + 0.5i)T^{2} \)
53 \( 1 - iT^{2} \)
59 \( 1 + (0.5 + 0.866i)T^{2} \)
61 \( 1 + (0.5 + 0.866i)T + (-0.5 + 0.866i)T^{2} \)
67 \( 1 + (1.67 - 0.448i)T + (0.866 - 0.5i)T^{2} \)
71 \( 1 + T^{2} \)
73 \( 1 + iT^{2} \)
79 \( 1 + (-0.5 + 0.866i)T^{2} \)
83 \( 1 + (0.258 - 0.965i)T + (-0.866 - 0.5i)T^{2} \)
89 \( 1 + 1.73T + T^{2} \)
97 \( 1 + (0.866 + 0.5i)T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

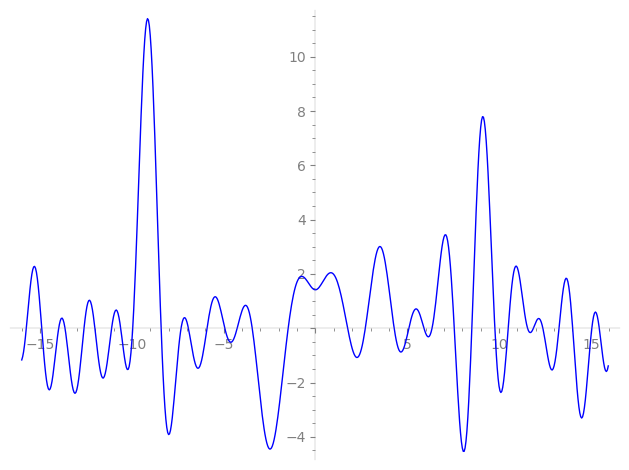
Imaginary part of the first few zeros on the critical line

−10.61024084148005872853728023029, −9.954811926163750646723557801932, −8.406894277197425139893893103049, −7.32072047898068679310585424246, −6.92197437504010791210308431352, −5.90615777641067929495360424540, −4.93889115701135289223646000387, −4.26439175718954740147527536408, −3.44535657682999888105332903457, −1.48653732501967385173032576407, 1.75272355592103926429228939309, 2.71510360452837235332501017325, 4.28726665817755760811856770182, 5.08773990719179494790862416046, 5.91054678387368203948835812751, 6.35007321993085162908791825440, 7.56171563207534452048257380197, 8.518442638723591320252741323745, 9.771875878425240809348035258557, 10.49820389654922155896267784016

Graph of the $Z$-function along the critical line