↑

Properties

Label 2-33e2-99.76-c0-0-1
Degree $2$
Conductor $1089$
Sign $-0.262 + 0.964i$
Analytic cond. $0.543481$
Root an. cond. $0.737212$
Motivic weight $0$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (1.22 − 0.707i)2-s + (0.5 − 0.866i)3-s + (0.499 − 0.866i)4-s + (0.5 − 0.866i)5-s − 1.41i·6-s + (−1.22 + 0.707i)7-s + (−0.499 − 0.866i)9-s − 1.41i·10-s + (−0.5 − 0.866i)12-s + (−0.999 + 1.73i)14-s + (−0.499 − 0.866i)15-s + (0.499 + 0.866i)16-s + (−1.22 − 0.707i)18-s + (−0.5 − 0.866i)20-s + 1.41i·21-s + ⋯
L(s)  = 1  + (1.22 − 0.707i)2-s + (0.5 − 0.866i)3-s + (0.499 − 0.866i)4-s + (0.5 − 0.866i)5-s − 1.41i·6-s + (−1.22 + 0.707i)7-s + (−0.499 − 0.866i)9-s − 1.41i·10-s + (−0.5 − 0.866i)12-s + (−0.999 + 1.73i)14-s + (−0.499 − 0.866i)15-s + (0.499 + 0.866i)16-s + (−1.22 − 0.707i)18-s + (−0.5 − 0.866i)20-s + 1.41i·21-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 1089 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.262 + 0.964i)\, \overline{\Lambda}(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 1089 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.262 + 0.964i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(1089\)    =    \(3^{2} \cdot 11^{2}\)
Sign: $-0.262 + 0.964i$
Analytic conductor: \(0.543481\)
Root analytic conductor: \(0.737212\)
Motivic weight: \(0\)
Rational: no
Arithmetic: yes
Character: $\chi_{1089} (967, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 1089,\ (\ :0),\ -0.262 + 0.964i)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(2.103875657\)
\(L(\frac12)\) \(\approx\) \(2.103875657\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad3 \( 1 + (-0.5 + 0.866i)T \)
11 \( 1 \)
good2 \( 1 + (-1.22 + 0.707i)T + (0.5 - 0.866i)T^{2} \)
5 \( 1 + (-0.5 + 0.866i)T + (-0.5 - 0.866i)T^{2} \)
7 \( 1 + (1.22 - 0.707i)T + (0.5 - 0.866i)T^{2} \)
13 \( 1 + (0.5 + 0.866i)T^{2} \)
17 \( 1 - T^{2} \)
19 \( 1 - T^{2} \)
23 \( 1 + (-0.5 - 0.866i)T^{2} \)
29 \( 1 + (-1.22 + 0.707i)T + (0.5 - 0.866i)T^{2} \)
31 \( 1 + (0.5 - 0.866i)T + (-0.5 - 0.866i)T^{2} \)
37 \( 1 + T + T^{2} \)
41 \( 1 + (0.5 + 0.866i)T^{2} \)
43 \( 1 + (0.5 - 0.866i)T^{2} \)
47 \( 1 + (-0.5 - 0.866i)T + (-0.5 + 0.866i)T^{2} \)
53 \( 1 - T + T^{2} \)
59 \( 1 + (0.5 - 0.866i)T + (-0.5 - 0.866i)T^{2} \)
61 \( 1 + (1.22 - 0.707i)T + (0.5 - 0.866i)T^{2} \)
67 \( 1 + (-0.5 + 0.866i)T + (-0.5 - 0.866i)T^{2} \)
71 \( 1 + T + T^{2} \)
73 \( 1 + 1.41iT - T^{2} \)
79 \( 1 + (0.5 - 0.866i)T^{2} \)
83 \( 1 + (1.22 - 0.707i)T + (0.5 - 0.866i)T^{2} \)
89 \( 1 + T^{2} \)
97 \( 1 + (0.5 + 0.866i)T + (-0.5 + 0.866i)T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

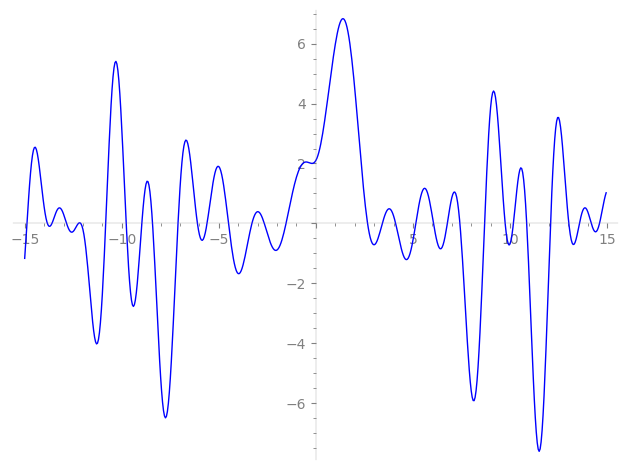
Imaginary part of the first few zeros on the critical line

−9.770163954859646592040401083465, −8.966222470897175577346281033624, −8.415217593764334968797809938997, −7.10297103897251148556946399910, −6.10160206583250533886459593606, −5.60857862348732504522626856422, −4.50045355799031918187645100809, −3.29622586802518816694758198894, −2.65769916299205601557110762508, −1.53911232748449003147121250377, 2.66182498676302749541908147473, 3.42175591788946648275141168233, 4.10667743345344212108250949011, 5.14571203954136845081634654854, 6.05841395278505951749810063999, 6.76905103510706204498182617591, 7.41655155789646222772413484922, 8.688234422047237810482274753567, 9.748844464190782596013385895739, 10.17277726283853219649859807951

Graph of the $Z$-function along the critical line