↑

Properties

Label 2-350-5.4-c7-0-35
Degree 22
Conductor 350350
Sign 0.447−0.894i0.447 - 0.894i
Analytic cond. 109.334109.334
Root an. cond. 10.456310.4563
Motivic weight 77
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + 8i·2-s + 49.3i·3-s − 64·4-s − 394.·6-s + 343i·7-s − 512i·8-s − 243.·9-s − 3.77e3·11-s − 3.15e3i·12-s − 6.86e3i·13-s − 2.74e3·14-s + 4.09e3·16-s + 1.52e4i·17-s − 1.94e3i·18-s − 2.10e4·19-s + ⋯
L(s)  = 1  + 0.707i·2-s + 1.05i·3-s − 0.5·4-s − 0.745·6-s + 0.377i·7-s − 0.353i·8-s − 0.111·9-s − 0.856·11-s − 0.527i·12-s − 0.866i·13-s − 0.267·14-s + 0.250·16-s + 0.753i·17-s − 0.0787i·18-s − 0.702·19-s + ⋯

Functional equation

Λ(s)=(350s/2 ΓC(s) L(s)=((0.447−0.894i) Λ‾(8−s)\begin{aligned}\Lambda(s)=\mathstrut & 350 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.447 - 0.894i)\, \overline{\Lambda}(8-s) \end{aligned}
Λ(s)=(350s/2 ΓC(s+7/2) L(s)=((0.447−0.894i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 350 ^{s/2} \, \Gamma_{\C}(s+7/2) \, L(s)\cr =\mathstrut & (0.447 - 0.894i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 350350    =    2⋅52⋅72 \cdot 5^{2} \cdot 7
Sign: 0.447−0.894i0.447 - 0.894i
Analytic conductor: 109.334109.334
Root analytic conductor: 10.456310.4563
Motivic weight: 77
Rational: no
Arithmetic: yes
Character: χ350(99,⋅)\chi_{350} (99, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 350, ( :7/2), 0.447−0.894i)(2,\ 350,\ (\ :7/2),\ 0.447 - 0.894i)

Particular Values

L(4)L(4) ≈\approx 1.5675000541.567500054
L(12)L(\frac12) ≈\approx 1.5675000541.567500054
L(92)L(\frac{9}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1−8iT 1 - 8iT
5 1 1
7 1−343iT 1 - 343iT
good3 1−49.3iT−2.18e3T2 1 - 49.3iT - 2.18e3T^{2}
11 1+3.77e3T+1.94e7T2 1 + 3.77e3T + 1.94e7T^{2}
13 1+6.86e3iT−6.27e7T2 1 + 6.86e3iT - 6.27e7T^{2}
17 1−1.52e4iT−4.10e8T2 1 - 1.52e4iT - 4.10e8T^{2}
19 1+2.10e4T+8.93e8T2 1 + 2.10e4T + 8.93e8T^{2}
23 1+9.24e4iT−3.40e9T2 1 + 9.24e4iT - 3.40e9T^{2}
29 1+7.86e4T+1.72e10T2 1 + 7.86e4T + 1.72e10T^{2}
31 1−1.52e5T+2.75e10T2 1 - 1.52e5T + 2.75e10T^{2}
37 1+4.45e5iT−9.49e10T2 1 + 4.45e5iT - 9.49e10T^{2}
41 1−3.84e5T+1.94e11T2 1 - 3.84e5T + 1.94e11T^{2}
43 1−2.64e5iT−2.71e11T2 1 - 2.64e5iT - 2.71e11T^{2}
47 1+2.25e5iT−5.06e11T2 1 + 2.25e5iT - 5.06e11T^{2}
53 1+2.63e5iT−1.17e12T2 1 + 2.63e5iT - 1.17e12T^{2}
59 1+9.43e5T+2.48e12T2 1 + 9.43e5T + 2.48e12T^{2}
61 1−2.40e6T+3.14e12T2 1 - 2.40e6T + 3.14e12T^{2}
67 1+4.18e6iT−6.06e12T2 1 + 4.18e6iT - 6.06e12T^{2}
71 1−5.10e6T+9.09e12T2 1 - 5.10e6T + 9.09e12T^{2}
73 1−3.16e6iT−1.10e13T2 1 - 3.16e6iT - 1.10e13T^{2}
79 1−5.00e6T+1.92e13T2 1 - 5.00e6T + 1.92e13T^{2}
83 1+5.49e5iT−2.71e13T2 1 + 5.49e5iT - 2.71e13T^{2}
89 1−3.34e6T+4.42e13T2 1 - 3.34e6T + 4.42e13T^{2}
97 1−1.54e6iT−8.07e13T2 1 - 1.54e6iT - 8.07e13T^{2}
show more
show less
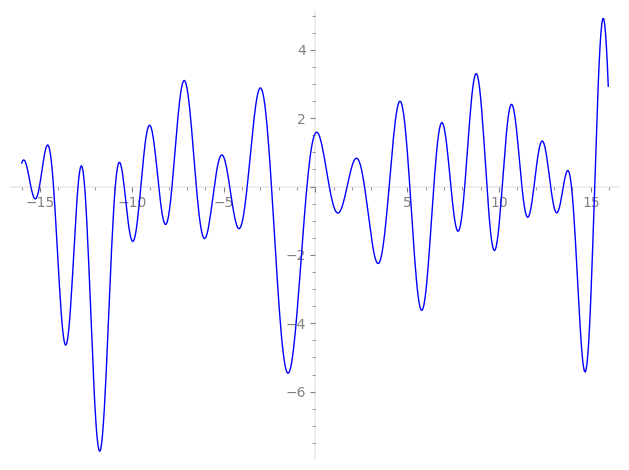
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.42010739906097248348726043709, −9.513059004794196542177872892550, −8.534589046782032329551650776592, −7.81405066536903758055418708164, −6.48850210883995776802624341478, −5.51079409022242760297772670868, −4.67191753970485882366629250370, −3.71880819934961154682809656266, −2.39496923066067519558058989281, −0.46487262549327110866363308595, 0.75331753309352303785891048237, 1.72510030869690200513554946762, 2.67197266616893315015538703294, 4.01341486589629185275671842654, 5.13901202851118832812273705421, 6.44601968186503434112930024299, 7.37848734233560318917213515344, 8.129567912294613114275924385527, 9.341257085204859170348690606474, 10.17223243065254885148484548966

Graph of the ZZ-function along the critical line