↑

Properties

Label 2-3549-273.116-c0-0-6
Degree $2$
Conductor $3549$
Sign $-0.301 + 0.953i$
Analytic cond. $1.77118$
Root an. cond. $1.33085$
Motivic weight $0$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.5 − 0.866i)3-s + (0.5 − 0.866i)4-s + 7-s + (−0.499 − 0.866i)9-s + (−0.499 − 0.866i)12-s + (−0.499 − 0.866i)16-s + (−1.5 + 0.866i)19-s + (0.5 − 0.866i)21-s + (0.5 − 0.866i)25-s − 0.999·27-s + (0.5 − 0.866i)28-s − 0.999·36-s + (1.5 − 0.866i)37-s − 43-s − 0.999·48-s + 49-s + ⋯
L(s)  = 1  + (0.5 − 0.866i)3-s + (0.5 − 0.866i)4-s + 7-s + (−0.499 − 0.866i)9-s + (−0.499 − 0.866i)12-s + (−0.499 − 0.866i)16-s + (−1.5 + 0.866i)19-s + (0.5 − 0.866i)21-s + (0.5 − 0.866i)25-s − 0.999·27-s + (0.5 − 0.866i)28-s − 0.999·36-s + (1.5 − 0.866i)37-s − 43-s − 0.999·48-s + 49-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 3549 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.301 + 0.953i)\, \overline{\Lambda}(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 3549 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.301 + 0.953i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(3549\)    =    \(3 \cdot 7 \cdot 13^{2}\)
Sign: $-0.301 + 0.953i$
Analytic conductor: \(1.77118\)
Root analytic conductor: \(1.33085\)
Motivic weight: \(0\)
Rational: no
Arithmetic: yes
Character: $\chi_{3549} (2027, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 3549,\ (\ :0),\ -0.301 + 0.953i)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(1.787713112\)
\(L(\frac12)\) \(\approx\) \(1.787713112\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad3 \( 1 + (-0.5 + 0.866i)T \)
7 \( 1 - T \)
13 \( 1 \)
good2 \( 1 + (-0.5 + 0.866i)T^{2} \)
5 \( 1 + (-0.5 + 0.866i)T^{2} \)
11 \( 1 + (-0.5 - 0.866i)T^{2} \)
17 \( 1 + (0.5 + 0.866i)T^{2} \)
19 \( 1 + (1.5 - 0.866i)T + (0.5 - 0.866i)T^{2} \)
23 \( 1 + (0.5 - 0.866i)T^{2} \)
29 \( 1 - T^{2} \)
31 \( 1 + (0.5 + 0.866i)T^{2} \)
37 \( 1 + (-1.5 + 0.866i)T + (0.5 - 0.866i)T^{2} \)
41 \( 1 + T^{2} \)
43 \( 1 + T + T^{2} \)
47 \( 1 + (-0.5 + 0.866i)T^{2} \)
53 \( 1 + (0.5 + 0.866i)T^{2} \)
59 \( 1 + (-0.5 - 0.866i)T^{2} \)
61 \( 1 + (0.5 + 0.866i)T + (-0.5 + 0.866i)T^{2} \)
67 \( 1 + (0.5 + 0.866i)T^{2} \)
71 \( 1 + T^{2} \)
73 \( 1 + (-1.5 - 0.866i)T + (0.5 + 0.866i)T^{2} \)
79 \( 1 + (-1 - 1.73i)T + (-0.5 + 0.866i)T^{2} \)
83 \( 1 + T^{2} \)
89 \( 1 + (-0.5 + 0.866i)T^{2} \)
97 \( 1 - 1.73iT - T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

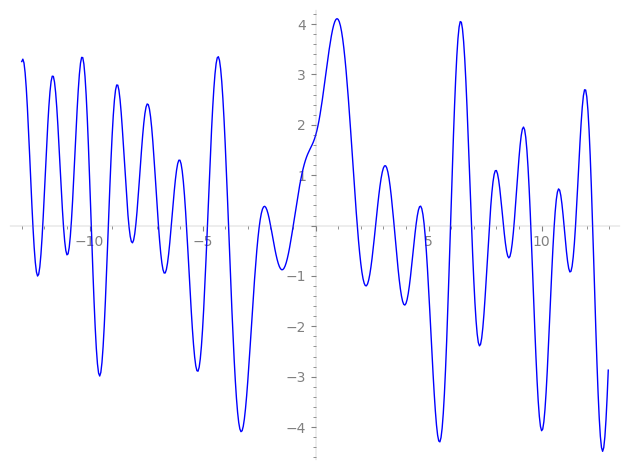
Imaginary part of the first few zeros on the critical line

−8.258345313121635897283444974163, −7.956596336802892185859258476163, −6.95075417051349831004792024554, −6.38289178660233816174991139505, −5.71184273714645165269111202039, −4.78435313950115788866885312619, −3.85003789344574746861109999485, −2.48642925924334335410721501670, −1.99002971842997088432551101453, −0.988385079620251236830817418952, 1.84470622892116988754578254410, 2.65549216054901037730773800983, 3.47547224503991014085091806831, 4.44098704077502661031754280930, 4.80937070239286446947957214667, 5.97051657210641814828685143893, 6.89935756452107476950234747110, 7.69223739874119623098489933960, 8.313887837299748150858049095623, 8.777046709203537479112421379895

Graph of the $Z$-function along the critical line