↑

Properties

Label 2-3960-1320.1139-c0-0-6
Degree $2$
Conductor $3960$
Sign $0.906 + 0.422i$
Analytic cond. $1.97629$
Root an. cond. $1.40580$
Motivic weight $0$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.309 + 0.951i)2-s + (−0.809 + 0.587i)4-s + (−0.951 − 0.309i)5-s + (−0.183 − 0.253i)7-s + (−0.809 − 0.587i)8-s − 0.999i·10-s + (0.987 + 0.156i)11-s + (−1.69 + 0.550i)13-s + (0.183 − 0.253i)14-s + (0.309 − 0.951i)16-s + (−0.363 + 0.5i)19-s + (0.951 − 0.309i)20-s + (0.156 + 0.987i)22-s − 1.61i·23-s + (0.809 + 0.587i)25-s + (−1.04 − 1.44i)26-s + ⋯
L(s)  = 1  + (0.309 + 0.951i)2-s + (−0.809 + 0.587i)4-s + (−0.951 − 0.309i)5-s + (−0.183 − 0.253i)7-s + (−0.809 − 0.587i)8-s − 0.999i·10-s + (0.987 + 0.156i)11-s + (−1.69 + 0.550i)13-s + (0.183 − 0.253i)14-s + (0.309 − 0.951i)16-s + (−0.363 + 0.5i)19-s + (0.951 − 0.309i)20-s + (0.156 + 0.987i)22-s − 1.61i·23-s + (0.809 + 0.587i)25-s + (−1.04 − 1.44i)26-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 3960 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.906 + 0.422i)\, \overline{\Lambda}(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 3960 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.906 + 0.422i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(3960\)    =    \(2^{3} \cdot 3^{2} \cdot 5 \cdot 11\)
Sign: $0.906 + 0.422i$
Analytic conductor: \(1.97629\)
Root analytic conductor: \(1.40580\)
Motivic weight: \(0\)
Rational: no
Arithmetic: yes
Character: $\chi_{3960} (3779, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 3960,\ (\ :0),\ 0.906 + 0.422i)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(0.6912506036\)
\(L(\frac12)\) \(\approx\) \(0.6912506036\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad2 \( 1 + (-0.309 - 0.951i)T \)
3 \( 1 \)
5 \( 1 + (0.951 + 0.309i)T \)
11 \( 1 + (-0.987 - 0.156i)T \)
good7 \( 1 + (0.183 + 0.253i)T + (-0.309 + 0.951i)T^{2} \)
13 \( 1 + (1.69 - 0.550i)T + (0.809 - 0.587i)T^{2} \)
17 \( 1 + (0.809 + 0.587i)T^{2} \)
19 \( 1 + (0.363 - 0.5i)T + (-0.309 - 0.951i)T^{2} \)
23 \( 1 + 1.61iT - T^{2} \)
29 \( 1 + (-0.309 + 0.951i)T^{2} \)
31 \( 1 + (0.809 - 0.587i)T^{2} \)
37 \( 1 + (-0.734 + 0.533i)T + (0.309 - 0.951i)T^{2} \)
41 \( 1 + (1.59 + 1.16i)T + (0.309 + 0.951i)T^{2} \)
43 \( 1 + T^{2} \)
47 \( 1 + (-1.11 + 1.53i)T + (-0.309 - 0.951i)T^{2} \)
53 \( 1 + (-1.11 + 0.363i)T + (0.809 - 0.587i)T^{2} \)
59 \( 1 + (0.533 + 0.734i)T + (-0.309 + 0.951i)T^{2} \)
61 \( 1 + (-0.809 - 0.587i)T^{2} \)
67 \( 1 - T^{2} \)
71 \( 1 + (-0.809 - 0.587i)T^{2} \)
73 \( 1 + (0.309 - 0.951i)T^{2} \)
79 \( 1 + (-0.809 + 0.587i)T^{2} \)
83 \( 1 + (0.809 + 0.587i)T^{2} \)
89 \( 1 + 1.97iT - T^{2} \)
97 \( 1 + (0.809 - 0.587i)T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

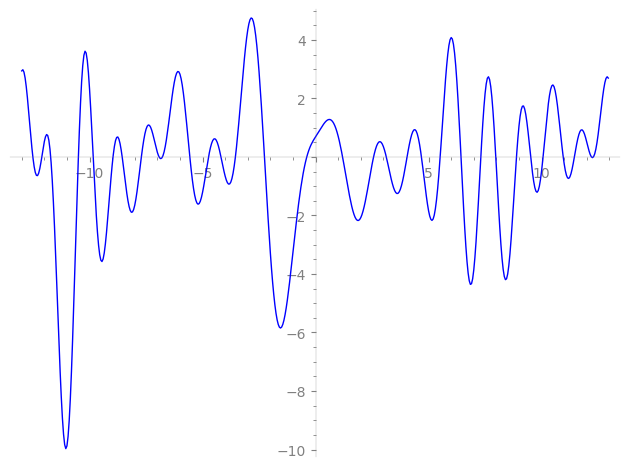
Imaginary part of the first few zeros on the critical line

−8.555221430967833891226206552787, −7.70744705493643018121631340916, −6.92256955615487964255563942485, −6.77496352184355833491013535459, −5.56200474417411809437939569882, −4.73523336662415282796589926153, −4.18463209746546954438641750333, −3.54716606456850685437782663586, −2.26106392409611313642146388499, −0.38944782631777374307839999932, 1.19461142653265045354560645438, 2.56653201128035107726089009610, 3.12969268690269905203591080046, 4.04786849237894158086851065134, 4.68412916565345552088149929481, 5.51256337533275786362725426817, 6.43494707204278847466191842170, 7.31147094890603645384477614367, 7.974098848952819058176474405445, 8.888808749503127841960944785187

Graph of the $Z$-function along the critical line