↑

Properties

Label 2-546-13.4-c1-0-2
Degree 22
Conductor 546546
Sign 0.824−0.565i0.824 - 0.565i
Analytic cond. 4.359834.35983
Root an. cond. 2.088022.08802
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−0.866 − 0.5i)2-s + (−0.5 + 0.866i)3-s + (0.499 + 0.866i)4-s + 0.732i·5-s + (0.866 − 0.499i)6-s + (0.866 − 0.5i)7-s − 0.999i·8-s + (−0.499 − 0.866i)9-s + (0.366 − 0.633i)10-s + (1.5 + 0.866i)11-s − 0.999·12-s + (1.59 − 3.23i)13-s − 0.999·14-s + (−0.633 − 0.366i)15-s + (−0.5 + 0.866i)16-s + (1.86 + 3.23i)17-s + ⋯
L(s)  = 1  + (−0.612 − 0.353i)2-s + (−0.288 + 0.499i)3-s + (0.249 + 0.433i)4-s + 0.327i·5-s + (0.353 − 0.204i)6-s + (0.327 − 0.188i)7-s − 0.353i·8-s + (−0.166 − 0.288i)9-s + (0.115 − 0.200i)10-s + (0.452 + 0.261i)11-s − 0.288·12-s + (0.443 − 0.896i)13-s − 0.267·14-s + (−0.163 − 0.0945i)15-s + (−0.125 + 0.216i)16-s + (0.452 + 0.783i)17-s + ⋯

Functional equation

Λ(s)=(546s/2 ΓC(s) L(s)=((0.824−0.565i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 546 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.824 - 0.565i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(546s/2 ΓC(s+1/2) L(s)=((0.824−0.565i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 546 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.824 - 0.565i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 546546    =    2⋅3⋅7⋅132 \cdot 3 \cdot 7 \cdot 13
Sign: 0.824−0.565i0.824 - 0.565i
Analytic conductor: 4.359834.35983
Root analytic conductor: 2.088022.08802
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ546(43,⋅)\chi_{546} (43, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 546, ( :1/2), 0.824−0.565i)(2,\ 546,\ (\ :1/2),\ 0.824 - 0.565i)

Particular Values

L(1)L(1) ≈\approx 0.990612+0.306880i0.990612 + 0.306880i
L(12)L(\frac12) ≈\approx 0.990612+0.306880i0.990612 + 0.306880i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1+(0.866+0.5i)T 1 + (0.866 + 0.5i)T
3 1+(0.5−0.866i)T 1 + (0.5 - 0.866i)T
7 1+(−0.866+0.5i)T 1 + (-0.866 + 0.5i)T
13 1+(−1.59+3.23i)T 1 + (-1.59 + 3.23i)T
good5 1−0.732iT−5T2 1 - 0.732iT - 5T^{2}
11 1+(−1.5−0.866i)T+(5.5+9.52i)T2 1 + (-1.5 - 0.866i)T + (5.5 + 9.52i)T^{2}
17 1+(−1.86−3.23i)T+(−8.5+14.7i)T2 1 + (-1.86 - 3.23i)T + (-8.5 + 14.7i)T^{2}
19 1+(−0.866+0.5i)T+(9.5−16.4i)T2 1 + (-0.866 + 0.5i)T + (9.5 - 16.4i)T^{2}
23 1+(1.73−3i)T+(−11.5−19.9i)T2 1 + (1.73 - 3i)T + (-11.5 - 19.9i)T^{2}
29 1+(3.23−5.59i)T+(−14.5−25.1i)T2 1 + (3.23 - 5.59i)T + (-14.5 - 25.1i)T^{2}
31 1−2.19iT−31T2 1 - 2.19iT - 31T^{2}
37 1+(−5.83−3.36i)T+(18.5+32.0i)T2 1 + (-5.83 - 3.36i)T + (18.5 + 32.0i)T^{2}
41 1+(−2.59−1.5i)T+(20.5+35.5i)T2 1 + (-2.59 - 1.5i)T + (20.5 + 35.5i)T^{2}
43 1+(−1.63−2.83i)T+(−21.5+37.2i)T2 1 + (-1.63 - 2.83i)T + (-21.5 + 37.2i)T^{2}
47 1−2.46iT−47T2 1 - 2.46iT - 47T^{2}
53 1−7T+53T2 1 - 7T + 53T^{2}
59 1+(−0.803+0.464i)T+(29.5−51.0i)T2 1 + (-0.803 + 0.464i)T + (29.5 - 51.0i)T^{2}
61 1+(2.59+4.5i)T+(−30.5+52.8i)T2 1 + (2.59 + 4.5i)T + (-30.5 + 52.8i)T^{2}
67 1+(−7.73−4.46i)T+(33.5+58.0i)T2 1 + (-7.73 - 4.46i)T + (33.5 + 58.0i)T^{2}
71 1+(1.90−1.09i)T+(35.5−61.4i)T2 1 + (1.90 - 1.09i)T + (35.5 - 61.4i)T^{2}
73 1+5.46iT−73T2 1 + 5.46iT - 73T^{2}
79 1−2.07T+79T2 1 - 2.07T + 79T^{2}
83 1−0.196iT−83T2 1 - 0.196iT - 83T^{2}
89 1+(9.06+5.23i)T+(44.5+77.0i)T2 1 + (9.06 + 5.23i)T + (44.5 + 77.0i)T^{2}
97 1+(−13.5+7.83i)T+(48.5−84.0i)T2 1 + (-13.5 + 7.83i)T + (48.5 - 84.0i)T^{2}
show more
show less
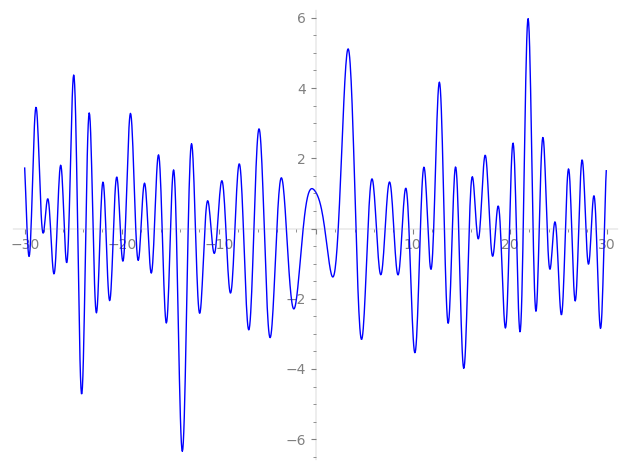
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.79138033454868803260646687652, −10.14101182143248310448590899383, −9.265719529422004260797475720291, −8.328420958027490393525284725526, −7.45635512146812402965429340057, −6.37243157379974201728589590801, −5.30416635511018551316427233145, −4.00716912079894669915291008645, −3.02685845403989968944866945151, −1.29360346199130214710424497254, 0.926301273700834640319346484287, 2.32096330944653758915800400891, 4.14794305922382447816013905362, 5.38567503252472026963338892338, 6.25984439166830131523684117458, 7.15873600195455352732704127888, 8.049184739844742437751944333469, 8.910811064175830418972629874358, 9.607515564867724926863919977306, 10.79085251521530519987053866857

Graph of the ZZ-function along the critical line