↑

Properties

Label 2-637-7.4-c1-0-32
Degree 22
Conductor 637637
Sign 0.605+0.795i0.605 + 0.795i
Analytic cond. 5.086475.08647
Root an. cond. 2.255322.25532
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.605 + 1.04i)2-s + (0.872 − 1.51i)3-s + (0.267 − 0.462i)4-s + (−1.10 − 1.91i)5-s + 2.11·6-s + 3.06·8-s + (−0.0222 − 0.0384i)9-s + (1.33 − 2.31i)10-s + (0.394 − 0.683i)11-s + (−0.465 − 0.807i)12-s − 13-s − 3.85·15-s + (1.32 + 2.29i)16-s + (0.872 − 1.51i)17-s + (0.0268 − 0.0465i)18-s + (−2.16 − 3.74i)19-s + ⋯
L(s)  = 1  + (0.428 + 0.741i)2-s + (0.503 − 0.872i)3-s + (0.133 − 0.231i)4-s + (−0.494 − 0.856i)5-s + 0.862·6-s + 1.08·8-s + (−0.00740 − 0.0128i)9-s + (0.423 − 0.733i)10-s + (0.118 − 0.206i)11-s + (−0.134 − 0.232i)12-s − 0.277·13-s − 0.995·15-s + (0.330 + 0.573i)16-s + (0.211 − 0.366i)17-s + (0.00633 − 0.0109i)18-s + (−0.495 − 0.858i)19-s + ⋯

Functional equation

Λ(s)=(637s/2 ΓC(s) L(s)=((0.605+0.795i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 637 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.605 + 0.795i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(637s/2 ΓC(s+1/2) L(s)=((0.605+0.795i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 637 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.605 + 0.795i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 637637    =    72⋅137^{2} \cdot 13
Sign: 0.605+0.795i0.605 + 0.795i
Analytic conductor: 5.086475.08647
Root analytic conductor: 2.255322.25532
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ637(508,⋅)\chi_{637} (508, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 637, ( :1/2), 0.605+0.795i)(2,\ 637,\ (\ :1/2),\ 0.605 + 0.795i)

Particular Values

L(1)L(1) ≈\approx 2.00242−0.992657i2.00242 - 0.992657i
L(12)L(\frac12) ≈\approx 2.00242−0.992657i2.00242 - 0.992657i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad7 1 1
13 1+T 1 + T
good2 1+(−0.605−1.04i)T+(−1+1.73i)T2 1 + (-0.605 - 1.04i)T + (-1 + 1.73i)T^{2}
3 1+(−0.872+1.51i)T+(−1.5−2.59i)T2 1 + (-0.872 + 1.51i)T + (-1.5 - 2.59i)T^{2}
5 1+(1.10+1.91i)T+(−2.5+4.33i)T2 1 + (1.10 + 1.91i)T + (-2.5 + 4.33i)T^{2}
11 1+(−0.394+0.683i)T+(−5.5−9.52i)T2 1 + (-0.394 + 0.683i)T + (-5.5 - 9.52i)T^{2}
17 1+(−0.872+1.51i)T+(−8.5−14.7i)T2 1 + (-0.872 + 1.51i)T + (-8.5 - 14.7i)T^{2}
19 1+(2.16+3.74i)T+(−9.5+16.4i)T2 1 + (2.16 + 3.74i)T + (-9.5 + 16.4i)T^{2}
23 1+(−0.556−0.963i)T+(−11.5+19.9i)T2 1 + (-0.556 - 0.963i)T + (-11.5 + 19.9i)T^{2}
29 1+8.48T+29T2 1 + 8.48T + 29T^{2}
31 1+(2.85−4.93i)T+(−15.5−26.8i)T2 1 + (2.85 - 4.93i)T + (-15.5 - 26.8i)T^{2}
37 1+(1.13+1.97i)T+(−18.5+32.0i)T2 1 + (1.13 + 1.97i)T + (-18.5 + 32.0i)T^{2}
41 1−12.1T+41T2 1 - 12.1T + 41T^{2}
43 1−8.06T+43T2 1 - 8.06T + 43T^{2}
47 1+(4.37+7.57i)T+(−23.5+40.7i)T2 1 + (4.37 + 7.57i)T + (-23.5 + 40.7i)T^{2}
53 1+(3.97−6.88i)T+(−26.5−45.8i)T2 1 + (3.97 - 6.88i)T + (-26.5 - 45.8i)T^{2}
59 1+(5.47−9.48i)T+(−29.5−51.0i)T2 1 + (5.47 - 9.48i)T + (-29.5 - 51.0i)T^{2}
61 1+(−6.53−11.3i)T+(−30.5+52.8i)T2 1 + (-6.53 - 11.3i)T + (-30.5 + 52.8i)T^{2}
67 1+(3.27−5.67i)T+(−33.5−58.0i)T2 1 + (3.27 - 5.67i)T + (-33.5 - 58.0i)T^{2}
71 1−5.85T+71T2 1 - 5.85T + 71T^{2}
73 1+(4.00−6.93i)T+(−36.5−63.2i)T2 1 + (4.00 - 6.93i)T + (-36.5 - 63.2i)T^{2}
79 1+(−3.45−5.98i)T+(−39.5+68.4i)T2 1 + (-3.45 - 5.98i)T + (-39.5 + 68.4i)T^{2}
83 1−3.14T+83T2 1 - 3.14T + 83T^{2}
89 1+(1.69+2.93i)T+(−44.5+77.0i)T2 1 + (1.69 + 2.93i)T + (-44.5 + 77.0i)T^{2}
97 1+0.0981T+97T2 1 + 0.0981T + 97T^{2}
show more
show less
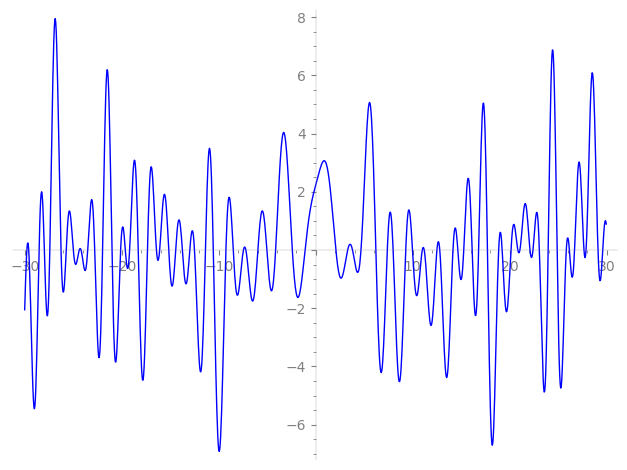
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.55797825945503111318775342222, −9.257586224336709357742995011261, −8.503224478997118369996463048244, −7.45042002162371669303556715424, −7.20128532660423368151301995332, −5.96154487708859454262693760297, −5.03660732250445029573639450357, −4.13365207863155134252920858907, −2.41278615077544224271228918786, −1.09603677917384233727651398137, 2.07830902742602164839761354412, 3.29069334475054533268532651372, 3.79277665833716766049605035926, 4.66594180245265279257420702879, 6.19783866404153235566283056276, 7.37793754287445938114259314969, 7.977954112632355904953663499377, 9.278216530478113267221587200901, 9.975559949260956912075262593962, 10.98665053660586708081036171828

Graph of the ZZ-function along the critical line