↑

Properties

Label 2-855-19.7-c1-0-25
Degree 22
Conductor 855855
Sign −0.0977+0.995i-0.0977 + 0.995i
Analytic cond. 6.827206.82720
Root an. cond. 2.612892.61289
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−0.207 − 0.358i)2-s + (0.914 − 1.58i)4-s + (−0.5 − 0.866i)5-s + 1.82·7-s − 1.58·8-s + (−0.207 + 0.358i)10-s + 2.82·11-s + (0.914 − 1.58i)13-s + (−0.378 − 0.655i)14-s + (−1.49 − 2.59i)16-s + (−0.585 − 1.01i)17-s + (4 + 1.73i)19-s − 1.82·20-s + (−0.585 − 1.01i)22-s + (−0.414 + 0.717i)23-s + ⋯
L(s)  = 1  + (−0.146 − 0.253i)2-s + (0.457 − 0.791i)4-s + (−0.223 − 0.387i)5-s + 0.691·7-s − 0.560·8-s + (−0.0654 + 0.113i)10-s + 0.852·11-s + (0.253 − 0.439i)13-s + (−0.101 − 0.175i)14-s + (−0.374 − 0.649i)16-s + (−0.142 − 0.246i)17-s + (0.917 + 0.397i)19-s − 0.408·20-s + (−0.124 − 0.216i)22-s + (−0.0863 + 0.149i)23-s + ⋯

Functional equation

Λ(s)=(855s/2 ΓC(s) L(s)=((−0.0977+0.995i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 855 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.0977 + 0.995i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(855s/2 ΓC(s+1/2) L(s)=((−0.0977+0.995i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 855 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (-0.0977 + 0.995i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 855855    =    32⋅5⋅193^{2} \cdot 5 \cdot 19
Sign: −0.0977+0.995i-0.0977 + 0.995i
Analytic conductor: 6.827206.82720
Root analytic conductor: 2.612892.61289
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ855(406,⋅)\chi_{855} (406, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 855, ( :1/2), −0.0977+0.995i)(2,\ 855,\ (\ :1/2),\ -0.0977 + 0.995i)

Particular Values

L(1)L(1) ≈\approx 1.11763−1.23277i1.11763 - 1.23277i
L(12)L(\frac12) ≈\approx 1.11763−1.23277i1.11763 - 1.23277i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad3 1 1
5 1+(0.5+0.866i)T 1 + (0.5 + 0.866i)T
19 1+(−4−1.73i)T 1 + (-4 - 1.73i)T
good2 1+(0.207+0.358i)T+(−1+1.73i)T2 1 + (0.207 + 0.358i)T + (-1 + 1.73i)T^{2}
7 1−1.82T+7T2 1 - 1.82T + 7T^{2}
11 1−2.82T+11T2 1 - 2.82T + 11T^{2}
13 1+(−0.914+1.58i)T+(−6.5−11.2i)T2 1 + (-0.914 + 1.58i)T + (-6.5 - 11.2i)T^{2}
17 1+(0.585+1.01i)T+(−8.5+14.7i)T2 1 + (0.585 + 1.01i)T + (-8.5 + 14.7i)T^{2}
23 1+(0.414−0.717i)T+(−11.5−19.9i)T2 1 + (0.414 - 0.717i)T + (-11.5 - 19.9i)T^{2}
29 1+(−4.82+8.36i)T+(−14.5−25.1i)T2 1 + (-4.82 + 8.36i)T + (-14.5 - 25.1i)T^{2}
31 1+5T+31T2 1 + 5T + 31T^{2}
37 1+2.17T+37T2 1 + 2.17T + 37T^{2}
41 1+(−1.41−2.44i)T+(−20.5+35.5i)T2 1 + (-1.41 - 2.44i)T + (-20.5 + 35.5i)T^{2}
43 1+(3.91+6.77i)T+(−21.5+37.2i)T2 1 + (3.91 + 6.77i)T + (-21.5 + 37.2i)T^{2}
47 1+(1.58−2.74i)T+(−23.5−40.7i)T2 1 + (1.58 - 2.74i)T + (-23.5 - 40.7i)T^{2}
53 1+(1−1.73i)T+(−26.5−45.8i)T2 1 + (1 - 1.73i)T + (-26.5 - 45.8i)T^{2}
59 1+(−29.5+51.0i)T2 1 + (-29.5 + 51.0i)T^{2}
61 1+(−4.15+7.19i)T+(−30.5−52.8i)T2 1 + (-4.15 + 7.19i)T + (-30.5 - 52.8i)T^{2}
67 1+(2.74−4.75i)T+(−33.5−58.0i)T2 1 + (2.74 - 4.75i)T + (-33.5 - 58.0i)T^{2}
71 1+(−5−8.66i)T+(−35.5+61.4i)T2 1 + (-5 - 8.66i)T + (-35.5 + 61.4i)T^{2}
73 1+(4.74+8.21i)T+(−36.5+63.2i)T2 1 + (4.74 + 8.21i)T + (-36.5 + 63.2i)T^{2}
79 1+(−1.67−2.89i)T+(−39.5+68.4i)T2 1 + (-1.67 - 2.89i)T + (-39.5 + 68.4i)T^{2}
83 1−8T+83T2 1 - 8T + 83T^{2}
89 1+(−6.24+10.8i)T+(−44.5−77.0i)T2 1 + (-6.24 + 10.8i)T + (-44.5 - 77.0i)T^{2}
97 1+(−3−5.19i)T+(−48.5+84.0i)T2 1 + (-3 - 5.19i)T + (-48.5 + 84.0i)T^{2}
show more
show less
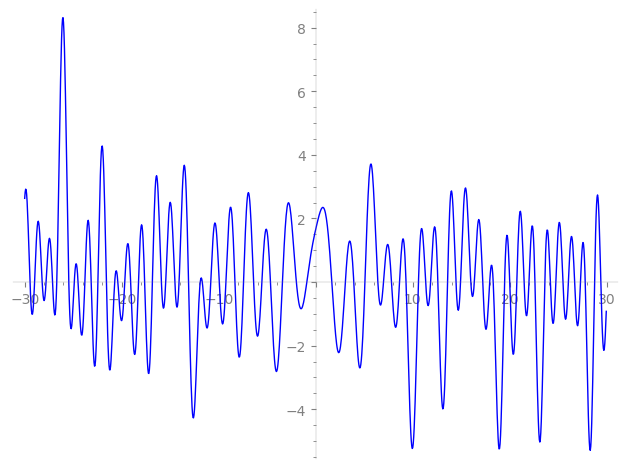
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−9.947722280057485080315481949949, −9.258567081997665989987758629663, −8.326299055699953988930225555920, −7.44159217249037496392029981502, −6.40476831805804803909153137550, −5.55215288811809189456164127551, −4.66718936476540772382927057986, −3.43197614292778025536600580339, −1.99465804068466328322066550493, −0.918218696833690515175329225242, 1.65939686926094256625508042628, 3.05356233117805522530622085727, 3.92471164930328643949208812155, 5.07077938489908334972766734758, 6.40437767461734359444151609195, 6.98640162379484144943588917881, 7.81120901884500628590531389722, 8.639325829248419407696360072546, 9.307887442883313096730002718773, 10.59086765557681449112254109521

Graph of the ZZ-function along the critical line