↑

Properties

Label 2-980-140.39-c0-0-5
Degree 22
Conductor 980980
Sign 0.328+0.944i0.328 + 0.944i
Analytic cond. 0.4890830.489083
Root an. cond. 0.6993450.699345
Motivic weight 00
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.866 − 0.5i)2-s + (0.499 − 0.866i)4-s + (0.258 − 0.965i)5-s − 0.999i·8-s + (0.5 + 0.866i)9-s + (−0.258 − 0.965i)10-s + 1.41i·13-s + (−0.5 − 0.866i)16-s + (−1.22 − 0.707i)17-s + (0.866 + 0.499i)18-s + (−0.707 − 0.707i)20-s + (−0.866 − 0.499i)25-s + (0.707 + 1.22i)26-s + (−0.866 − 0.499i)32-s − 1.41·34-s + ⋯
L(s)  = 1  + (0.866 − 0.5i)2-s + (0.499 − 0.866i)4-s + (0.258 − 0.965i)5-s − 0.999i·8-s + (0.5 + 0.866i)9-s + (−0.258 − 0.965i)10-s + 1.41i·13-s + (−0.5 − 0.866i)16-s + (−1.22 − 0.707i)17-s + (0.866 + 0.499i)18-s + (−0.707 − 0.707i)20-s + (−0.866 − 0.499i)25-s + (0.707 + 1.22i)26-s + (−0.866 − 0.499i)32-s − 1.41·34-s + ⋯

Functional equation

Λ(s)=(980s/2 ΓC(s) L(s)=((0.328+0.944i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 980 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.328 + 0.944i)\, \overline{\Lambda}(1-s) \end{aligned}
Λ(s)=(980s/2 ΓC(s) L(s)=((0.328+0.944i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 980 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.328 + 0.944i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 980980    =    22⋅5⋅722^{2} \cdot 5 \cdot 7^{2}
Sign: 0.328+0.944i0.328 + 0.944i
Analytic conductor: 0.4890830.489083
Root analytic conductor: 0.6993450.699345
Motivic weight: 00
Rational: no
Arithmetic: yes
Character: χ980(459,⋅)\chi_{980} (459, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 980, ( :0), 0.328+0.944i)(2,\ 980,\ (\ :0),\ 0.328 + 0.944i)

Particular Values

L(12)L(\frac{1}{2}) ≈\approx 1.6983061241.698306124
L(12)L(\frac12) ≈\approx 1.6983061241.698306124
L(1)L(1) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1+(−0.866+0.5i)T 1 + (-0.866 + 0.5i)T
5 1+(−0.258+0.965i)T 1 + (-0.258 + 0.965i)T
7 1 1
good3 1+(−0.5−0.866i)T2 1 + (-0.5 - 0.866i)T^{2}
11 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
13 1−1.41iT−T2 1 - 1.41iT - T^{2}
17 1+(1.22+0.707i)T+(0.5+0.866i)T2 1 + (1.22 + 0.707i)T + (0.5 + 0.866i)T^{2}
19 1+(0.5−0.866i)T2 1 + (0.5 - 0.866i)T^{2}
23 1+(−0.5+0.866i)T2 1 + (-0.5 + 0.866i)T^{2}
29 1+T2 1 + T^{2}
31 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
37 1+(1.73−i)T+(0.5−0.866i)T2 1 + (1.73 - i)T + (0.5 - 0.866i)T^{2}
41 1−1.41T+T2 1 - 1.41T + T^{2}
43 1+T2 1 + T^{2}
47 1+(−0.5+0.866i)T2 1 + (-0.5 + 0.866i)T^{2}
53 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
59 1+(0.5+0.866i)T2 1 + (0.5 + 0.866i)T^{2}
61 1+(−0.707−1.22i)T+(−0.5+0.866i)T2 1 + (-0.707 - 1.22i)T + (-0.5 + 0.866i)T^{2}
67 1+(−0.5−0.866i)T2 1 + (-0.5 - 0.866i)T^{2}
71 1−T2 1 - T^{2}
73 1+(−1.22−0.707i)T+(0.5+0.866i)T2 1 + (-1.22 - 0.707i)T + (0.5 + 0.866i)T^{2}
79 1+(0.5−0.866i)T2 1 + (0.5 - 0.866i)T^{2}
83 1+T2 1 + T^{2}
89 1+(0.707+1.22i)T+(−0.5+0.866i)T2 1 + (0.707 + 1.22i)T + (-0.5 + 0.866i)T^{2}
97 1+1.41iT−T2 1 + 1.41iT - T^{2}
show more
show less
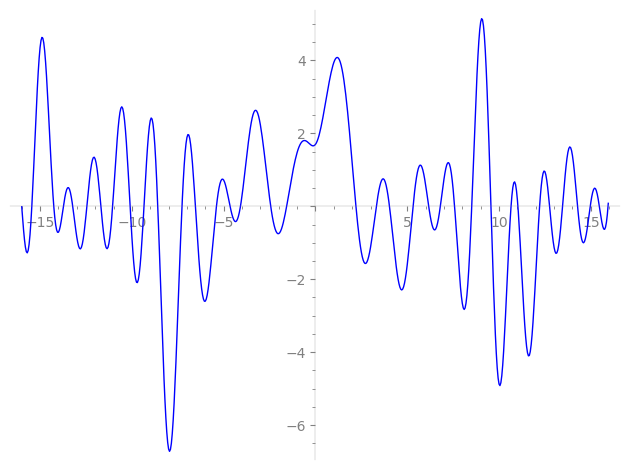
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.09668256403529590553036571258, −9.339961940217215710522070140503, −8.590576557424563564510420489027, −7.26936529084338686246055238888, −6.53997326886289478204797337404, −5.39359275853674283116118357473, −4.65137667267739863832428856911, −4.08533714243369585529571767341, −2.43702674696240688646207824865, −1.56997585376123356412284055248, 2.20521203852142335097996614980, 3.31666910974646657766852397326, 4.03137258369416807580917582221, 5.31501265359363504375946607783, 6.16827925347434225808974143010, 6.80238051486425567189278622551, 7.57719904095778612843745925885, 8.512280805834303309378364583846, 9.557920937791690140886301999819, 10.65429995339887270788488095082

Graph of the ZZ-function along the critical line