↑

Properties

Label 2-240-240.149-c0-0-0
Degree $2$
Conductor $240$
Sign $0.923 + 0.382i$
Analytic cond. $0.119775$
Root an. cond. $0.346086$
Motivic weight $0$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.707 − 0.707i)2-s + (−0.707 + 0.707i)3-s − 1.00i·4-s + (0.707 + 0.707i)5-s + 1.00i·6-s + (−0.707 − 0.707i)8-s − 1.00i·9-s + 1.00·10-s + (0.707 + 0.707i)12-s − 1.00·15-s − 1.00·16-s − 1.41·17-s + (−0.707 − 0.707i)18-s + (−1 + i)19-s + (0.707 − 0.707i)20-s + ⋯
L(s)  = 1  + (0.707 − 0.707i)2-s + (−0.707 + 0.707i)3-s − 1.00i·4-s + (0.707 + 0.707i)5-s + 1.00i·6-s + (−0.707 − 0.707i)8-s − 1.00i·9-s + 1.00·10-s + (0.707 + 0.707i)12-s − 1.00·15-s − 1.00·16-s − 1.41·17-s + (−0.707 − 0.707i)18-s + (−1 + i)19-s + (0.707 − 0.707i)20-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 240 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.923 + 0.382i)\, \overline{\Lambda}(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 240 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.923 + 0.382i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(240\)    =    \(2^{4} \cdot 3 \cdot 5\)
Sign: $0.923 + 0.382i$
Analytic conductor: \(0.119775\)
Root analytic conductor: \(0.346086\)
Motivic weight: \(0\)
Rational: no
Arithmetic: yes
Character: $\chi_{240} (149, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 240,\ (\ :0),\ 0.923 + 0.382i)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(0.8766671503\)
\(L(\frac12)\) \(\approx\) \(0.8766671503\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad2 \( 1 + (-0.707 + 0.707i)T \)
3 \( 1 + (0.707 - 0.707i)T \)
5 \( 1 + (-0.707 - 0.707i)T \)
good7 \( 1 + T^{2} \)
11 \( 1 - iT^{2} \)
13 \( 1 + iT^{2} \)
17 \( 1 + 1.41T + T^{2} \)
19 \( 1 + (1 - i)T - iT^{2} \)
23 \( 1 + 1.41iT - T^{2} \)
29 \( 1 + iT^{2} \)
31 \( 1 + T^{2} \)
37 \( 1 - iT^{2} \)
41 \( 1 + T^{2} \)
43 \( 1 - iT^{2} \)
47 \( 1 - 1.41T + T^{2} \)
53 \( 1 + iT^{2} \)
59 \( 1 - iT^{2} \)
61 \( 1 + (-1 + i)T - iT^{2} \)
67 \( 1 + iT^{2} \)
71 \( 1 + T^{2} \)
73 \( 1 + T^{2} \)
79 \( 1 - 2T + T^{2} \)
83 \( 1 - iT^{2} \)
89 \( 1 + T^{2} \)
97 \( 1 - T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

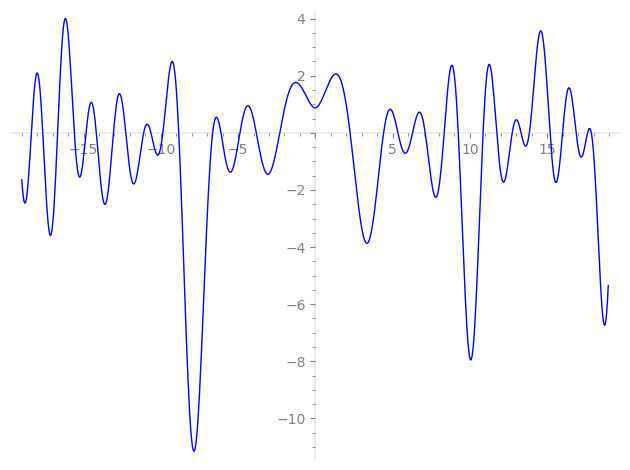
Imaginary part of the first few zeros on the critical line

−12.26721417849261293596645460060, −11.11131220770607798226918165643, −10.64097464556387682232035748743, −9.869253903909641976622981782502, −8.841082183980231248155593852572, −6.64680116357307140519216654282, −6.10824185492806981195453492813, −4.88125479089220107901179134265, −3.82307167587796902320542056164, −2.30617234132287283414117722721, 2.24575443364944395837814610849, 4.41427234721809658050812687514, 5.34247706028749470396480426010, 6.28632739387403651003711591542, 7.10643469094892591735622019280, 8.332017620077728990333548048250, 9.243675452755684177654724180201, 10.84841673103753683404825725208, 11.73527978311156713498965175251, 12.73502049729843391068627570637

Graph of the $Z$-function along the critical line