↑

Properties

Label 2-2420-11.10-c2-0-5
Degree 22
Conductor 24202420
Sign −0.975−0.219i-0.975 - 0.219i
Analytic cond. 65.940265.9402
Root an. cond. 8.120358.12035
Motivic weight 22
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + 2.84·3-s + 2.23·5-s + 3.16i·7-s − 0.887·9-s + 1.15i·13-s + 6.36·15-s + 17.5i·17-s + 20.0i·19-s + 9.01i·21-s − 36.9·23-s + 5.00·25-s − 28.1·27-s − 5.00i·29-s − 61.1·31-s + 7.07i·35-s + ⋯
L(s)  = 1  + 0.949·3-s + 0.447·5-s + 0.452i·7-s − 0.0986·9-s + 0.0885i·13-s + 0.424·15-s + 1.03i·17-s + 1.05i·19-s + 0.429i·21-s − 1.60·23-s + 0.200·25-s − 1.04·27-s − 0.172i·29-s − 1.97·31-s + 0.202i·35-s + ⋯

Functional equation

Λ(s)=(2420s/2 ΓC(s) L(s)=((−0.975−0.219i) Λ‾(3−s)\begin{aligned}\Lambda(s)=\mathstrut & 2420 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.975 - 0.219i)\, \overline{\Lambda}(3-s) \end{aligned}
Λ(s)=(2420s/2 ΓC(s+1) L(s)=((−0.975−0.219i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 2420 ^{s/2} \, \Gamma_{\C}(s+1) \, L(s)\cr =\mathstrut & (-0.975 - 0.219i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 24202420    =    22⋅5⋅1122^{2} \cdot 5 \cdot 11^{2}
Sign: −0.975−0.219i-0.975 - 0.219i
Analytic conductor: 65.940265.9402
Root analytic conductor: 8.120358.12035
Motivic weight: 22
Rational: no
Arithmetic: yes
Character: χ2420(241,⋅)\chi_{2420} (241, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 2420, ( :1), −0.975−0.219i)(2,\ 2420,\ (\ :1),\ -0.975 - 0.219i)

Particular Values

L(32)L(\frac{3}{2}) ≈\approx 0.77956579890.7795657989
L(12)L(\frac12) ≈\approx 0.77956579890.7795657989
L(2)L(2) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1 1
5 1−2.23T 1 - 2.23T
11 1 1
good3 1−2.84T+9T2 1 - 2.84T + 9T^{2}
7 1−3.16iT−49T2 1 - 3.16iT - 49T^{2}
13 1−1.15iT−169T2 1 - 1.15iT - 169T^{2}
17 1−17.5iT−289T2 1 - 17.5iT - 289T^{2}
19 1−20.0iT−361T2 1 - 20.0iT - 361T^{2}
23 1+36.9T+529T2 1 + 36.9T + 529T^{2}
29 1+5.00iT−841T2 1 + 5.00iT - 841T^{2}
31 1+61.1T+961T2 1 + 61.1T + 961T^{2}
37 1+26.0T+1.36e3T2 1 + 26.0T + 1.36e3T^{2}
41 1+60.2iT−1.68e3T2 1 + 60.2iT - 1.68e3T^{2}
43 1+65.3iT−1.84e3T2 1 + 65.3iT - 1.84e3T^{2}
47 1+26.6T+2.20e3T2 1 + 26.6T + 2.20e3T^{2}
53 1−3.09T+2.80e3T2 1 - 3.09T + 2.80e3T^{2}
59 1+9.31T+3.48e3T2 1 + 9.31T + 3.48e3T^{2}
61 1+33.9iT−3.72e3T2 1 + 33.9iT - 3.72e3T^{2}
67 1+17.1T+4.48e3T2 1 + 17.1T + 4.48e3T^{2}
71 1−50.7T+5.04e3T2 1 - 50.7T + 5.04e3T^{2}
73 1−41.3iT−5.32e3T2 1 - 41.3iT - 5.32e3T^{2}
79 1−42.2iT−6.24e3T2 1 - 42.2iT - 6.24e3T^{2}
83 1−80.7iT−6.88e3T2 1 - 80.7iT - 6.88e3T^{2}
89 1+109.T+7.92e3T2 1 + 109.T + 7.92e3T^{2}
97 1+20.0T+9.40e3T2 1 + 20.0T + 9.40e3T^{2}
show more
show less
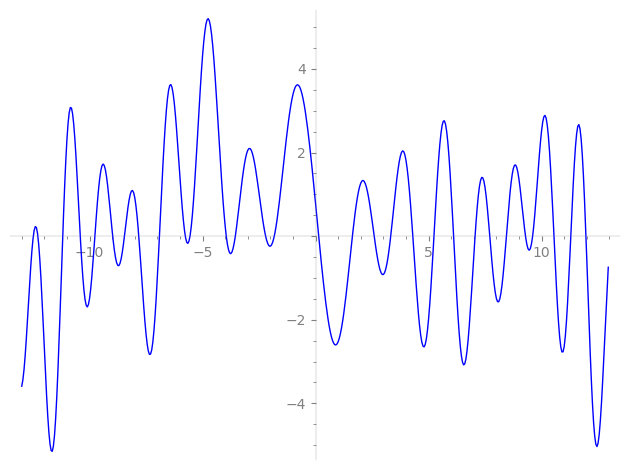
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−8.974571657695429616143013695560, −8.456423904528071816280871864479, −7.82178593848562173108549533592, −6.90816196526916888760088369351, −5.76271632955760923107715439983, −5.55479270422444914462375032901, −3.95060359714113281790156352560, −3.55037832603159004683057080147, −2.20905280310797240779454119822, −1.82739558288042118796951315780, 0.13848682864015244424811847369, 1.62940490322693700738289515821, 2.59435235954579377110927474556, 3.32497853482693905804391656900, 4.30325777054530748782259410704, 5.23760413010839323771538385755, 6.10658922275077174895258421732, 7.05705987651970233140495719258, 7.70805130390452841761376977300, 8.442387184077120257227159217054

Graph of the ZZ-function along the critical line