↑

Properties

Label 2-2646-9.7-c1-0-5
Degree 22
Conductor 26462646
Sign 0.422−0.906i0.422 - 0.906i
Analytic cond. 21.128421.1284
Root an. cond. 4.596564.59656
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.5 − 0.866i)2-s + (−0.499 − 0.866i)4-s + (0.517 + 0.896i)5-s − 0.999·8-s + 1.03·10-s + (−0.133 + 0.232i)11-s + (0.896 + 1.55i)13-s + (−0.5 + 0.866i)16-s − 6.83·17-s − 4.38·19-s + (0.517 − 0.896i)20-s + (0.133 + 0.232i)22-s + (2.73 + 4.73i)23-s + (1.96 − 3.40i)25-s + 1.79·26-s + ⋯
L(s)  = 1  + (0.353 − 0.612i)2-s + (−0.249 − 0.433i)4-s + (0.231 + 0.400i)5-s − 0.353·8-s + 0.327·10-s + (−0.0403 + 0.0699i)11-s + (0.248 + 0.430i)13-s + (−0.125 + 0.216i)16-s − 1.65·17-s − 1.00·19-s + (0.115 − 0.200i)20-s + (0.0285 + 0.0494i)22-s + (0.569 + 0.986i)23-s + (0.392 − 0.680i)25-s + 0.351·26-s + ⋯

Functional equation

Λ(s)=(2646s/2 ΓC(s) L(s)=((0.422−0.906i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 2646 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.422 - 0.906i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(2646s/2 ΓC(s+1/2) L(s)=((0.422−0.906i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 2646 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.422 - 0.906i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 26462646    =    2⋅33⋅722 \cdot 3^{3} \cdot 7^{2}
Sign: 0.422−0.906i0.422 - 0.906i
Analytic conductor: 21.128421.1284
Root analytic conductor: 4.596564.59656
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ2646(883,⋅)\chi_{2646} (883, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 2646, ( :1/2), 0.422−0.906i)(2,\ 2646,\ (\ :1/2),\ 0.422 - 0.906i)

Particular Values

L(1)L(1) ≈\approx 1.3334336151.333433615
L(12)L(\frac12) ≈\approx 1.3334336151.333433615
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1+(−0.5+0.866i)T 1 + (-0.5 + 0.866i)T
3 1 1
7 1 1
good5 1+(−0.517−0.896i)T+(−2.5+4.33i)T2 1 + (-0.517 - 0.896i)T + (-2.5 + 4.33i)T^{2}
11 1+(0.133−0.232i)T+(−5.5−9.52i)T2 1 + (0.133 - 0.232i)T + (-5.5 - 9.52i)T^{2}
13 1+(−0.896−1.55i)T+(−6.5+11.2i)T2 1 + (-0.896 - 1.55i)T + (-6.5 + 11.2i)T^{2}
17 1+6.83T+17T2 1 + 6.83T + 17T^{2}
19 1+4.38T+19T2 1 + 4.38T + 19T^{2}
23 1+(−2.73−4.73i)T+(−11.5+19.9i)T2 1 + (-2.73 - 4.73i)T + (-11.5 + 19.9i)T^{2}
29 1+(2−3.46i)T+(−14.5−25.1i)T2 1 + (2 - 3.46i)T + (-14.5 - 25.1i)T^{2}
31 1+(−3.34−5.79i)T+(−15.5+26.8i)T2 1 + (-3.34 - 5.79i)T + (-15.5 + 26.8i)T^{2}
37 1−7.46T+37T2 1 - 7.46T + 37T^{2}
41 1+(−4.31−7.46i)T+(−20.5+35.5i)T2 1 + (-4.31 - 7.46i)T + (-20.5 + 35.5i)T^{2}
43 1+(0.133−0.232i)T+(−21.5−37.2i)T2 1 + (0.133 - 0.232i)T + (-21.5 - 37.2i)T^{2}
47 1+(0.378−0.656i)T+(−23.5−40.7i)T2 1 + (0.378 - 0.656i)T + (-23.5 - 40.7i)T^{2}
53 1+10.9T+53T2 1 + 10.9T + 53T^{2}
59 1+(−0.637−1.10i)T+(−29.5+51.0i)T2 1 + (-0.637 - 1.10i)T + (-29.5 + 51.0i)T^{2}
61 1+(6.31−10.9i)T+(−30.5−52.8i)T2 1 + (6.31 - 10.9i)T + (-30.5 - 52.8i)T^{2}
67 1+(6.23+10.7i)T+(−33.5+58.0i)T2 1 + (6.23 + 10.7i)T + (-33.5 + 58.0i)T^{2}
71 1+9.46T+71T2 1 + 9.46T + 71T^{2}
73 1+5.41T+73T2 1 + 5.41T + 73T^{2}
79 1+(4.46−7.73i)T+(−39.5−68.4i)T2 1 + (4.46 - 7.73i)T + (-39.5 - 68.4i)T^{2}
83 1+(3.29−5.70i)T+(−41.5−71.8i)T2 1 + (3.29 - 5.70i)T + (-41.5 - 71.8i)T^{2}
89 1−7.07T+89T2 1 - 7.07T + 89T^{2}
97 1+(9.07−15.7i)T+(−48.5−84.0i)T2 1 + (9.07 - 15.7i)T + (-48.5 - 84.0i)T^{2}
show more
show less
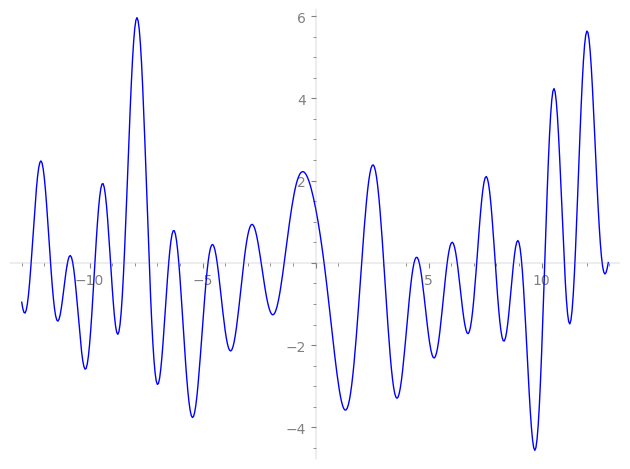
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−9.069430898785647362337613279030, −8.474695590631897327891703967657, −7.35836014521929690482811297822, −6.49801076311127886739193809480, −6.05063969197329179570555368951, −4.75883382212447270263802171926, −4.35275762374032254639276554214, −3.17621336617359618351002460851, −2.40628988757595518830022885550, −1.38777777582587973401121325985, 0.37608286951440586019149715419, 2.03859035898900920146664470968, 3.01828328418148232123461006968, 4.35685619960537945574319479058, 4.58811372933363090468088979615, 5.82304341513114286414476789448, 6.26752903400932846684288603062, 7.12927353924239498033796085633, 7.950120646666905359748944394193, 8.760611698349618937367105282170

Graph of the ZZ-function along the critical line