↑

Properties

Label 2-3380-260.199-c0-0-3
Degree $2$
Conductor $3380$
Sign $-0.499 + 0.866i$
Analytic cond. $1.68683$
Root an. cond. $1.29878$
Motivic weight $0$
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank $0$

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.866 + 0.5i)2-s + (−0.900 + 1.56i)3-s + (0.499 + 0.866i)4-s + i·5-s + (−1.56 + 0.900i)6-s + (−0.385 + 0.222i)7-s + 0.999i·8-s + (−1.12 − 1.94i)9-s + (−0.5 + 0.866i)10-s − 1.80·12-s − 0.445·14-s + (−1.56 − 0.900i)15-s + (−0.5 + 0.866i)16-s − 2.24i·18-s + (−0.866 + 0.499i)20-s − 0.801i·21-s + ⋯
L(s)  = 1  + (0.866 + 0.5i)2-s + (−0.900 + 1.56i)3-s + (0.499 + 0.866i)4-s + i·5-s + (−1.56 + 0.900i)6-s + (−0.385 + 0.222i)7-s + 0.999i·8-s + (−1.12 − 1.94i)9-s + (−0.5 + 0.866i)10-s − 1.80·12-s − 0.445·14-s + (−1.56 − 0.900i)15-s + (−0.5 + 0.866i)16-s − 2.24i·18-s + (−0.866 + 0.499i)20-s − 0.801i·21-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 3380 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.499 + 0.866i)\, \overline{\Lambda}(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 3380 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.499 + 0.866i)\, \overline{\Lambda}(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(3380\)    =    \(2^{2} \cdot 5 \cdot 13^{2}\)
Sign: $-0.499 + 0.866i$
Analytic conductor: \(1.68683\)
Root analytic conductor: \(1.29878\)
Motivic weight: \(0\)
Rational: no
Arithmetic: yes
Character: $\chi_{3380} (1499, \cdot )$
Primitive: yes
Self-dual: no
Analytic rank: \(0\)
Selberg data: \((2,\ 3380,\ (\ :0),\ -0.499 + 0.866i)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(1.164495796\)
\(L(\frac12)\) \(\approx\) \(1.164495796\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad2 \( 1 + (-0.866 - 0.5i)T \)
5 \( 1 - iT \)
13 \( 1 \)
good3 \( 1 + (0.900 - 1.56i)T + (-0.5 - 0.866i)T^{2} \)
7 \( 1 + (0.385 - 0.222i)T + (0.5 - 0.866i)T^{2} \)
11 \( 1 + (-0.5 - 0.866i)T^{2} \)
17 \( 1 + (0.5 - 0.866i)T^{2} \)
19 \( 1 + (-0.5 + 0.866i)T^{2} \)
23 \( 1 + (0.623 - 1.07i)T + (-0.5 - 0.866i)T^{2} \)
29 \( 1 + (-0.900 + 1.56i)T + (-0.5 - 0.866i)T^{2} \)
31 \( 1 + T^{2} \)
37 \( 1 + (-0.5 - 0.866i)T^{2} \)
41 \( 1 + (-0.385 - 0.222i)T + (0.5 + 0.866i)T^{2} \)
43 \( 1 + (0.623 + 1.07i)T + (-0.5 + 0.866i)T^{2} \)
47 \( 1 - 1.24iT - T^{2} \)
53 \( 1 - T^{2} \)
59 \( 1 + (-0.5 + 0.866i)T^{2} \)
61 \( 1 + (-0.222 - 0.385i)T + (-0.5 + 0.866i)T^{2} \)
67 \( 1 + (-1.07 - 0.623i)T + (0.5 + 0.866i)T^{2} \)
71 \( 1 + (-0.5 + 0.866i)T^{2} \)
73 \( 1 + T^{2} \)
79 \( 1 - T^{2} \)
83 \( 1 - 1.80iT - T^{2} \)
89 \( 1 + (-1.07 - 0.623i)T + (0.5 + 0.866i)T^{2} \)
97 \( 1 + (-0.5 + 0.866i)T^{2} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

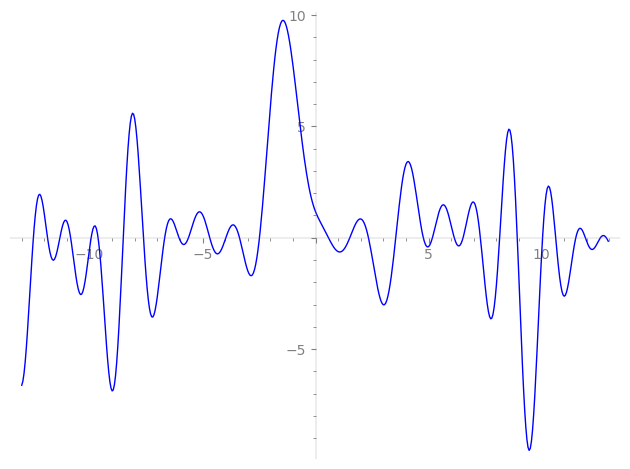
Imaginary part of the first few zeros on the critical line

−9.622138568555005166744702573901, −8.508810752169691237740382640951, −7.61405118205600771669953088113, −6.67604840758029486624224351810, −6.04094550293886930895688259155, −5.62301049518186736211696972087, −4.68208550517152644313435500020, −3.96886677605457110447487823064, −3.36496412211232152366631603809, −2.48308321934877943424920698010, 0.57016178644690592563445887186, 1.51415988002894388174364802078, 2.33961915151079165590267788065, 3.54709859381052730583812569166, 4.76764973664758085161829698644, 5.17007727693803576070613451600, 6.13729235154775748345393095208, 6.52568533544690427915511095798, 7.29142011989877076191377474271, 8.144256571343769364385501600882

Graph of the $Z$-function along the critical line