↑

Properties

Label 2-448-112.27-c1-0-13
Degree 22
Conductor 448448
Sign 0.315+0.948i0.315 + 0.948i
Analytic cond. 3.577293.57729
Root an. cond. 1.891371.89137
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (1.73 − 1.73i)3-s + (1.73 − 1.73i)5-s + (2 − 1.73i)7-s − 2.99i·9-s + (−3 + 3i)11-s + (1.73 + 1.73i)13-s − 5.99i·15-s + (−5.19 + 5.19i)19-s + (0.464 − 6.46i)21-s − 4·23-s − 0.999i·25-s + (−1 + i)29-s + 6.92·31-s + 10.3i·33-s + (0.464 − 6.46i)35-s + ⋯
L(s)  = 1  + (0.999 − 0.999i)3-s + (0.774 − 0.774i)5-s + (0.755 − 0.654i)7-s − 0.999i·9-s + (−0.904 + 0.904i)11-s + (0.480 + 0.480i)13-s − 1.54i·15-s + (−1.19 + 1.19i)19-s + (0.101 − 1.41i)21-s − 0.834·23-s − 0.199i·25-s + (−0.185 + 0.185i)29-s + 1.24·31-s + 1.80i·33-s + (0.0784 − 1.09i)35-s + ⋯

Functional equation

Λ(s)=(448s/2 ΓC(s) L(s)=((0.315+0.948i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 448 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.315 + 0.948i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(448s/2 ΓC(s+1/2) L(s)=((0.315+0.948i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 448 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.315 + 0.948i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 448448    =    26⋅72^{6} \cdot 7
Sign: 0.315+0.948i0.315 + 0.948i
Analytic conductor: 3.577293.57729
Root analytic conductor: 1.891371.89137
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ448(335,⋅)\chi_{448} (335, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 448, ( :1/2), 0.315+0.948i)(2,\ 448,\ (\ :1/2),\ 0.315 + 0.948i)

Particular Values

L(1)L(1) ≈\approx 1.77043−1.27703i1.77043 - 1.27703i
L(12)L(\frac12) ≈\approx 1.77043−1.27703i1.77043 - 1.27703i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1 1
7 1+(−2+1.73i)T 1 + (-2 + 1.73i)T
good3 1+(−1.73+1.73i)T−3iT2 1 + (-1.73 + 1.73i)T - 3iT^{2}
5 1+(−1.73+1.73i)T−5iT2 1 + (-1.73 + 1.73i)T - 5iT^{2}
11 1+(3−3i)T−11iT2 1 + (3 - 3i)T - 11iT^{2}
13 1+(−1.73−1.73i)T+13iT2 1 + (-1.73 - 1.73i)T + 13iT^{2}
17 1−17T2 1 - 17T^{2}
19 1+(5.19−5.19i)T−19iT2 1 + (5.19 - 5.19i)T - 19iT^{2}
23 1+4T+23T2 1 + 4T + 23T^{2}
29 1+(1−i)T−29iT2 1 + (1 - i)T - 29iT^{2}
31 1−6.92T+31T2 1 - 6.92T + 31T^{2}
37 1+(5+5i)T+37iT2 1 + (5 + 5i)T + 37iT^{2}
41 1+3.46T+41T2 1 + 3.46T + 41T^{2}
43 1+(−1+i)T−43iT2 1 + (-1 + i)T - 43iT^{2}
47 1−6.92T+47T2 1 - 6.92T + 47T^{2}
53 1+(−3−3i)T+53iT2 1 + (-3 - 3i)T + 53iT^{2}
59 1+(8.66+8.66i)T+59iT2 1 + (8.66 + 8.66i)T + 59iT^{2}
61 1+(5.19+5.19i)T+61iT2 1 + (5.19 + 5.19i)T + 61iT^{2}
67 1+(−7−7i)T+67iT2 1 + (-7 - 7i)T + 67iT^{2}
71 1+4T+71T2 1 + 4T + 71T^{2}
73 1−10.3T+73T2 1 - 10.3T + 73T^{2}
79 1+2iT−79T2 1 + 2iT - 79T^{2}
83 1+(−1.73+1.73i)T−83iT2 1 + (-1.73 + 1.73i)T - 83iT^{2}
89 1+3.46T+89T2 1 + 3.46T + 89T^{2}
97 1−97T2 1 - 97T^{2}
show more
show less
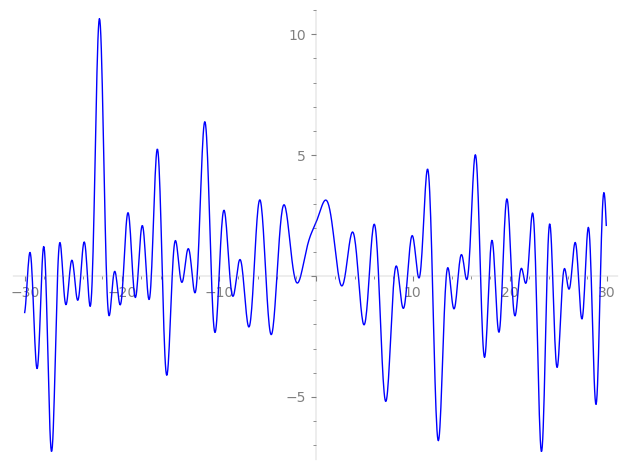
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.75394406722260626457008333998, −9.960250891604774584576080773723, −8.851272935628582998623881583594, −8.150587815170368605984695726553, −7.49297242633907576214351225586, −6.39056139587399566686664815132, −5.13580793369108407138204949821, −4.00026884744127410785103692246, −2.19766122255295951016106398865, −1.55116467791809345414874933503, 2.36467818548282549620160120415, 3.01687137233733318075405017661, 4.39582372557744403637613722850, 5.51427358424172059383476477608, 6.47709431036068933829509773669, 8.097023630883514118928827000810, 8.533198746207018025616520850098, 9.458868589011985383933622960679, 10.55145719360837288237524214178, 10.72767159300261519967591279242

Graph of the ZZ-function along the critical line