↑

Properties

Label 2-464-29.24-c1-0-1
Degree 22
Conductor 464464
Sign −0.712−0.701i-0.712 - 0.701i
Analytic cond. 3.705053.70505
Root an. cond. 1.924851.92485
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.760 + 3.33i)3-s + (1.00 + 1.26i)5-s + (−0.338 − 1.48i)7-s + (−7.81 + 3.76i)9-s + (1.70 + 0.822i)11-s + (2.87 + 1.38i)13-s + (−3.44 + 4.31i)15-s − 1.69·17-s + (−0.818 + 3.58i)19-s + (4.68 − 2.25i)21-s + (1.67 − 2.10i)23-s + (0.531 − 2.33i)25-s + (−12.1 − 15.1i)27-s + (−4.31 − 3.21i)29-s + (1.11 + 1.39i)31-s + ⋯
L(s)  = 1  + (0.439 + 1.92i)3-s + (0.450 + 0.564i)5-s + (−0.127 − 0.560i)7-s + (−2.60 + 1.25i)9-s + (0.515 + 0.248i)11-s + (0.796 + 0.383i)13-s + (−0.888 + 1.11i)15-s − 0.412·17-s + (−0.187 + 0.822i)19-s + (1.02 − 0.492i)21-s + (0.349 − 0.438i)23-s + (0.106 − 0.466i)25-s + (−2.32 − 2.92i)27-s + (−0.801 − 0.597i)29-s + (0.199 + 0.250i)31-s + ⋯

Functional equation

Λ(s)=(464s/2 ΓC(s) L(s)=((−0.712−0.701i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 464 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.712 - 0.701i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(464s/2 ΓC(s+1/2) L(s)=((−0.712−0.701i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 464 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (-0.712 - 0.701i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 464464    =    24⋅292^{4} \cdot 29
Sign: −0.712−0.701i-0.712 - 0.701i
Analytic conductor: 3.705053.70505
Root analytic conductor: 1.924851.92485
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ464(401,⋅)\chi_{464} (401, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 464, ( :1/2), −0.712−0.701i)(2,\ 464,\ (\ :1/2),\ -0.712 - 0.701i)

Particular Values

L(1)L(1) ≈\approx 0.615477+1.50203i0.615477 + 1.50203i
L(12)L(\frac12) ≈\approx 0.615477+1.50203i0.615477 + 1.50203i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1 1
29 1+(4.31+3.21i)T 1 + (4.31 + 3.21i)T
good3 1+(−0.760−3.33i)T+(−2.70+1.30i)T2 1 + (-0.760 - 3.33i)T + (-2.70 + 1.30i)T^{2}
5 1+(−1.00−1.26i)T+(−1.11+4.87i)T2 1 + (-1.00 - 1.26i)T + (-1.11 + 4.87i)T^{2}
7 1+(0.338+1.48i)T+(−6.30+3.03i)T2 1 + (0.338 + 1.48i)T + (-6.30 + 3.03i)T^{2}
11 1+(−1.70−0.822i)T+(6.85+8.60i)T2 1 + (-1.70 - 0.822i)T + (6.85 + 8.60i)T^{2}
13 1+(−2.87−1.38i)T+(8.10+10.1i)T2 1 + (-2.87 - 1.38i)T + (8.10 + 10.1i)T^{2}
17 1+1.69T+17T2 1 + 1.69T + 17T^{2}
19 1+(0.818−3.58i)T+(−17.1−8.24i)T2 1 + (0.818 - 3.58i)T + (-17.1 - 8.24i)T^{2}
23 1+(−1.67+2.10i)T+(−5.11−22.4i)T2 1 + (-1.67 + 2.10i)T + (-5.11 - 22.4i)T^{2}
31 1+(−1.11−1.39i)T+(−6.89+30.2i)T2 1 + (-1.11 - 1.39i)T + (-6.89 + 30.2i)T^{2}
37 1+(−6.37+3.06i)T+(23.0−28.9i)T2 1 + (-6.37 + 3.06i)T + (23.0 - 28.9i)T^{2}
41 1+5.03T+41T2 1 + 5.03T + 41T^{2}
43 1+(−1.18+1.48i)T+(−9.56−41.9i)T2 1 + (-1.18 + 1.48i)T + (-9.56 - 41.9i)T^{2}
47 1+(−2.31−1.11i)T+(29.3+36.7i)T2 1 + (-2.31 - 1.11i)T + (29.3 + 36.7i)T^{2}
53 1+(−5.14−6.44i)T+(−11.7+51.6i)T2 1 + (-5.14 - 6.44i)T + (-11.7 + 51.6i)T^{2}
59 1+2.95T+59T2 1 + 2.95T + 59T^{2}
61 1+(−1.72−7.55i)T+(−54.9+26.4i)T2 1 + (-1.72 - 7.55i)T + (-54.9 + 26.4i)T^{2}
67 1+(−2.23+1.07i)T+(41.7−52.3i)T2 1 + (-2.23 + 1.07i)T + (41.7 - 52.3i)T^{2}
71 1+(−5.46−2.63i)T+(44.2+55.5i)T2 1 + (-5.46 - 2.63i)T + (44.2 + 55.5i)T^{2}
73 1+(−2.33+2.93i)T+(−16.2−71.1i)T2 1 + (-2.33 + 2.93i)T + (-16.2 - 71.1i)T^{2}
79 1+(−8.30+4.00i)T+(49.2−61.7i)T2 1 + (-8.30 + 4.00i)T + (49.2 - 61.7i)T^{2}
83 1+(1.22−5.38i)T+(−74.7−36.0i)T2 1 + (1.22 - 5.38i)T + (-74.7 - 36.0i)T^{2}
89 1+(−9.95−12.4i)T+(−19.8+86.7i)T2 1 + (-9.95 - 12.4i)T + (-19.8 + 86.7i)T^{2}
97 1+(3.31−14.5i)T+(−87.3−42.0i)T2 1 + (3.31 - 14.5i)T + (-87.3 - 42.0i)T^{2}
show more
show less
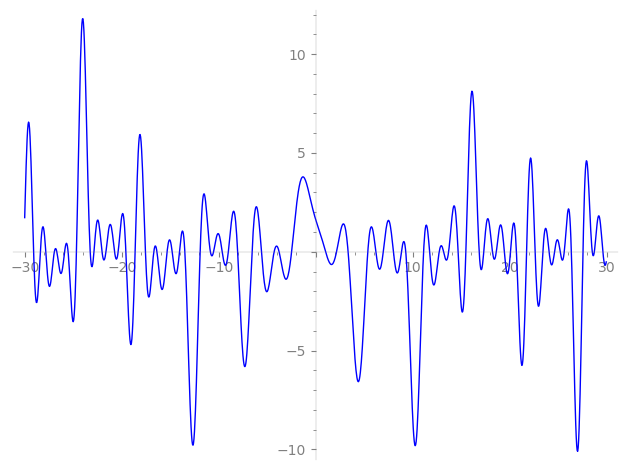
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.85743464102314270297358537952, −10.53063768887285285672017130198, −9.641449398601057299927872607666, −9.007950727387200401026629290701, −8.049827910076534516127810892828, −6.56531993905283643740252528931, −5.59410865504235806617607765887, −4.28517537281962612865865388242, −3.77610315713645486682598900420, −2.48738137143071201227512970315, 1.01703864079630608611094691626, 2.14555504329320380835915004101, 3.33795268404707396111420087610, 5.36868356401212993050070282498, 6.20120128095594458551448710312, 6.96203569374746893776100307101, 8.011025390220776620630465657335, 8.834696460599497613628830459364, 9.279651858090733368149530468775, 11.11855370648164043237860577776

Graph of the ZZ-function along the critical line