↑

Properties

Label 2-630-5.4-c1-0-9
Degree 22
Conductor 630630
Sign 0.894+0.447i0.894 + 0.447i
Analytic cond. 5.030575.03057
Root an. cond. 2.242892.24289
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + i·2-s − 4-s + (−2 − i)5-s + i·7-s − i·8-s + (1 − 2i)10-s − 4i·13-s − 14-s + 16-s − 2i·17-s + 8·19-s + (2 + i)20-s − 8i·23-s + (3 + 4i)25-s + 4·26-s + ⋯
L(s)  = 1  + 0.707i·2-s − 0.5·4-s + (−0.894 − 0.447i)5-s + 0.377i·7-s − 0.353i·8-s + (0.316 − 0.632i)10-s − 1.10i·13-s − 0.267·14-s + 0.250·16-s − 0.485i·17-s + 1.83·19-s + (0.447 + 0.223i)20-s − 1.66i·23-s + (0.600 + 0.800i)25-s + 0.784·26-s + ⋯

Functional equation

Λ(s)=(630s/2 ΓC(s) L(s)=((0.894+0.447i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 630 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.894 + 0.447i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(630s/2 ΓC(s+1/2) L(s)=((0.894+0.447i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 630 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.894 + 0.447i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 630630    =    2⋅32⋅5⋅72 \cdot 3^{2} \cdot 5 \cdot 7
Sign: 0.894+0.447i0.894 + 0.447i
Analytic conductor: 5.030575.03057
Root analytic conductor: 2.242892.24289
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ630(379,⋅)\chi_{630} (379, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 630, ( :1/2), 0.894+0.447i)(2,\ 630,\ (\ :1/2),\ 0.894 + 0.447i)

Particular Values

L(1)L(1) ≈\approx 1.00663−0.237634i1.00663 - 0.237634i
L(12)L(\frac12) ≈\approx 1.00663−0.237634i1.00663 - 0.237634i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1−iT 1 - iT
3 1 1
5 1+(2+i)T 1 + (2 + i)T
7 1−iT 1 - iT
good11 1+11T2 1 + 11T^{2}
13 1+4iT−13T2 1 + 4iT - 13T^{2}
17 1+2iT−17T2 1 + 2iT - 17T^{2}
19 1−8T+19T2 1 - 8T + 19T^{2}
23 1+8iT−23T2 1 + 8iT - 23T^{2}
29 1+8T+29T2 1 + 8T + 29T^{2}
31 1−4T+31T2 1 - 4T + 31T^{2}
37 1+8iT−37T2 1 + 8iT - 37T^{2}
41 1−12T+41T2 1 - 12T + 41T^{2}
43 1+8iT−43T2 1 + 8iT - 43T^{2}
47 1+4iT−47T2 1 + 4iT - 47T^{2}
53 1−6iT−53T2 1 - 6iT - 53T^{2}
59 1+8T+59T2 1 + 8T + 59T^{2}
61 1+6T+61T2 1 + 6T + 61T^{2}
67 1−8iT−67T2 1 - 8iT - 67T^{2}
71 1+71T2 1 + 71T^{2}
73 1−4iT−73T2 1 - 4iT - 73T^{2}
79 1+8T+79T2 1 + 8T + 79T^{2}
83 1−83T2 1 - 83T^{2}
89 1−4T+89T2 1 - 4T + 89T^{2}
97 1+12iT−97T2 1 + 12iT - 97T^{2}
show more
show less
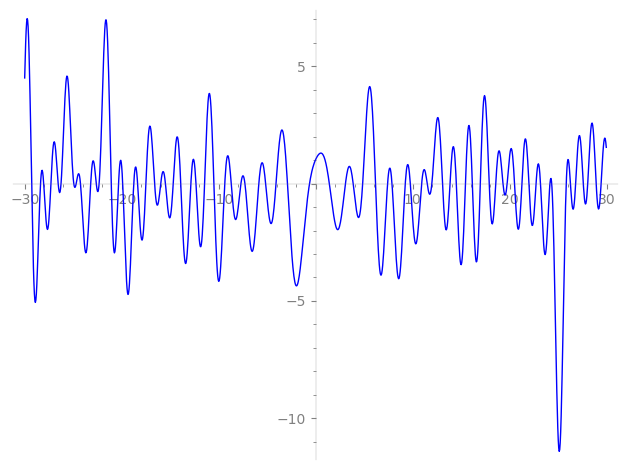
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.49839725824576295199357472012, −9.375143355219890940722532391734, −8.710471852531880161530895307943, −7.70978173658112462198926068231, −7.29784820732422506511104865715, −5.86477934218199323320095027219, −5.16243260990912172191610139147, −4.09237732048846523750102007496, −2.91353021490250363503403738067, −0.63892303427725341813276550352, 1.40245221211779527951862810289, 3.06440711733884952843546864526, 3.85889533379040075872733995812, 4.83849137185205450889737786480, 6.16337119095468751719743091885, 7.40815005074694681976714668290, 7.86094990335840108523802619017, 9.231004043744810732276820062365, 9.750325669363806175511469432261, 10.91946341175581805790662520964

Graph of the ZZ-function along the critical line