↑

Properties

Label 2-672-168.11-c1-0-11
Degree 22
Conductor 672672
Sign 0.982−0.188i0.982 - 0.188i
Analytic cond. 5.365945.36594
Root an. cond. 2.316452.31645
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−1.66 + 0.493i)3-s + (1.09 + 1.89i)5-s + (0.451 − 2.60i)7-s + (2.51 − 1.63i)9-s + (1.45 + 0.837i)11-s − 1.56i·13-s + (−2.74 − 2.60i)15-s + (−0.278 − 0.160i)17-s + (−2.87 − 4.97i)19-s + (0.537 + 4.55i)21-s + (3.26 + 5.65i)23-s + (0.114 − 0.198i)25-s + (−3.36 + 3.96i)27-s + 7.04·29-s + (7.76 + 4.48i)31-s + ⋯
L(s)  = 1  + (−0.958 + 0.285i)3-s + (0.488 + 0.845i)5-s + (0.170 − 0.985i)7-s + (0.837 − 0.546i)9-s + (0.437 + 0.252i)11-s − 0.432i·13-s + (−0.709 − 0.671i)15-s + (−0.0676 − 0.0390i)17-s + (−0.659 − 1.14i)19-s + (0.117 + 0.993i)21-s + (0.681 + 1.17i)23-s + (0.0229 − 0.0396i)25-s + (−0.646 + 0.762i)27-s + 1.30·29-s + (1.39 + 0.805i)31-s + ⋯

Functional equation

Λ(s)=(672s/2 ΓC(s) L(s)=((0.982−0.188i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 672 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.982 - 0.188i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(672s/2 ΓC(s+1/2) L(s)=((0.982−0.188i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 672 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.982 - 0.188i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 672672    =    25⋅3⋅72^{5} \cdot 3 \cdot 7
Sign: 0.982−0.188i0.982 - 0.188i
Analytic conductor: 5.365945.36594
Root analytic conductor: 2.316452.31645
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ672(431,⋅)\chi_{672} (431, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 672, ( :1/2), 0.982−0.188i)(2,\ 672,\ (\ :1/2),\ 0.982 - 0.188i)

Particular Values

L(1)L(1) ≈\approx 1.26555+0.120380i1.26555 + 0.120380i
L(12)L(\frac12) ≈\approx 1.26555+0.120380i1.26555 + 0.120380i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1 1
3 1+(1.66−0.493i)T 1 + (1.66 - 0.493i)T
7 1+(−0.451+2.60i)T 1 + (-0.451 + 2.60i)T
good5 1+(−1.09−1.89i)T+(−2.5+4.33i)T2 1 + (-1.09 - 1.89i)T + (-2.5 + 4.33i)T^{2}
11 1+(−1.45−0.837i)T+(5.5+9.52i)T2 1 + (-1.45 - 0.837i)T + (5.5 + 9.52i)T^{2}
13 1+1.56iT−13T2 1 + 1.56iT - 13T^{2}
17 1+(0.278+0.160i)T+(8.5+14.7i)T2 1 + (0.278 + 0.160i)T + (8.5 + 14.7i)T^{2}
19 1+(2.87+4.97i)T+(−9.5+16.4i)T2 1 + (2.87 + 4.97i)T + (-9.5 + 16.4i)T^{2}
23 1+(−3.26−5.65i)T+(−11.5+19.9i)T2 1 + (-3.26 - 5.65i)T + (-11.5 + 19.9i)T^{2}
29 1−7.04T+29T2 1 - 7.04T + 29T^{2}
31 1+(−7.76−4.48i)T+(15.5+26.8i)T2 1 + (-7.76 - 4.48i)T + (15.5 + 26.8i)T^{2}
37 1+(−0.946+0.546i)T+(18.5−32.0i)T2 1 + (-0.946 + 0.546i)T + (18.5 - 32.0i)T^{2}
41 1+3.44iT−41T2 1 + 3.44iT - 41T^{2}
43 1−11.6T+43T2 1 - 11.6T + 43T^{2}
47 1+(−2.25−3.90i)T+(−23.5+40.7i)T2 1 + (-2.25 - 3.90i)T + (-23.5 + 40.7i)T^{2}
53 1+(4.42−7.67i)T+(−26.5−45.8i)T2 1 + (4.42 - 7.67i)T + (-26.5 - 45.8i)T^{2}
59 1+(5.37+3.10i)T+(29.5+51.0i)T2 1 + (5.37 + 3.10i)T + (29.5 + 51.0i)T^{2}
61 1+(−3.80+2.19i)T+(30.5−52.8i)T2 1 + (-3.80 + 2.19i)T + (30.5 - 52.8i)T^{2}
67 1+(0.716−1.24i)T+(−33.5−58.0i)T2 1 + (0.716 - 1.24i)T + (-33.5 - 58.0i)T^{2}
71 1−6.37T+71T2 1 - 6.37T + 71T^{2}
73 1+(4.49−7.77i)T+(−36.5−63.2i)T2 1 + (4.49 - 7.77i)T + (-36.5 - 63.2i)T^{2}
79 1+(−3.58+2.07i)T+(39.5−68.4i)T2 1 + (-3.58 + 2.07i)T + (39.5 - 68.4i)T^{2}
83 1+9.06iT−83T2 1 + 9.06iT - 83T^{2}
89 1+(−4.57+2.64i)T+(44.5−77.0i)T2 1 + (-4.57 + 2.64i)T + (44.5 - 77.0i)T^{2}
97 1−4.23T+97T2 1 - 4.23T + 97T^{2}
show more
show less
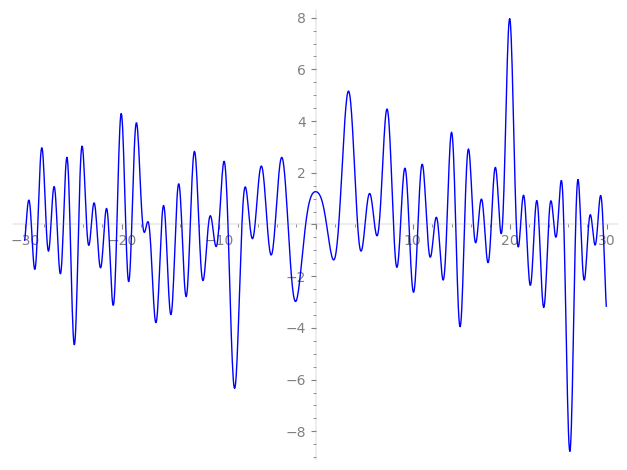
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.65067438052112579374656976473, −9.967392569365098419867796232857, −9.047637746933330444654188467343, −7.60607428345784660637800341060, −6.79393330629371334815965874833, −6.25577678369048567540457599444, −4.99794744931052194245462834413, −4.18367968130574883797994907628, −2.85465793280021317871943817650, −1.04101922771862168294472133804, 1.11772078575837535168978741870, 2.37154115504505573980526093247, 4.31743447306163495206882182477, 5.08284785578325137829620472059, 6.06500615978195378971839681642, 6.53730810416618329294284843531, 8.015472152129602021917846587247, 8.745501626997243903087725562614, 9.616210322301302814318405194819, 10.54112400353428642845564658909

Graph of the ZZ-function along the critical line