↑

Properties

Label 2-825-5.4-c3-0-22
Degree 22
Conductor 825825
Sign −0.894−0.447i-0.894 - 0.447i
Analytic cond. 48.676548.6765
Root an. cond. 6.976866.97686
Motivic weight 33
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + 4.97i·2-s − 3i·3-s − 16.7·4-s + 14.9·6-s + 5.48i·7-s − 43.3i·8-s − 9·9-s + 11·11-s + 50.1i·12-s − 24.5i·13-s − 27.2·14-s + 81.6·16-s − 59.3i·17-s − 44.7i·18-s − 5.89·19-s + ⋯
L(s)  = 1  + 1.75i·2-s − 0.577i·3-s − 2.08·4-s + 1.01·6-s + 0.296i·7-s − 1.91i·8-s − 0.333·9-s + 0.301·11-s + 1.20i·12-s − 0.524i·13-s − 0.520·14-s + 1.27·16-s − 0.847i·17-s − 0.585i·18-s − 0.0711·19-s + ⋯

Functional equation

Λ(s)=(825s/2 ΓC(s) L(s)=((−0.894−0.447i) Λ‾(4−s)\begin{aligned}\Lambda(s)=\mathstrut & 825 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.894 - 0.447i)\, \overline{\Lambda}(4-s) \end{aligned}
Λ(s)=(825s/2 ΓC(s+3/2) L(s)=((−0.894−0.447i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 825 ^{s/2} \, \Gamma_{\C}(s+3/2) \, L(s)\cr =\mathstrut & (-0.894 - 0.447i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 825825    =    3⋅52⋅113 \cdot 5^{2} \cdot 11
Sign: −0.894−0.447i-0.894 - 0.447i
Analytic conductor: 48.676548.6765
Root analytic conductor: 6.976866.97686
Motivic weight: 33
Rational: no
Arithmetic: yes
Character: χ825(199,⋅)\chi_{825} (199, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 825, ( :3/2), −0.894−0.447i)(2,\ 825,\ (\ :3/2),\ -0.894 - 0.447i)

Particular Values

L(2)L(2) ≈\approx 1.4333407341.433340734
L(12)L(\frac12) ≈\approx 1.4333407341.433340734
L(52)L(\frac{5}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad3 1+3iT 1 + 3iT
5 1 1
11 1−11T 1 - 11T
good2 1−4.97iT−8T2 1 - 4.97iT - 8T^{2}
7 1−5.48iT−343T2 1 - 5.48iT - 343T^{2}
13 1+24.5iT−2.19e3T2 1 + 24.5iT - 2.19e3T^{2}
17 1+59.3iT−4.91e3T2 1 + 59.3iT - 4.91e3T^{2}
19 1+5.89T+6.85e3T2 1 + 5.89T + 6.85e3T^{2}
23 1−68.4iT−1.21e4T2 1 - 68.4iT - 1.21e4T^{2}
29 1−265.T+2.43e4T2 1 - 265.T + 2.43e4T^{2}
31 1+196.T+2.97e4T2 1 + 196.T + 2.97e4T^{2}
37 1−166.iT−5.06e4T2 1 - 166. iT - 5.06e4T^{2}
41 1−424.T+6.89e4T2 1 - 424.T + 6.89e4T^{2}
43 1−177.iT−7.95e4T2 1 - 177. iT - 7.95e4T^{2}
47 1−141.iT−1.03e5T2 1 - 141. iT - 1.03e5T^{2}
53 1−339.iT−1.48e5T2 1 - 339. iT - 1.48e5T^{2}
59 1+416.T+2.05e5T2 1 + 416.T + 2.05e5T^{2}
61 1−662.T+2.26e5T2 1 - 662.T + 2.26e5T^{2}
67 1−313.iT−3.00e5T2 1 - 313. iT - 3.00e5T^{2}
71 1+153.T+3.57e5T2 1 + 153.T + 3.57e5T^{2}
73 1+153.iT−3.89e5T2 1 + 153. iT - 3.89e5T^{2}
79 1+403.T+4.93e5T2 1 + 403.T + 4.93e5T^{2}
83 1−652.iT−5.71e5T2 1 - 652. iT - 5.71e5T^{2}
89 1+1.22e3T+7.04e5T2 1 + 1.22e3T + 7.04e5T^{2}
97 1−959.iT−9.12e5T2 1 - 959. iT - 9.12e5T^{2}
show more
show less
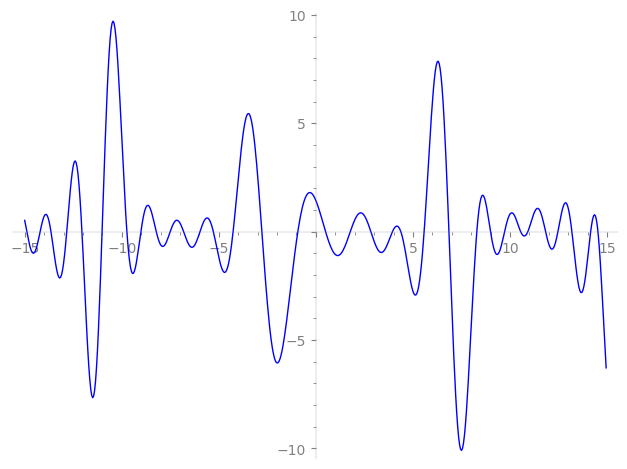
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−9.735129307905865494755214278691, −9.014223582511925788466147782831, −8.222442823441254634169944053694, −7.50491342153128530059579954211, −6.81363901096238439245231268137, −5.97123731429077855535952746905, −5.28277252274552073245733820714, −4.26486558659487439820287666440, −2.78316261502009388736625661354, −0.931091076678150464713588190043, 0.49894755797449731922978876321, 1.77105345417228666139525434763, 2.83073795781045484529770970591, 3.94242892453941661332561963177, 4.37429123430493752225928330783, 5.57489993554492383010913253612, 6.85178230249117176547417292095, 8.288514750313401562848550273326, 8.995741904051576072763499012853, 9.719403420406324237010382383421

Graph of the ZZ-function along the critical line