↑

Properties

Label 2-975-13.9-c1-0-18
Degree 22
Conductor 975975
Sign 0.859−0.511i0.859 - 0.511i
Analytic cond. 7.785417.78541
Root an. cond. 2.790232.79023
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.5 − 0.866i)2-s + (−0.5 + 0.866i)3-s + (0.500 + 0.866i)4-s + (0.499 + 0.866i)6-s + (1 + 1.73i)7-s + 3·8-s + (−0.499 − 0.866i)9-s + (1 − 1.73i)11-s − 12-s + (3.5 + 0.866i)13-s + 1.99·14-s + (0.500 − 0.866i)16-s + (−3.5 − 6.06i)17-s − 0.999·18-s + (3 + 5.19i)19-s + ⋯
L(s)  = 1  + (0.353 − 0.612i)2-s + (−0.288 + 0.499i)3-s + (0.250 + 0.433i)4-s + (0.204 + 0.353i)6-s + (0.377 + 0.654i)7-s + 1.06·8-s + (−0.166 − 0.288i)9-s + (0.301 − 0.522i)11-s − 0.288·12-s + (0.970 + 0.240i)13-s + 0.534·14-s + (0.125 − 0.216i)16-s + (−0.848 − 1.47i)17-s − 0.235·18-s + (0.688 + 1.19i)19-s + ⋯

Functional equation

Λ(s)=(975s/2 ΓC(s) L(s)=((0.859−0.511i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 975 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.859 - 0.511i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(975s/2 ΓC(s+1/2) L(s)=((0.859−0.511i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 975 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.859 - 0.511i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 975975    =    3⋅52⋅133 \cdot 5^{2} \cdot 13
Sign: 0.859−0.511i0.859 - 0.511i
Analytic conductor: 7.785417.78541
Root analytic conductor: 2.790232.79023
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ975(451,⋅)\chi_{975} (451, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 975, ( :1/2), 0.859−0.511i)(2,\ 975,\ (\ :1/2),\ 0.859 - 0.511i)

Particular Values

L(1)L(1) ≈\approx 2.06286+0.566944i2.06286 + 0.566944i
L(12)L(\frac12) ≈\approx 2.06286+0.566944i2.06286 + 0.566944i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad3 1+(0.5−0.866i)T 1 + (0.5 - 0.866i)T
5 1 1
13 1+(−3.5−0.866i)T 1 + (-3.5 - 0.866i)T
good2 1+(−0.5+0.866i)T+(−1−1.73i)T2 1 + (-0.5 + 0.866i)T + (-1 - 1.73i)T^{2}
7 1+(−1−1.73i)T+(−3.5+6.06i)T2 1 + (-1 - 1.73i)T + (-3.5 + 6.06i)T^{2}
11 1+(−1+1.73i)T+(−5.5−9.52i)T2 1 + (-1 + 1.73i)T + (-5.5 - 9.52i)T^{2}
17 1+(3.5+6.06i)T+(−8.5+14.7i)T2 1 + (3.5 + 6.06i)T + (-8.5 + 14.7i)T^{2}
19 1+(−3−5.19i)T+(−9.5+16.4i)T2 1 + (-3 - 5.19i)T + (-9.5 + 16.4i)T^{2}
23 1+(3−5.19i)T+(−11.5−19.9i)T2 1 + (3 - 5.19i)T + (-11.5 - 19.9i)T^{2}
29 1+(−0.5+0.866i)T+(−14.5−25.1i)T2 1 + (-0.5 + 0.866i)T + (-14.5 - 25.1i)T^{2}
31 1−4T+31T2 1 - 4T + 31T^{2}
37 1+(−0.5+0.866i)T+(−18.5−32.0i)T2 1 + (-0.5 + 0.866i)T + (-18.5 - 32.0i)T^{2}
41 1+(4.5−7.79i)T+(−20.5−35.5i)T2 1 + (4.5 - 7.79i)T + (-20.5 - 35.5i)T^{2}
43 1+(−3−5.19i)T+(−21.5+37.2i)T2 1 + (-3 - 5.19i)T + (-21.5 + 37.2i)T^{2}
47 1+6T+47T2 1 + 6T + 47T^{2}
53 1−9T+53T2 1 - 9T + 53T^{2}
59 1+(−29.5+51.0i)T2 1 + (-29.5 + 51.0i)T^{2}
61 1+(0.5+0.866i)T+(−30.5+52.8i)T2 1 + (0.5 + 0.866i)T + (-30.5 + 52.8i)T^{2}
67 1+(1−1.73i)T+(−33.5−58.0i)T2 1 + (1 - 1.73i)T + (-33.5 - 58.0i)T^{2}
71 1+(3+5.19i)T+(−35.5+61.4i)T2 1 + (3 + 5.19i)T + (-35.5 + 61.4i)T^{2}
73 1+11T+73T2 1 + 11T + 73T^{2}
79 1+4T+79T2 1 + 4T + 79T^{2}
83 1−14T+83T2 1 - 14T + 83T^{2}
89 1+(−7+12.1i)T+(−44.5−77.0i)T2 1 + (-7 + 12.1i)T + (-44.5 - 77.0i)T^{2}
97 1+(1+1.73i)T+(−48.5+84.0i)T2 1 + (1 + 1.73i)T + (-48.5 + 84.0i)T^{2}
show more
show less
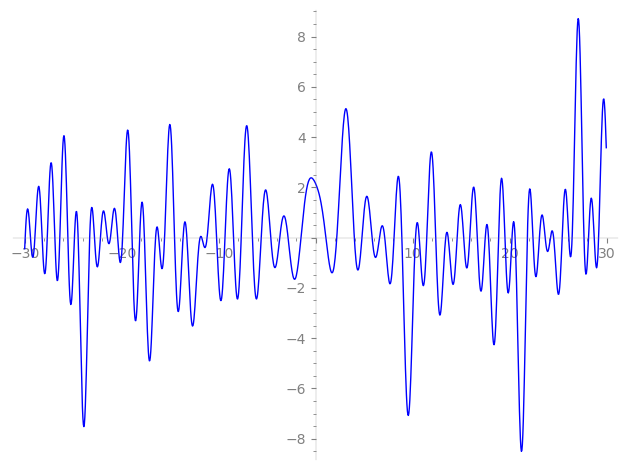
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.21818677558584196905902620188, −9.353207798472219718059114735473, −8.471658067851327598948094056383, −7.68070126782303781015585045849, −6.53331360353935987321803524227, −5.61977205413655949854890441339, −4.63377611233615817552777812590, −3.70705435397126112206404499671, −2.84936832497497622174317357224, −1.52046856734953241814898679373, 1.04866736705705732431020383137, 2.16939868031340990616177510771, 3.97849771921763688101968870004, 4.76306152758463431978843986820, 5.81267216774949321709633951043, 6.57136884557580550893840586768, 7.11476239730583052951184189369, 8.073787969366282799835236718343, 8.878815298447009765257447612629, 10.28747122814160851206407319985

Graph of the ZZ-function along the critical line