↑

Properties

Label 2-1920-120.29-c0-0-6
Degree $2$
Conductor $1920$
Sign $1$
Analytic cond. $0.958204$
Root an. cond. $0.978879$
Motivic weight $0$
Arithmetic yes
Rational yes
Primitive yes
Self-dual yes
Analytic rank $0$

Origins

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + 3-s + 5-s + 9-s + 15-s − 2·23-s + 25-s + 27-s − 2·29-s − 2·43-s + 45-s + 2·47-s + 49-s − 2·67-s − 2·69-s + 75-s + 81-s − 2·87-s + 2·101-s − 2·115-s + ⋯
L(s)  = 1  + 3-s + 5-s + 9-s + 15-s − 2·23-s + 25-s + 27-s − 2·29-s − 2·43-s + 45-s + 2·47-s + 49-s − 2·67-s − 2·69-s + 75-s + 81-s − 2·87-s + 2·101-s − 2·115-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 1920 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & \, \Lambda(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 1920 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & \, \Lambda(1-s) \end{aligned}\]

Invariants

Degree: \(2\)
Conductor: \(1920\)    =    \(2^{7} \cdot 3 \cdot 5\)
Sign: $1$
Analytic conductor: \(0.958204\)
Root analytic conductor: \(0.978879\)
Motivic weight: \(0\)
Rational: yes
Arithmetic: yes
Character: $\chi_{1920} (449, \cdot )$
Primitive: yes
Self-dual: yes
Analytic rank: \(0\)
Selberg data: \((2,\ 1920,\ (\ :0),\ 1)\)

Particular Values

\(L(\frac{1}{2})\) \(\approx\) \(1.885009323\)
\(L(\frac12)\) \(\approx\) \(1.885009323\)
\(L(1)\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$F_p(T)$
bad2 \( 1 \)
3 \( 1 - T \)
5 \( 1 - T \)
good7 \( ( 1 - T )( 1 + T ) \)
11 \( 1 + T^{2} \)
13 \( 1 + T^{2} \)
17 \( 1 + T^{2} \)
19 \( ( 1 - T )( 1 + T ) \)
23 \( ( 1 + T )^{2} \)
29 \( ( 1 + T )^{2} \)
31 \( 1 + T^{2} \)
37 \( 1 + T^{2} \)
41 \( ( 1 - T )( 1 + T ) \)
43 \( ( 1 + T )^{2} \)
47 \( ( 1 - T )^{2} \)
53 \( ( 1 - T )( 1 + T ) \)
59 \( 1 + T^{2} \)
61 \( ( 1 - T )( 1 + T ) \)
67 \( ( 1 + T )^{2} \)
71 \( ( 1 - T )( 1 + T ) \)
73 \( ( 1 - T )( 1 + T ) \)
79 \( 1 + T^{2} \)
83 \( ( 1 - T )( 1 + T ) \)
89 \( ( 1 - T )( 1 + T ) \)
97 \( ( 1 - T )( 1 + T ) \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

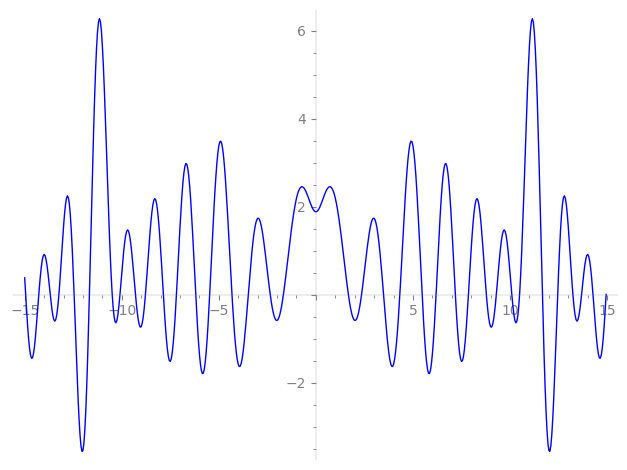
Imaginary part of the first few zeros on the critical line

−9.292538845169000671451836209939, −8.780910300492600097267113344300, −7.85564758843381750571978705382, −7.18427323727915640917830058966, −6.18921084075095116236138322392, −5.47194327604924545404379565562, −4.32279217912789080584434997630, −3.48418478593803961018549036198, −2.35125817870869143538302608520, −1.67444121606388394473781174539, 1.67444121606388394473781174539, 2.35125817870869143538302608520, 3.48418478593803961018549036198, 4.32279217912789080584434997630, 5.47194327604924545404379565562, 6.18921084075095116236138322392, 7.18427323727915640917830058966, 7.85564758843381750571978705382, 8.780910300492600097267113344300, 9.292538845169000671451836209939

Graph of the $Z$-function along the critical line