↑

Properties

Label 2-550-11.4-c1-0-12
Degree 22
Conductor 550550
Sign 0.970−0.242i0.970 - 0.242i
Analytic cond. 4.391774.39177
Root an. cond. 2.095652.09565
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (0.809 + 0.587i)2-s + (0.5 − 1.53i)3-s + (0.309 + 0.951i)4-s + (1.30 − 0.951i)6-s + (0.690 + 2.12i)7-s + (−0.309 + 0.951i)8-s + (0.309 + 0.224i)9-s + (3.23 − 0.726i)11-s + 1.61·12-s + (0.190 + 0.138i)13-s + (−0.690 + 2.12i)14-s + (−0.809 + 0.587i)16-s + (−0.309 + 0.224i)17-s + (0.118 + 0.363i)18-s + (0.809 − 2.48i)19-s + ⋯
L(s)  = 1  + (0.572 + 0.415i)2-s + (0.288 − 0.888i)3-s + (0.154 + 0.475i)4-s + (0.534 − 0.388i)6-s + (0.261 + 0.803i)7-s + (−0.109 + 0.336i)8-s + (0.103 + 0.0748i)9-s + (0.975 − 0.219i)11-s + 0.467·12-s + (0.0529 + 0.0384i)13-s + (−0.184 + 0.568i)14-s + (−0.202 + 0.146i)16-s + (−0.0749 + 0.0544i)17-s + (0.0278 + 0.0856i)18-s + (0.185 − 0.571i)19-s + ⋯

Functional equation

Λ(s)=(550s/2 ΓC(s) L(s)=((0.970−0.242i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 550 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.970 - 0.242i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(550s/2 ΓC(s+1/2) L(s)=((0.970−0.242i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 550 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.970 - 0.242i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 550550    =    2⋅52⋅112 \cdot 5^{2} \cdot 11
Sign: 0.970−0.242i0.970 - 0.242i
Analytic conductor: 4.391774.39177
Root analytic conductor: 2.095652.09565
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ550(301,⋅)\chi_{550} (301, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 550, ( :1/2), 0.970−0.242i)(2,\ 550,\ (\ :1/2),\ 0.970 - 0.242i)

Particular Values

L(1)L(1) ≈\approx 2.33946+0.287582i2.33946 + 0.287582i
L(12)L(\frac12) ≈\approx 2.33946+0.287582i2.33946 + 0.287582i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1+(−0.809−0.587i)T 1 + (-0.809 - 0.587i)T
5 1 1
11 1+(−3.23+0.726i)T 1 + (-3.23 + 0.726i)T
good3 1+(−0.5+1.53i)T+(−2.42−1.76i)T2 1 + (-0.5 + 1.53i)T + (-2.42 - 1.76i)T^{2}
7 1+(−0.690−2.12i)T+(−5.66+4.11i)T2 1 + (-0.690 - 2.12i)T + (-5.66 + 4.11i)T^{2}
13 1+(−0.190−0.138i)T+(4.01+12.3i)T2 1 + (-0.190 - 0.138i)T + (4.01 + 12.3i)T^{2}
17 1+(0.309−0.224i)T+(5.25−16.1i)T2 1 + (0.309 - 0.224i)T + (5.25 - 16.1i)T^{2}
19 1+(−0.809+2.48i)T+(−15.3−11.1i)T2 1 + (-0.809 + 2.48i)T + (-15.3 - 11.1i)T^{2}
23 1+3.85T+23T2 1 + 3.85T + 23T^{2}
29 1+(0.163+0.502i)T+(−23.4+17.0i)T2 1 + (0.163 + 0.502i)T + (-23.4 + 17.0i)T^{2}
31 1+(−8.28−6.01i)T+(9.57+29.4i)T2 1 + (-8.28 - 6.01i)T + (9.57 + 29.4i)T^{2}
37 1+(2.73+8.42i)T+(−29.9+21.7i)T2 1 + (2.73 + 8.42i)T + (-29.9 + 21.7i)T^{2}
41 1+(1.14−3.52i)T+(−33.1−24.0i)T2 1 + (1.14 - 3.52i)T + (-33.1 - 24.0i)T^{2}
43 1+9.47T+43T2 1 + 9.47T + 43T^{2}
47 1+(−0.0729+0.224i)T+(−38.0−27.6i)T2 1 + (-0.0729 + 0.224i)T + (-38.0 - 27.6i)T^{2}
53 1+(1.11+0.812i)T+(16.3+50.4i)T2 1 + (1.11 + 0.812i)T + (16.3 + 50.4i)T^{2}
59 1+(3.54+10.9i)T+(−47.7+34.6i)T2 1 + (3.54 + 10.9i)T + (-47.7 + 34.6i)T^{2}
61 1+(1.80−1.31i)T+(18.8−58.0i)T2 1 + (1.80 - 1.31i)T + (18.8 - 58.0i)T^{2}
67 1+9.32T+67T2 1 + 9.32T + 67T^{2}
71 1+(7.92−5.75i)T+(21.9−67.5i)T2 1 + (7.92 - 5.75i)T + (21.9 - 67.5i)T^{2}
73 1+(−2.51−7.74i)T+(−59.0+42.9i)T2 1 + (-2.51 - 7.74i)T + (-59.0 + 42.9i)T^{2}
79 1+(−9.66−7.02i)T+(24.4+75.1i)T2 1 + (-9.66 - 7.02i)T + (24.4 + 75.1i)T^{2}
83 1+(7.28−5.29i)T+(25.6−78.9i)T2 1 + (7.28 - 5.29i)T + (25.6 - 78.9i)T^{2}
89 1+13.1T+89T2 1 + 13.1T + 89T^{2}
97 1+(9.70+7.05i)T+(29.9+92.2i)T2 1 + (9.70 + 7.05i)T + (29.9 + 92.2i)T^{2}
show more
show less
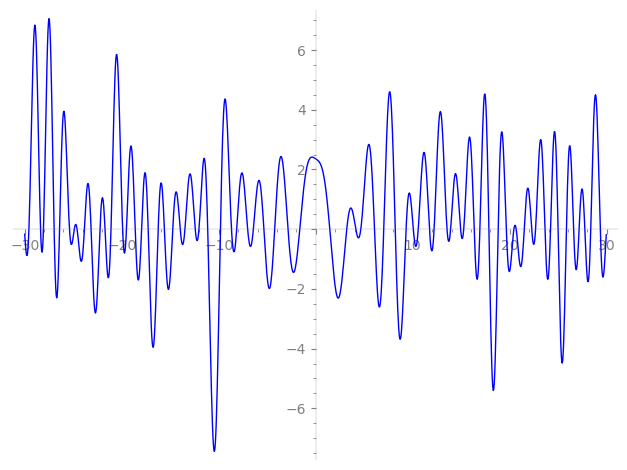
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−11.13321964509832851051515312003, −9.823748839916816534096535340990, −8.681749797210188084068191382790, −8.148426609054947401298120110770, −7.01703346470665150888252092779, −6.42774877131851644296201087652, −5.34632524689397124616372472283, −4.23162899185671318961552330918, −2.85716819555706553983343282281, −1.64704053097485234803427798234, 1.44840835629022151443446558423, 3.19717357854908788317891381201, 4.13154587604028447398252618662, 4.64654250736063069771338783213, 6.06023753451115941612270917194, 7.01241332524899192723445514658, 8.197858715340911673854251924115, 9.312947609700835660352450051289, 10.05854996027490666055482008300, 10.56669560627442646699821727631

Graph of the ZZ-function along the critical line