↑

Properties

Label 2-1682-29.28-c1-0-31
Degree 22
Conductor 16821682
Sign −0.989−0.141i-0.989 - 0.141i
Analytic cond. 13.430813.4308
Root an. cond. 3.664813.66481
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + i·2-s + 2.88i·3-s − 4-s + 2.81·5-s − 2.88·6-s + 3.85·7-s − i·8-s − 5.35·9-s + 2.81i·10-s + 2.90i·11-s − 2.88i·12-s + 0.364·13-s + 3.85i·14-s + 8.12i·15-s + 16-s − 0.925i·17-s + ⋯
L(s)  = 1  + 0.707i·2-s + 1.66i·3-s − 0.5·4-s + 1.25·5-s − 1.17·6-s + 1.45·7-s − 0.353i·8-s − 1.78·9-s + 0.889i·10-s + 0.876i·11-s − 0.834i·12-s + 0.101·13-s + 1.02i·14-s + 2.09i·15-s + 0.250·16-s − 0.224i·17-s + ⋯

Functional equation

Λ(s)=(1682s/2 ΓC(s) L(s)=((−0.989−0.141i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 1682 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (-0.989 - 0.141i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(1682s/2 ΓC(s+1/2) L(s)=((−0.989−0.141i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 1682 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (-0.989 - 0.141i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 16821682    =    2⋅2922 \cdot 29^{2}
Sign: −0.989−0.141i-0.989 - 0.141i
Analytic conductor: 13.430813.4308
Root analytic conductor: 3.664813.66481
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ1682(1681,⋅)\chi_{1682} (1681, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 1682, ( :1/2), −0.989−0.141i)(2,\ 1682,\ (\ :1/2),\ -0.989 - 0.141i)

Particular Values

L(1)L(1) ≈\approx 2.2729967512.272996751
L(12)L(\frac12) ≈\approx 2.2729967512.272996751
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1−iT 1 - iT
29 1 1
good3 1−2.88iT−3T2 1 - 2.88iT - 3T^{2}
5 1−2.81T+5T2 1 - 2.81T + 5T^{2}
7 1−3.85T+7T2 1 - 3.85T + 7T^{2}
11 1−2.90iT−11T2 1 - 2.90iT - 11T^{2}
13 1−0.364T+13T2 1 - 0.364T + 13T^{2}
17 1+0.925iT−17T2 1 + 0.925iT - 17T^{2}
19 1+5.15iT−19T2 1 + 5.15iT - 19T^{2}
23 1+2.53T+23T2 1 + 2.53T + 23T^{2}
31 1−8.03iT−31T2 1 - 8.03iT - 31T^{2}
37 1−3.62iT−37T2 1 - 3.62iT - 37T^{2}
41 1+4.99iT−41T2 1 + 4.99iT - 41T^{2}
43 1−8.55iT−43T2 1 - 8.55iT - 43T^{2}
47 1−7.50iT−47T2 1 - 7.50iT - 47T^{2}
53 1+9.16T+53T2 1 + 9.16T + 53T^{2}
59 1+4.66T+59T2 1 + 4.66T + 59T^{2}
61 1−6.85iT−61T2 1 - 6.85iT - 61T^{2}
67 1−1.40T+67T2 1 - 1.40T + 67T^{2}
71 1−4.31T+71T2 1 - 4.31T + 71T^{2}
73 1−12.5iT−73T2 1 - 12.5iT - 73T^{2}
79 1+12.0iT−79T2 1 + 12.0iT - 79T^{2}
83 1−14.5T+83T2 1 - 14.5T + 83T^{2}
89 1+11.4iT−89T2 1 + 11.4iT - 89T^{2}
97 1+12.4iT−97T2 1 + 12.4iT - 97T^{2}
show more
show less
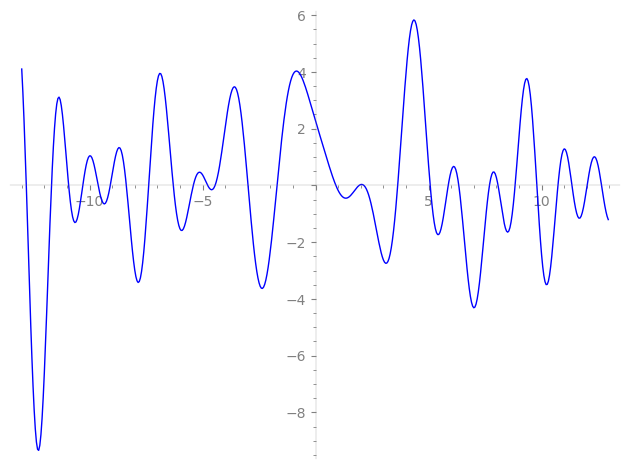
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−9.617397322650222683943807783940, −9.082776953316463275870123612334, −8.372636027775550897483432205449, −7.38441643697355189228920853813, −6.29533982985320044601714023126, −5.40906125250230330894555940311, −4.77303665223866491057415088614, −4.44582938694923730169300578471, −2.98803071366726838731385772621, −1.70508040777107485058005780019, 0.898344286791321625907857709007, 1.92812615418588373601155604097, 2.13709702147950374047655033403, 3.63360343215276494722032065958, 5.07127992496119563403588717908, 5.86924895764484201513929794542, 6.33649293421323134509840427149, 7.69322815596617329915033735389, 8.073161155591694097760190676853, 8.827925399722118377653916982609

Graph of the ZZ-function along the critical line